Green arc : Red arc = 7 : 11
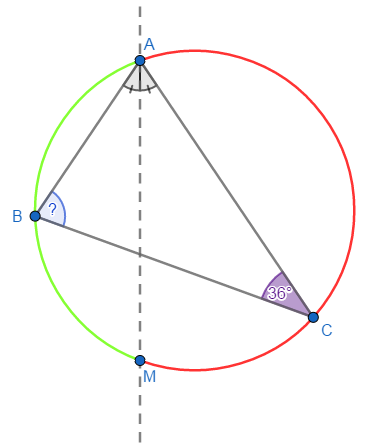
In △ A B C , internal angle bisector of ∠ B A C divides △ A B C 's circumcircle into two arcs whose ratio of lengths is 7 : 1 1 . Given that ∠ A C B = 3 6 ° , find the measure of ∠ A B C in degrees.
The answer is 76.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
 Let
O
be the circumcenter of
△
A
B
C
, and
M
be the intersection point of circumcircle and bisector of
∠
B
A
C
. Ratio of lengths of minor and major arc of
A
M
is
7
:
1
1
, so if
R
is the circumradius, we have:
Let
O
be the circumcenter of
△
A
B
C
, and
M
be the intersection point of circumcircle and bisector of
∠
B
A
C
. Ratio of lengths of minor and major arc of
A
M
is
7
:
1
1
, so if
R
is the circumradius, we have:
3 6 0 ° 3 6 0 ° − ∠ A O M 2 π R 3 6 0 ° ∠ A O M 2 π R = 3 6 0 ° − ∠ A O M ∠ A O M = 1 1 7 ⇒ ∠ A O M = 1 4 0 °
∠ A C M is inscribed, so: ∠ A C M = 2 1 ∠ A O M = 7 0 ° . Also, quadrilateral A B M C is cyclic, so: ∠ B C M = ∠ B A M = 2 1 ∠ B A C .
Now we have that ∠ A C M = ∠ B C A + ∠ B C M = 3 6 ° + 2 1 ∠ B A C = 7 0 ° ⇒ ∠ B A C = 6 8 ° .
Finally, ∠ A B C = 1 8 0 ° − ∠ B A C − ∠ A C B = 7 6 °
Since A BM ⌢ + A CM ⌢ = 3 6 0 ∘ use the equation 7 x + 1 1 x = 3 6 0 ∘ to get x = 2 0 ∘ and so A BM ⌢ = 7 x = 7 ⋅ 2 0 ∘ = 1 4 0 ∘
A B ⌢ = 2 × ∠ C = 7 2 ∘
BM ⌢ = 1 4 0 ∘ − 7 2 ∘ = 6 8 ∘
∠ B A M = 2 1 ⋅ 6 8 ∘ = 3 4 ∘ , so ∠ B A C = 6 8 ∘
∠ B = 1 8 0 ∘ = 6 8 ∘ − 3 6 ∘ = 7 6 ∘