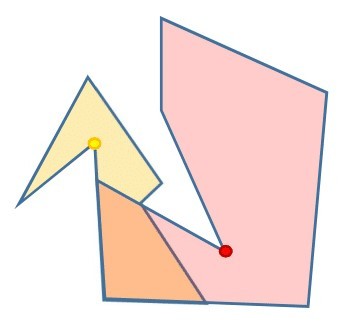
Guarding the Museum
An artist has a bird-shaped gallery. To ensure the museum is fully guarded, he places 4 guards at the corners with red dots so that every inner wall is visible by at least one guard.
Can he fully guard the museum with fewer guards?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
9 solutions

The author failed to state that the guards were interior rather than exterior. If the intent is to guard the art from exterior attack then four are needed.
Excellent and concise solution.
It is not stated that the bottom right corner is in line with the line towards it. It is possible that there is a small part which can be left ungaurded. I think the other solution is better.
Damn, I thought the Guards were standing at outer wall. Never thought of inner wall. :(
I also thought they were outside! :(
One guard can do it -- with security cameras. And s(he) doesn't even have to be onsite. :-) :-)
Log in to reply
Wrong. Zero guards can do it that way, just employ a motion detection armed drone and code it to incapacitate robbers or vandals.
What if they had bad eyesight
Top left is not in a corner.
Log in to reply
Doesn't say anywhere in the question that they have to be in corners. The example given has them all in corners but the guards can be anywhere.
I just realised how dumb I was
Wouldn't it be better to place the guards at the top of the head and tail of the swam shape building. So the guard at the top of the stand does not need to see at a 180 degrees for security.
i thought they were outside
i'd put it below and one on the top for impact
Aren't they supposed to be placed at corners?
Impossible with only two guards unless they can see through a wall!!
Log in to reply
The guards are on the inside of the museum, not on the outside.
But its an art gallery.. its gonna be crawling with people the guard on the far right corner will definitely miss on guarding the upper part
Log in to reply
Whether or not there are people in the gallery isn't relevant to the question. Unless otherwise stated, you always assume that there are no obstacles blocking the guards' field of view except for the walls themselves.
Guards are not at a corner!
The guards are not even in the same spots in each picture
This solution is nice because it uses only the corners, but the other is more realistic as guards do not have 320 degree vision!
If you're aiming for max coverage per guard assuming 360 degree vision, i.e. maximum overlap between what the two guards can see, the yellow guard is optimally placed like this, but the red guard should be moved to the bottom wall at the extension of the wall that has a ~120 degree with respect to the positive x-axis.
Cant look back.
I have a pair of eyes at the back of my head too!
Nice solution! If guards had 2 pair of eyes..
Although some people claim this is wrong, this is simply presented, like for that
This was the one I had in mind. Thanks, Marta Reece.
"to ensure the museum is fully guarded". Having only 2 guards is not going to be fully guarded because the 2 guards should have breaks and lunches according to voted law of place.
Log in to reply
How do you know the museum is not open only from 10 am to 11 am? :-p
If you want realistic guards, with a limited field of view which they can continuously monitor, placing both in a corner is best, and can be done with two guards, labeled as filled circles. (The yellow guard can see the yellow, orange and green parts, the blue guard can see the green, dark blue and blue parts in the following image.)
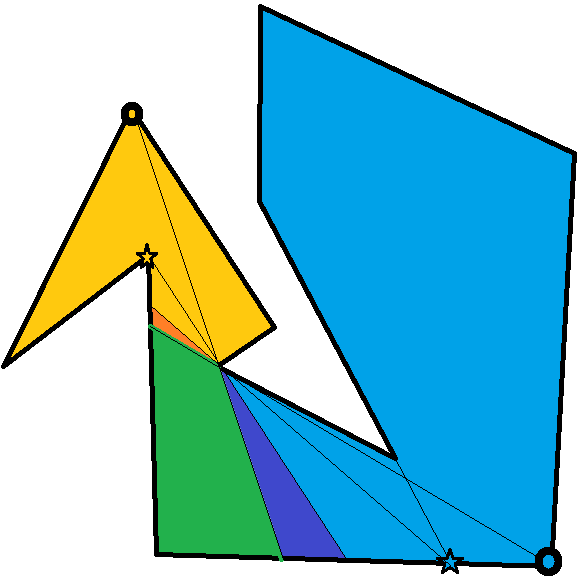
Alternatively, if your guards have a ridiculously large field of view, they could be placed at different locations, labeled as filled stars, so that they cover the largest area twice. (The yellow guard can see the yellow, orange, green and dark blue parts, the blue guard can see the orange, green, dark blue and blue parts in the image.) An additional advantage of these starred locations would be that the guards are nearest to each other (while maintaining full coverage), so in case of a break-in they can assist each other sooner.
PS: Assuming 360 degree vision, even other non-wall locations are possible, for example in the case of the yellow guard on the line between the yellow circle and star.
You are all wrong. The guards are outside, not inside and they can't see through the walls. 4 is actually the correct answer!
Log in to reply
I thought the same, but sadly the answer does say "inner wall is visible". If they were outside, no inner wall would be visible.
The question asks for guards that together can see all of the inner wall, which cannot be done with guards on the outside.
But suppose the outside wall needed to be guarded. In that case the 4-guard option presented in the question is not even a solution... The guard from the "tip of the beak" should have been moved to the "top of the swan's head".
it seem like the blue guard has a ,much larger AOR than the yellow.
Log in to reply
Indeed, the shape of the beak poses a big restriction on the location of the yellow guard. He has to be in (a small region within) the head of the swan, and the neck therefore limits his view. As a consequence, after placing only the yellow guard, most of the gallery is still unguarded, and the blue guard has to be placed somewhere near the bottom right corner to be able to cover this large area.
I call B.S. You said at the corners.. Not anywhere along the wall.. Therefore the correct answer is 3
Log in to reply
No that's just for the example. It doesn't say anything in the question about the guards being required to stay in the corners, therefore the obvious assumption is that the guards can be anywhere.
Log in to reply
You should say the guards can be placed in a position other than a corner.
The circles are in corners, right? That solution is a demonstration that 2 guards in corners are enough.
The problem didn't require corners, but even that clearly is possible with 2 guards.
Finding the 'Star Region ' is the technique to use, complex analysis. Really I should just have played about with moving dots around to ascertain maximum view gained.
Log in to reply
Problem solving: look at all the sides.
Just curious - How do you use complex analysis to solve that?
Log in to reply
tbh I cannot remember. It's been 38 yrs since I did anything on ' Star Regions' in 'Complex Analysis'.
I agree.You said corners
Your sketch is incorrect. if you see the image of the question, the guard on the top would not be able to sea everything that you claimed they would, therefore you are incorrect.
We need to consider the guards' plain sights without rotating head much. If we place one guard near the top corner of the peaky head of the bird (slightly below down the right wall to cover some part of the verticall wall of the neck) one more at the bottom right corner (intersection of tail outer and bottom belly) all the inner walls will be covered. Not only that, considering the picture is drawn to scale, the bottom guard will require less than 90 deg vision; so almost negligible turning of head. The top guard will require about 180 deg vision but the walls are much closer.
Most of the solutions provide a picture representing the guards and their view, which is how I solved it, but I was wondering whether or not there is a more rigorous solution to this puzzle.
i too was wondering
I look forward to seeing a rigorous and more general solution (for all shapes) from the staff
Can be done with 1 guard if you make your walls out of glass.... Hey, the question was "Can he fully guard the museum with fewer guards? " and my answer is yes, with one guard.
I'm not being facetious, I genuinely think people often constrain themselves by assuming limits that the question does not put on them. Anyway, glass walls are cool. :)
WE can place two guards here. One at the top left corner of the gallery and other at the base of gallery by shifting the second guard from the left to the other side but, not fully at the corner, but at a distance from the corner. Thanks.
Let the points be labelled from A to K, clockwise from the guard on the bird's beak. Let A' be where AK extended meets BC.
A good strategy is to try moving a guard to cover a proper superset of the area currently covered by that guard. The guard on A, currently covering exactly the triangle ABA', can be moved to anywhere in this triangle, thereby still covering the entire triangle and, in most cases, some more. If this guard is moved to K then he will cover the largest possible superset of ABA', including the entire area covered by the guard on C, so this guard can now be removed.
And then, you can move the guard on H to the intersection of FE extended and IJ, and remove the guard on J. The whole gallery is now covered by two guards.
1 can head of bird and Otherone is bottom corner of the bird