Harvard MIT MT

By the angle bisector theorem, $\frac{AX}{AC} = \frac{BX}{BC}$ , or $\frac{AX}{4} = \frac{5 - AX}{6}$ , which solves to $AX = 2$ , which means $BX = 5 - 2 = 3$ .

$\triangle AXN \sim \triangle ABC$ by AA similarity (since $\angle A$ is shared and $\angle C \cong \angle ANX$ by corresponding angles), so $\frac{XN}{AX} = \frac{BC}{AB}$ , or $\frac{XN}{2} = \frac{6}{5}$ , which solves to $XN = \frac{12}{5}$ .

Likewise, $\triangle BXM \sim \triangle ABC$ by AA similarity (since $\angle B$ is shared and $\angle A \cong \angle BXM$ by corresponding angles), so $\frac{XM}{BX} = \frac{AC}{AB}$ , or $\frac{XM}{3} = \frac{4}{5}$ , which solves to $XM = \frac{12}{5}$ .

Since $\angle C \cong \angle ANX$ by corresponding angles, and $\angle ANX \cong \angle NXM$ by alternate interior angles, $\angle C \cong \angle NXM$ .

By the law of cosines on $\triangle ABC$ , $\cos C = \frac{4^2 + 6^2 - 5^2}{2 \cdot 4 \cdot 6} = \frac{9}{16}$ . And by the law of cosines on $\triangle NXM$ , $\cos NXM = \cos C$ $=$ $\frac{(\frac{12}{5})^2 + (\frac{12}{5})^2 - x^2}{2 \cdot (\frac{12}{5}) \cdot (\frac{12}{5})}$ . So $\frac{9}{16} = \frac{(\frac{12}{5})^2 + (\frac{12}{5})^2 - x^2}{2 \cdot (\frac{12}{5}) \cdot (\frac{12}{5})}$ , which solves to $x = \frac{3 \sqrt{14}}{5} \approx \boxed{2.244}$ .

Harvard, MIT are you kidding me! >::

It's easy to see that AXN and XBM are both similar to ABC. By the angle bisector theorem, we have AX = 2, XB = 3. Thus XN = XM = 12/5, using our similar triangles..

By parallel lines XN and BC, with transversal NM, we see that angles NMC and XNM are congruent. Additionally, by the smaller similar triangles which form straight angle AXB with angle NXM, we see that NXM is congruent to angle ACB. This means that XNM and CNM are similar.

Observe that XNMB is a parallelogram so that AX = BM = 18/5, which can be found using similar triangles. Now this means CM = 12/5, so we have that triangles XNM and CNM are in fact congruent.

A little formula for the length of angle bisector CX (or even Stewart's theorem) gives that CX = 3√2. Suppose CX and NM meet at D. Since CX is an angle bisector and CNM is isosceles, CD is an altitude. Additionally, XNCM is a parallelogram so that D is the midpoint of CX.

Now we have the hypotenuse and one leg of right triangle CDN, so DN can be calculated using the Pythagorean theorem, and furthermore doubled to get MN since D is also a midpoint.

I am sparing the calculations because I am on mobile, but it comes out to MN = 6/5 * √(7/2) ~ 2.24.

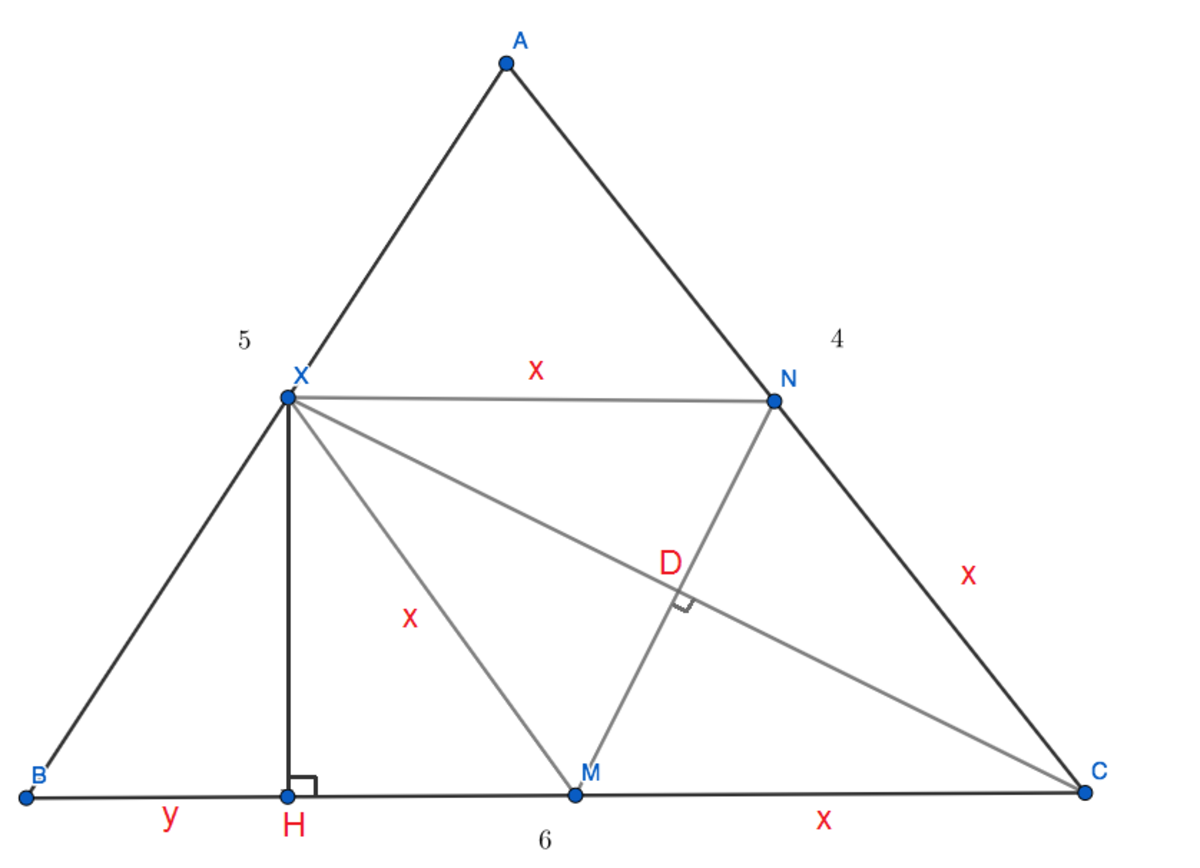
It is clear that $XNCM$ is a parallelogram. $XC$ is a diagonal of $XNCM$ which bisects this parallelogram's angle $\angle MCN$ . A property of a rhombus is that its diagonals bisect their corresponding angles, which means that $XNCM$ is not only a parallelogram, but a rhombus as well. So, $XN = NC = MC = XM = x$ .

Because $XM || AC$ and $XN || BC$ , $\triangle ABC$ is congruent to both $\triangle XBM$ and $\triangle AXN$

We can find the length of $x$ from the congruence between $\triangle ABC$ and $\triangle AXN$ :

$\dfrac{XN}{BC} = \dfrac{AN}{AC}$

$XN = x$

$AN = AC - NC = 4 - x$

$\dfrac{x}{6} = \dfrac{4 - x}{4} \Rightarrow \boxed{x = \dfrac{12}{5}}$

Using congruence between $\triangle ABC$ and $\triangle XBM$ :

$\dfrac{XB}{AB} = \dfrac{XM}{AC}$

$XB = \dfrac{XM}{AC} \times AB = \dfrac{x}{4} \times 5 = 3$

Now, we pick a point $H$ on side $BC$ such that $XH \perp BC$ , and let $y = BH$ . By Pythagoras, we have:

$XB^2 - BH^2 = XM^2 - HM^2 = XC^2 - HC^2 = XH^2$

Now, we find $y$ like this:

$HM = BC - MC - y = 6 - x- y$

$XB^2 - BH^2 = XM^2 - HM^2$

$9 - y^2 = x^2 - (6 - x - y)^2$

$9 - y^2 = (\dfrac{12}{5})^2 - (\dfrac{18}{5} - y)^2$

Solving this equation, we get that $\boxed{y = \dfrac{9}{4}}$ So, we can now determine the length of $XC$ :

$XC^2 = HC^2 + XB^2 - BH^2 = (6 - y)^2 + 9 - y^2 = 18$

Now, another property of a rhombus is that its diagonals are perpendicular to each other and bisect each other. Let $D$ be the point of intersection of $XNCM$ 's diagonals, $XC$ and $MN$ . Now we have:

$DM^2 + DC^2 = (\dfrac{MN}{2})^2 + (\dfrac{XC}{2})^2 = \dfrac{MN^2}{4} + \dfrac{XC^2}{4} = MC^2 = x^2$ .

Finally:

$MC = \sqrt{4x^2 - XC^2} = \sqrt{4 \times (\dfrac{12}{5})^2 - 18} = \dfrac{3}{5} \sqrt{14} \approx \boxed{2.244}$