Heptagon's fine, but!
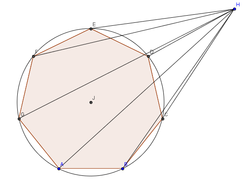 There's a regular heptagon
A
B
C
D
E
F
G
in the Cartesian plane with center of it's circumcircle at point
J
(
8
,
8
)
.
There's a regular heptagon
A
B
C
D
E
F
G
in the Cartesian plane with center of it's circumcircle at point
J
(
8
,
8
)
.
Every side of the heptagon is of length 4 sin 7 π .
Point H has coordinate ( 1 6 , 1 2 ) .
Find H A 2 + H B 2 + H C 2 + H D 2 + H E 2 + H F 2 + H G 2 .
Details and assumptions :
-
A regular heptagon has 7 vertices and all sides are of equal length.
-
The angle 7 π is in radians.
The answer is 588.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Thanks for this cool approach , did u learn this technique before , or this is original ..? @Aditya Raut
Log in to reply
Not original, I learnt that in physics, not too different in geometry though... Noticed that a problem of this type can be made, so posted one ;)
Did it the same way. This can easily be done by vectors too. :)
Lol now I feel stupid for complex bashing with roots of unity
I will use a vector approach here. First of all, it is clear that the radius of the circumcircle of the heptagon is 2 units. This is because the length of side of a regular n-gon is: s = 2 r sin n π Which can be proved by simple trigonometry. So, for the given case: 4 sin 7 π = 2 r sin 7 π ∴ r = 2 Now, consider: H A 2 = ∣ H A ∣ 2 = ( H J + J A ) . ( H J + J A ) = H J 2 + 2 H J . J A + J A 2 Let d be the distance between H and J : ∴ H A 2 = d 2 + 2 H J . J A + r 2 Thus, we can rewrite the original expression as follows: 7 ( d 2 + r 2 ) + 2 H J . ( J A + J B + J C + J D + J E + J F + J G ) = 7 ( d 2 + r 2 ) + 2 H J . ( 0 ) Which can be explained easily by symmetry. Thus, the required answer is: = 7 ( d 2 + r 2 ) = 7 ( 8 0 + 4 ) = 5 8 8
Note: This technique is essentially the same as the moment of inertia approach . This basically works as a proof for the parallel axis theorem, where J is the centre of mass of the system.
Thanks for the nice simple approach.
Well, though looks geometry, this is Moment of inertia! Attach unit masses at all the 7 vertices.
We know that moment of inertia I of a system of mass m , having moment of inertia I c o m about center of mass, about a point at distance l from center of mass is given by I =I c o m + m l 2
From the given side length, we conclude that circumradius, i.e. JA is 2 units, moment of inertia of each vertex being 1 × 2 2 = 4 .
I c m = 7 × 4 = 2 8 .
The center of mass will be J by simple observation, so l = J H
J H = ( 1 6 − 8 ) 2 + ( 1 2 − 8 ) 2 . . . Distance formula = 8 0
I c o m = 2 8 , m = 7 , l = 8 0
Hence, I H = 2 8 + 7 × 8 0 = 5 8 8 .