Here I go
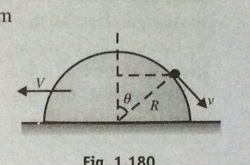 A bead of mass 'm' is kept at the top of a smooth hemispherical wedge of mass M and radius R . The bead Is gently pushed towards right . As a result the wedge slides due left . For the angular displacemt
of the bead , If the speed of the wedge can be represented as (where a,b,c are constants) find a+b+c
A bead of mass 'm' is kept at the top of a smooth hemispherical wedge of mass M and radius R . The bead Is gently pushed towards right . As a result the wedge slides due left . For the angular displacemt
of the bead , If the speed of the wedge can be represented as (where a,b,c are constants) find a+b+c
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
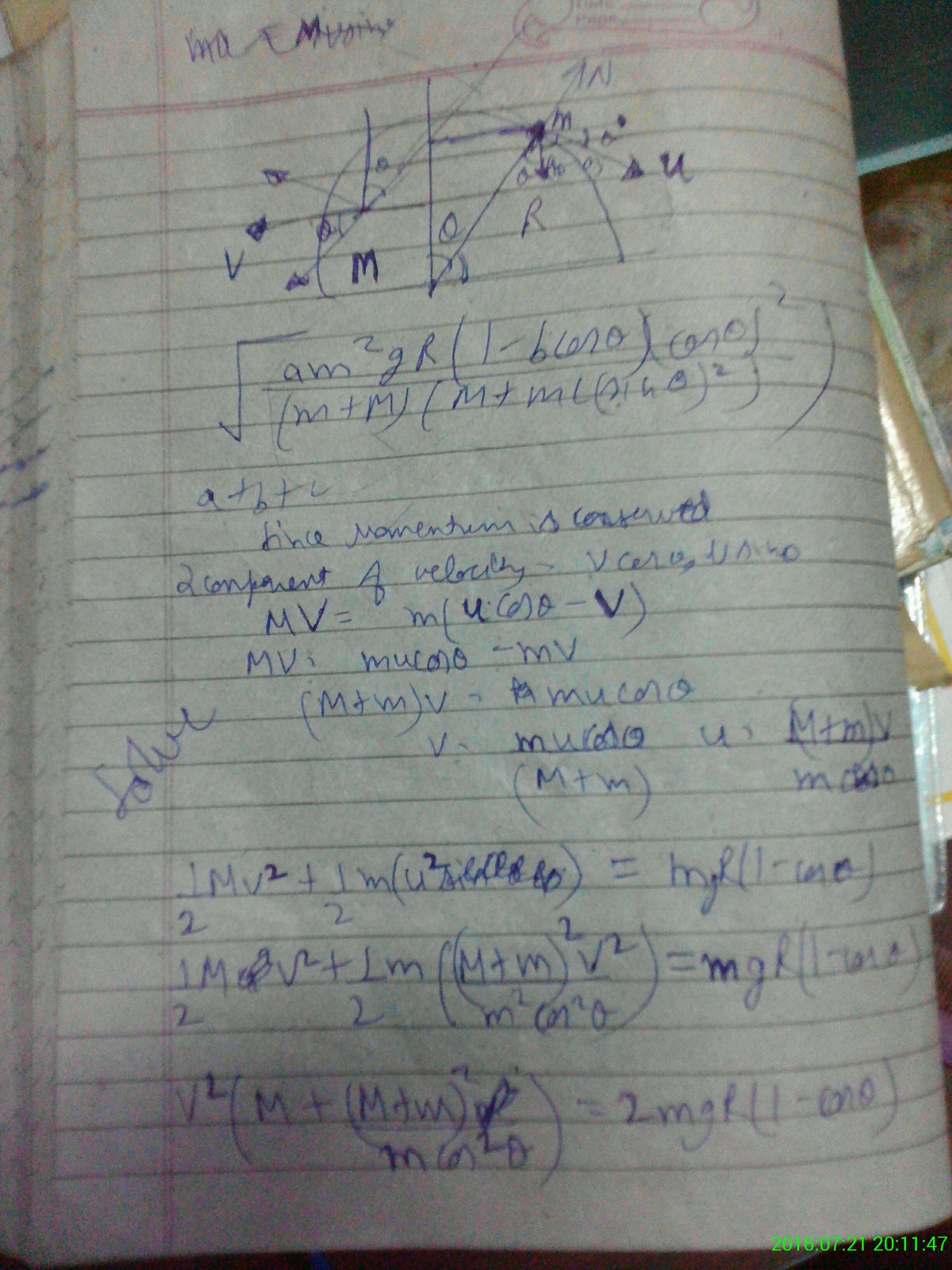 After you solve solve you get a=2 b=1 c=1 , i could have uploaded the whole solution but i leave that an excercise to you.
After you solve solve you get a=2 b=1 c=1 , i could have uploaded the whole solution but i leave that an excercise to you.
