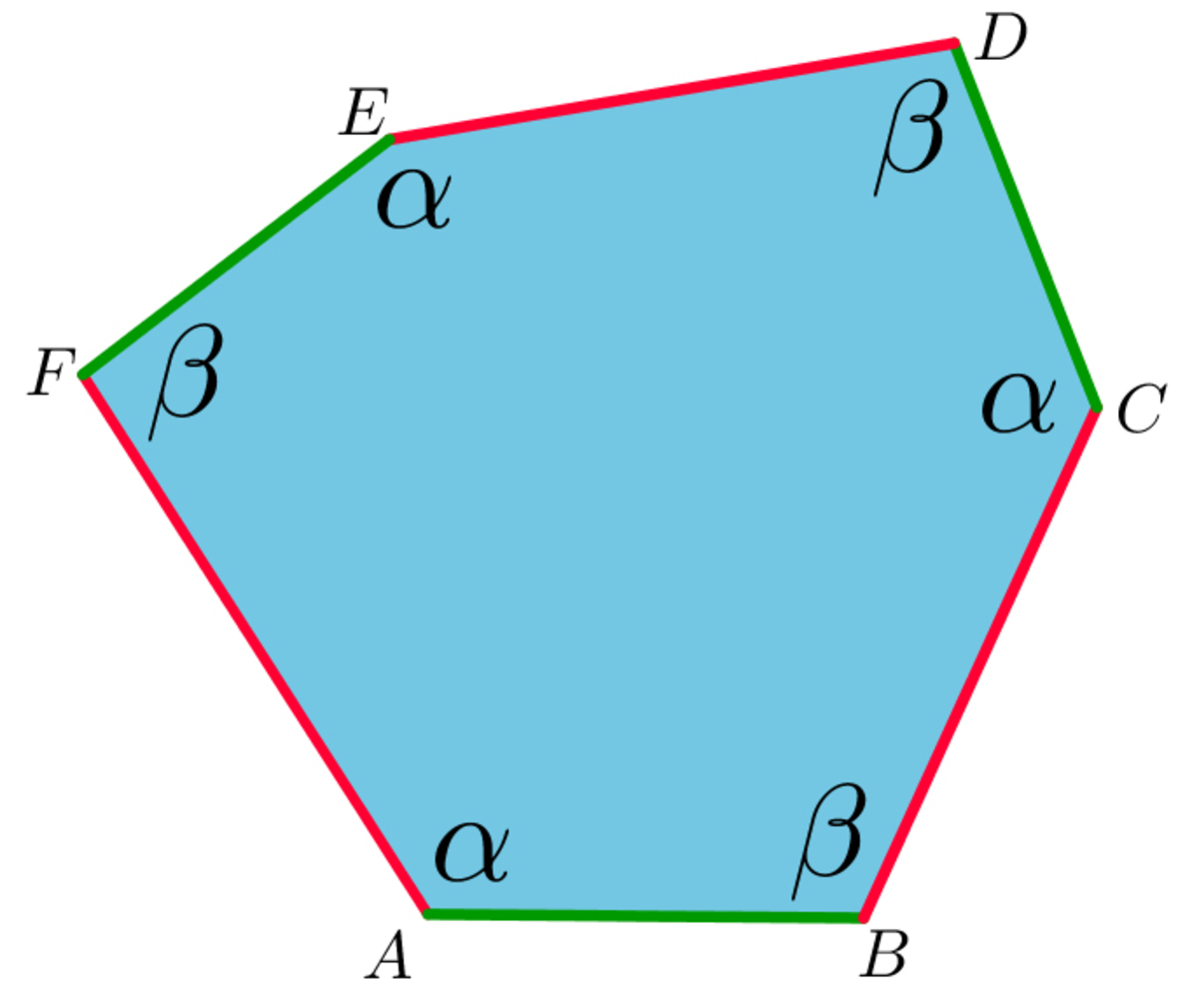
Hexagon time

The $AB, CD, EF$ lines define $\triangle PQR$ . Note that the sum of the angles in a hexagon is $720°$ , so $3\alpha+3\beta=720°$ , hence $\alpha+\beta=720°/3=240°$ . Then $\alpha'+\beta'=(180°-\alpha)+(180°-\beta)=120°$ . From that each angle of $\triangle PQR$ is $180°-120°=60°$ . Therefore $\triangle PQR$ is equilateral.

Since $FA=BC=DE$ , $\triangle PAF, \triangle QBC$ and $\triangle REF$ are congurent. Now it is clear that since a equilateral triangle has equal sides, $AB=CD=EF$ is definitely true!