High speed circular motion!
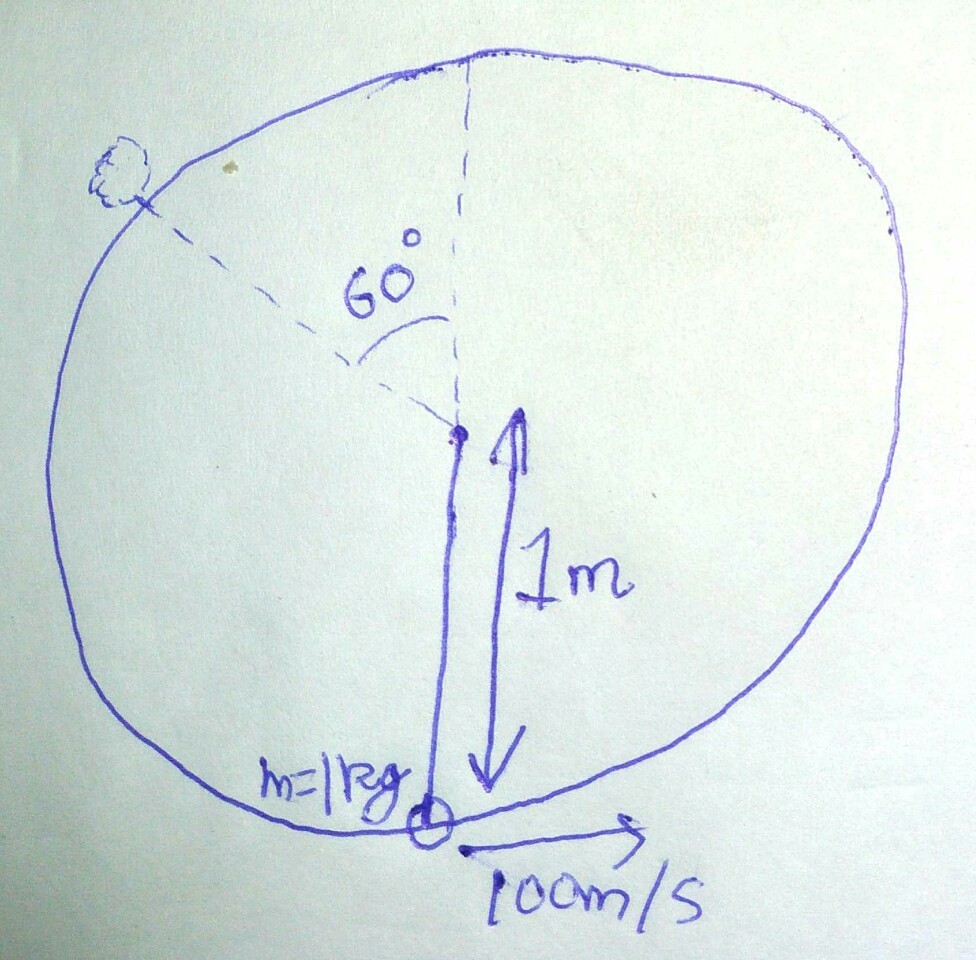
A ball of mass 1kg is hung with a massless rope of length 1m and is hit with a bat such that it attains a velocity of 100m/s in no time. Find the tension in the rope when the ball is at an angle of 60° with the topmost position attained by the ball(as shown in the diagram).
Details and Assumptions
• Take value of g as 10m/s² .
• Assume that as soon as the ball is hit, it attains a velocity of 100m/s (or in no time).
• Enter answer in Newton .
The answer is 9965.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Kinetic energy at bottommost point= 2 1 ×1×100²=5000J
At 60°
P.E.=1×10×(1+1sin30°)=15J
P.E.+K.E.=5000 (Law of conservation of energy)
15+1/2×1×v²=5000
v²=9970m²/s²
Centripetal force applied by rope - Weight applied by ball on rope at 60°= Tension in string
1 1 × 9 9 7 0 -10cos60°=T
9965N=T