Hole in 1 Cut

Logic Level 1

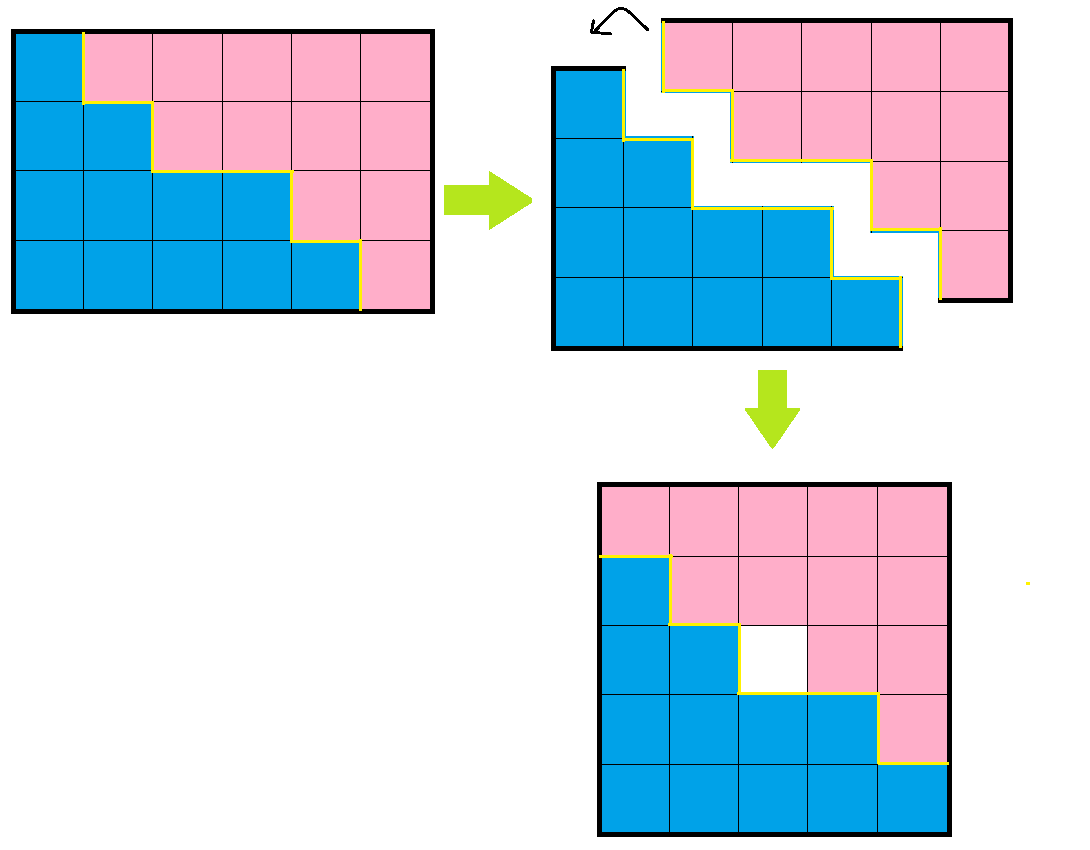
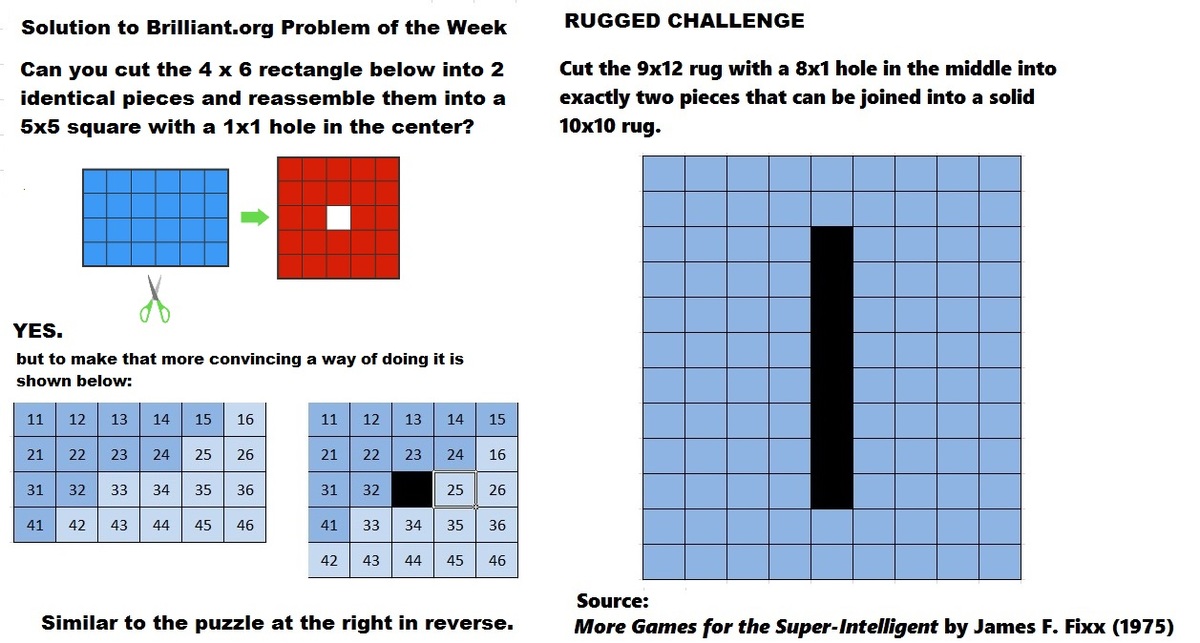
Using a single (possibly zig-zagging) line, can we cut the $4 \times 6$ rectangle into 2 identical pieces, and rearrange them into a $5 \times 5$ square with a $1 \times 1$ hole in the center?

Note : The cut may not be straight.

As an explicit example, this is how to get a $6 \times 5$ rectangle with a $2 \times 3$ hole in the center:

Yes No Not enough information

3 solutions

Worranat Pakornrat
Mar 15, 2017

Moderator note:

This particular dissection is used in a geometric vanish (where the area of a figure appears to change by just rearranging the parts).

Full video here.

More general problem: Show that we can always cut $\left(n-1\right)\times\left(n+1\right)$ rectangle in this way. (When $n > 1$ is odd.)

Jesse Nieminen - 4 years, 2 months ago

Wow! Cool! ;)

Worranat Pakornrat - 4 years, 2 months ago

@Calvin Lin What is a geometric vanish?

Agnishom Chattopadhyay - 4 years, 2 months ago

IE We made the center square appear to vanish. How was that done?

Hint: It is exactly this problem. Watch what the man does with the pieces.

Calvin Lin Staff - 4 years, 2 months ago

Robert DeLisle
Mar 24, 2017

What motivates you to come up with this cut?

Christopher Boo - 4 years, 2 months ago

Daniel Witting da Prato
Mar 24, 2017

Since it was not explicitly prohibited I imagined a solution with overlapping squares, but this is smarter.

@Daniel Witting da Prato - Could you share your solution with overlapping squares?

Aayush Agarwal - 4 years, 1 month ago

That wouldn't be possible because the first figure has an area of 6 * 4 = 24 squares and the second figure has an area of (5 * 5) - 1 = 24 squares as well.

Mira Jain - 3 years, 5 months ago

Hole in 1 Cut

3 solutions

Moderator note:

0 pending reports