Honeycomb Puzzle
Logic
Level
3
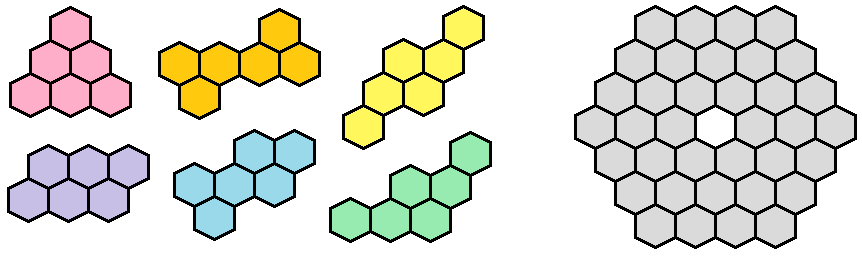
Allowing for rotations and reflections, is possible to use each of the colored pieces exactly once to completely cover every gray hexagon on the grid to the right?
No
Yes
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
There are 3 6 hexagons on the pierced hexagonal grid,. and 6 hexagons in each of the six pieces. Thus, to cover the whole grid, the six pieces must be laid in a non-overlapping manner.
Colour the pierced hexagonal grid with three colours as shown below, noting that the grid contains 1 2 hexagons of each colour.
The first five pieces always cover two hexagons of each colour, no matter how they are placed on the board, but the sixth piece does not do so. Suppose that the first five pieces have been placed (in a non-overlapping manner) on the board. Then there will be two hexagons of each colour as yet uncovered, and it will no t be possible to cover these six hexagons with the sixth piece (although it might be possible to do so with one of the first five).
Thus it is not possible to tile the pierced hexagonal with the six pieces shown.