How am I supposed to do that?

Let $z=x+iy$ where $i=\sqrt{-1}$ .Then $x^{2}+y^{2}=z(x-iy)$

$x+\frac{x}{x^{2}+y^{2}}=\frac{12}{5}$ ...(1)

$y-\frac{y}{x^{2}+y^{2}}=\frac{4}{5}$ .....(2)

$(1)+i\times(2)$ gives

$x+iy+\frac{x-iy}{x^{2}+y^{2}}=\frac{4}{5}(3+i)$

or, $z+\frac{1}{z}=2t$ where $t=\frac{2}{5}(3+i)$

Solving , $z=t+\sqrt{t^{2}-1}$ or $z=t-\sqrt{t^{2}-1}$

$t^{2}-1=\frac{1}{25}(5+24i)=(\frac{4+3i}{5})^{2}$

So , $z=\frac{6+2i}{5}+\frac{4+3i}{5}$ or $z=\frac{6+2i}{5}-\frac{4+3i}{5}$

Comparing, $x=2,y=1$ or $x=\frac{2}{5},y=-\frac{1}{5}$

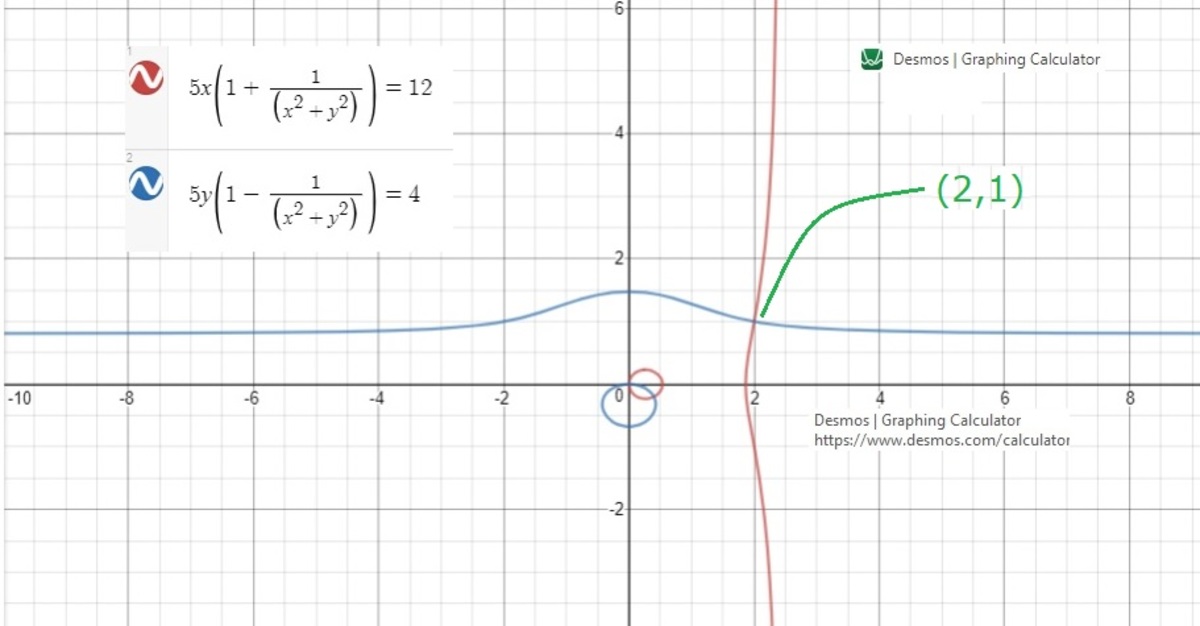Let $x^2+y^2=t > 0$ . From the second equation, we can see that $t \neq 1$ . Also, the brackets in both equations must be non-zero, so we can write $t=\left(\frac{12t}{5(t+1)}\right)^2+\left(\frac{4t}{5(t-1)}\right)^2$ $\frac{25}{16}=\frac{9t}{t^2+2t+1}+\frac{t}{t^2-2t+1}=\frac{t(10t^2-16t+10)}{(t+1)^2(t-1)^2}$ $25(t^2-1)^2=160t(t^2-\frac{8}{5}t+1)$

$P(t)=25t^4-160t^3+206t^2-160t+25=0$

Nasty equation added to inventory! It's time to try guessing roots. The obvious $-1, 0, 1$ give no decent results. Therefore, let's try rational roots.

We know that if $\frac{a}{b}$ is a root (with $a,b$ coprime), then after multiplying $P(t)$ by $b^4$ and taking the whole equation modulo $a$ , we get $25b^4\equiv0$ . But $a,b$ are coprime, so it implies $a|25$ . Similarly, we could take the whole equation $b^4P(t)=0$ modulo $b$ , obtaining $b|25$ .

We're free to try roots $\frac{a}{b}=\frac{1}{25},\frac{1}{5},5,25$ (we've already tried $1$ ). And, we can see that both $5$ and $\frac{1}{5}$ are roots. Factorising them out gives $P(t)=(t-5)\left(t-\frac{1}{5}\right)(25t^2+25-30t)=0$ and we can find out that $25t^2+25-30t=0$ has no real roots (its determinant is negative), so $t$ must be either $5$ or $\frac{1}{5}$ .

The rest is trivial: plug both values of $t=x^2+y^2$ into both initial equations and find the values of $x,y$ .

1) 1 + 1/ (x^2 + y^2) = 12/ (5 x)

2) 1 - 1/ (x^2 + y^2) = 4/ (5 y)

2 = 4/ 5 (3/ x + 1/ y)

y = 2 x/ (5 x - 6)

Since x <>0,

125 x^4 - 600 x^3 + 1045 x^2 - 780 x + 180 = 0

Divides with G.C.D. of 5 to polynomial equation obtained:

25 x^4 - 120 x^3 + 209 x^2 - 156 x + 36 = 0 {Ignore "How I am suppose to do that?"}

x1 = 1.2 - j 0.6

x2 = 0.4

x3 = 2

x4 = 1.2 + j 0.6

Substitute real x to check with y for original equations:

x = 0.4; 2 sum = 3.2

y = -0.2; 1

Eq1 = 12; 12

Eq2 = 4; 4

Answer: 3.2