How can you relate these two points?
 In
,
. The internal angle bisectors of
meet their respective opposite sides at
. Let
be a point on segment
such that
. Let the circumcircle of
intersects
at
.
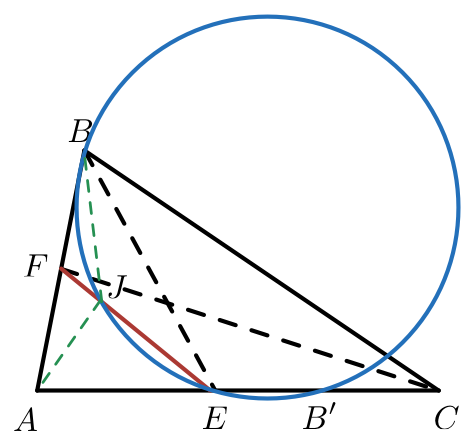
In
,
. The internal angle bisectors of
meet their respective opposite sides at
. Let
be a point on segment
such that
. Let the circumcircle of
intersects
at
.
Find the measure (in degrees) of .
The answer is 165.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
We extend F E to meet B C at K . It is a well known fact that A K is external angle bisector of ∠ A . Note that ∠ B J F = ∠ B B ′ A = 9 0 − 2 ∠ A = ∠ B A K , this means B , J , A , K are concyclic ⟹ ∠ A J B = 1 8 0 − ∠ A K B = 1 8 0 − ( ∠ B − 9 0 + 2 ∠ A ) = 1 6 5 .
To prove the well known property mentioned above, it suffices to show tht K C K B = A C A B , which can be done using Menelaus's theorem.