How many cylinders ?
A 10 x 10 x 10 cubic meter box - X is completely packed with 1000 small cubic boxes of dimension 1x1x1 meter. Now all the small cubic boxes are replaced with cylindrical shaped objects each of radius 0.5 and height 1. How many additional cylindrical boxes can be packed into the box X. The cylinders are arranged in
the box X as shown in the pic below
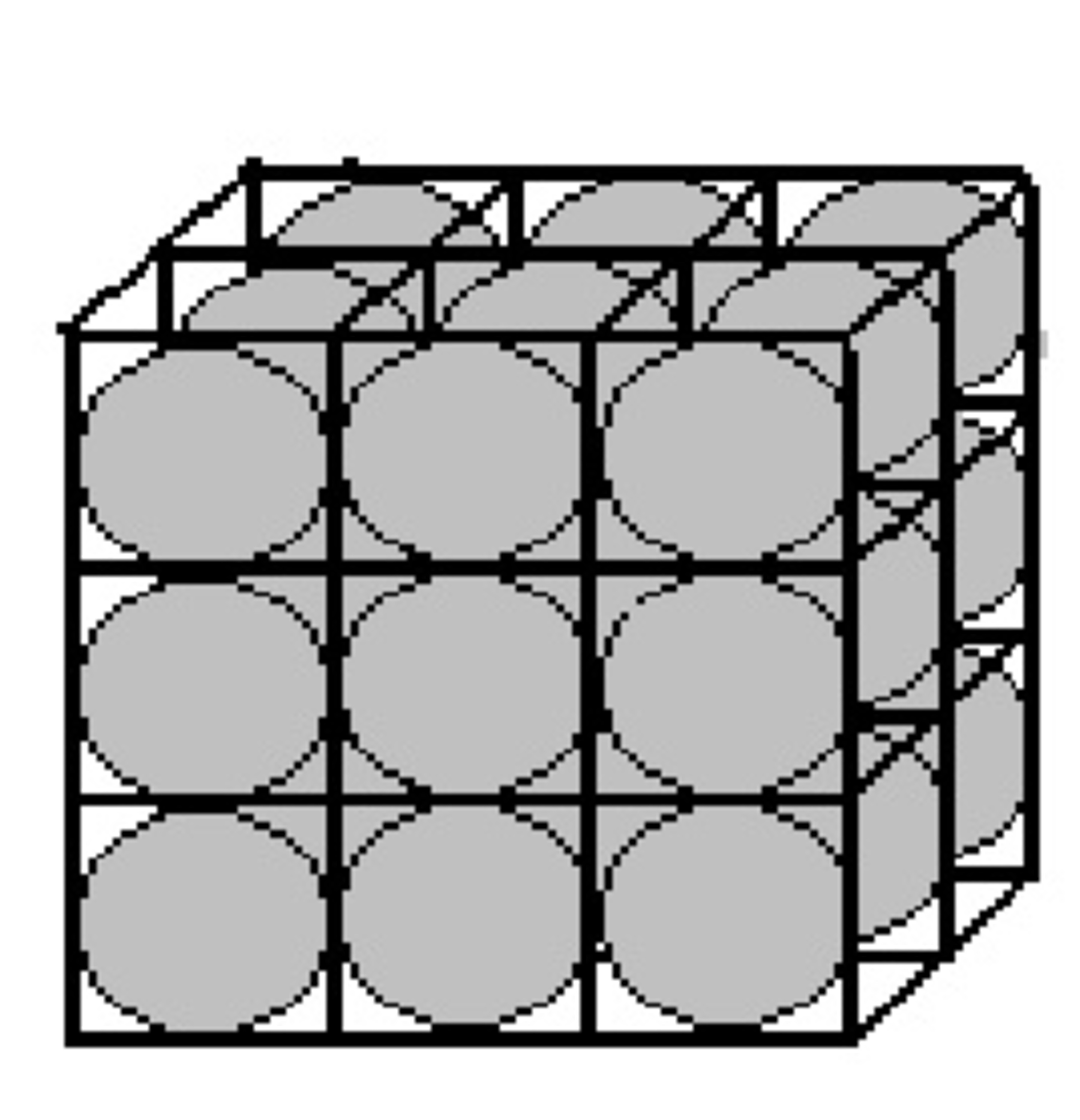The picture shows a cross section of the cylinders inside the cuboid and does not show the exact dimensions.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The diameter of each cylinder is 1 meter and 10 cylinders along the length of a 10x10x10 box can be packed. Similarly a maximum of 10 cylinders can be packed along the height and a maximum of 10 cylinders can be packed along the breadth. So there can be only 1000 cylinders packed. Originally there were 1000 small boxes of 1x1x1 dimension which were packed into the box. But however the total volume occupied by 1000 cylinders would be 1000 * pi * 0.5* 0.5* 1 = 785.71 and hence the free space would be 214.29. Hence the number of additional cylinders which can packed is 0.