How Many Triangles?
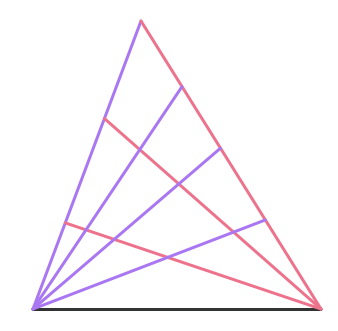
How many triangles are there which include the black line as a side?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
I'm not sure why only 55% got this problem right. I thought it should be straight-forward.
As a separate question, should I ask for the total number of triangles? That would be trickier to count.
Log in to reply
Yup, sounds like a good follow up... I'm also not sure they this problem has a 55% solve rate... :-/
This problem is pretty hard
This problem was pretty hard to me
Log in to reply
What did you find hard about it? Did you understand the solution?
I counted 25 and don't understand why you don't count both base angles to form the triangles
Log in to reply
Did you count any that don't have a black base?
Wouldn't it be 13..the whole thing is a triangle with the black line being a base
surely there are more than 20?
I counted more than 20 as well
Log in to reply
really ? I only counted 13
yeah I counted that many too
Did you ensure that the black line forms a side?
Here is the problem where we remove that restriction, and there are indeed much more than 20 triangles.
I counted 12, including the entire triangle.
Log in to reply
yeah but did you count the small triangles ?
I counted 13
You see carefully.
Can you explain which 13 you found? Maybe draw an image with these 13 triangles individually highlighted.
I appliaed pemutaion and combination and beleive including the bigger triangle it 12 only
Every triangle to be counted must have the black side, one purple side and one pink side. Combinatorics says there are four purple sides and three pink ones. So there are 4 choices for the purple side and three for the pink side, and 4*3 = 12. To check, you can count the points of intersection between the pink and purple lines (the possible top vertices of the triangles). The bottom purple line intersects exactly once with each pink line, and those points of intersection define three triangles. The second purple line also intersects exactly once with each pink line, as do the third and fourth purple lines. 3 + 3 + 3 + 3 = 12.
There are many more than 12 triangles in the image, but only 12 of them have the black line as a side.
The way this problem is set up, the intersections of purple and pink line segments form the third vertex of each triangle. Four purple line segments and three pink ones implies:
4 × 3 = 1 2