How much Rope can be there?
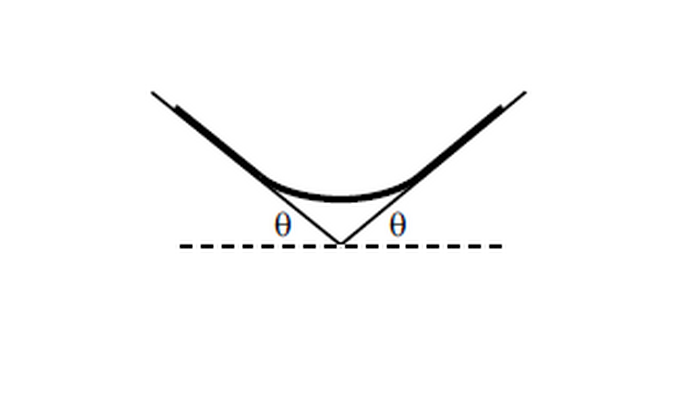 A rope rests on two platforms that are both inclined at an angle
θ
(which you are free to pick), as shown above. The rope has uniform mass density, and its coefficient of friction with platform is 1. The system has left-right symmetry. What is the largest possible fraction of the rope that does not touch the platforms?
A rope rests on two platforms that are both inclined at an angle
θ
(which you are free to pick), as shown above. The rope has uniform mass density, and its coefficient of friction with platform is 1. The system has left-right symmetry. What is the largest possible fraction of the rope that does not touch the platforms?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
Yups its a famous and innovative kinda problem..!! The first timers generally feel difficulty with it..!!
Log in to reply
There is very good solution to this problem in the Internet. (in PDF form)
This is a famous equilibrium problem. One of the approach is by considering the rope as 3 blocks having mass kx,ky and kx where k is mass per unit length and x is length lying on inclined plane and y be the length of hanging part. Now draw FBD and solve for equilibrium condition. U will get fraction as f = ( F ( θ ) / [ F ( θ ) + 1 ] ) w h e r e F ( θ ) = ( sin θ × cos θ − sin θ 2 ) Now applying calculus, we get fraction.