Hyperbola and Ellipse.
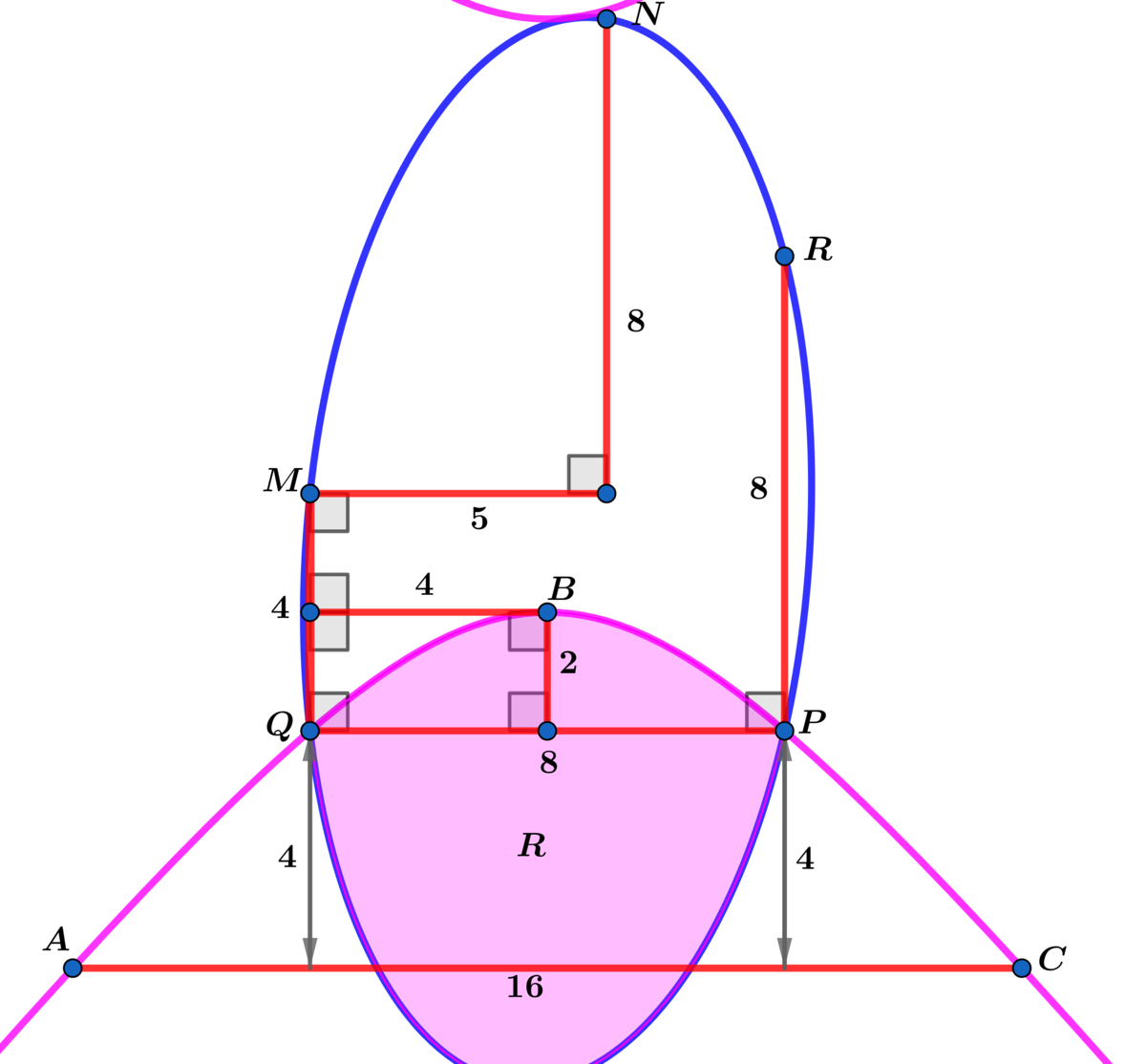
In the above diagram, the ellipse goes thru the points and the hyperbola goes thru the points .
Find the area of the region bounded by the hyperbola and the ellipse above to five decimal places.
The answer is 44.91295.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Using a x 2 + b x y + c y 2 + d x + e y = 0 and the points I chose above to generate the ellipse.
(1) ( 0 , − 4 ) : 4 c − e = 0 ⟹ c = 4 e
(2) ( 8 , 4 ) : 1 6 a + 8 b + 4 c + 2 d + e = 0
(3) ( 8 , − 4 ) : 1 6 a − 8 b + 4 c + 2 d − e = 0
Subtracting (3) from (2) we obtain: 8 b + e = 0 ⟹ b = − 8 e
(4) ( 5 , 8 ) : 2 5 a + 4 0 b + 6 4 c + 5 d + 8 c = 0
Replacing c = 4 e and b = − 8 e into (2) and (4) ⟹
1 6 a + 2 d = − e
2 5 a + 5 d = − 1 9 e
⟹ a = 1 0 1 1 e and d = − 1 0 9 3 e
⟹ 1 0 1 1 x 2 − 8 1 x y + 4 1 y 2 − 1 0 9 3 x + y = 0 ⟹ 4 4 x 2 − 5 x y + 1 0 y 2 − 3 7 2 x + 4 0 y = 0 ⟹ 1 0 y 2 + 5 ( x − 8 ) y + 4 4 x 2 − 3 7 2 x = 0
Solving for y we obtain:
y = 4 x − 8 ± 2 0 1 3 4 7 5 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 .
y e ( x ) = 4 x − 8 − 2 0 1 3 4 7 5 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 for the portion of the ellipse below the line y = 4 x − 8 .
Using x 2 + B x y + C y 2 + D x + E y + F = 0 and the points I chose above to generate the hyperbola.
(1) ( 0 , − 4 ) : 1 6 C − 4 E + F = 0 ⟹ F = 4 E − 1 6 C
(2) ( 8 , − 4 ) : 4 B − D = 8 ⟹ D = 4 B − 8 .
Using (1) and (2) above ⟹
(3): ( − 4 , − 8 ) : 4 B + 1 2 C − E = − 1 2
(4) ( 1 2 , − 8 ) : − 1 2 B + 1 2 C − E = − 1 2
(5) ( 4 , − 2 ) : 4 B − 6 C + E = 8
(3) + (5) ⟹ 4 B + 3 C = − 2
(3) - (4) ⟹ B = 0 ⟹ C = − 3 2 ⟹ D = − 8 ⟹ E = 4 ⟹ F = 3 8 0
⟹ 3 x 2 − 2 y 2 − 2 4 x + 1 2 y = − 8 0 ⟹ − 3 x 2 + 2 y 2 + 2 4 x − 1 2 y = 8 0 ⟹
2 ( y − 3 ) 2 − 3 ( x − 4 ) 2 = 5 0
Using the given portion of hyperbola below the line 4 x − 8 ⟹ y h ( x ) = 3 − 2 1 ( 5 0 + 3 ( x − 4 ) 3 ⟹ A h = ∫ 0 8 y h ( x ) d x .
For I ( x ) = 2 1 ∫ 5 0 + 3 ( x − 4 ) 2
Let 3 ( x − 4 ) = 5 0 tan ( θ ) ⟹ d x = 3 5 0 sec 2 ( θ ) d θ ⟹ I ( θ ) = 6 5 0 ∫ sec 3 ( θ ) d θ
For ∫ sec 3 ( θ ) d θ
Let u = sec ( θ ) ⟹ d u = sec ( θ ) tan ( θ ) and d v = sec 2 ( θ ) ⟹ v = tan ( θ ) ⟹ ∫ sec 3 ( θ ) d θ = sec ( θ ) tan ( θ ) − ∫ sec ( θ ) + ∫ sec ( θ ) d θ ⟹ ( ∫ sec 3 ( θ ) d θ = 2 1 ( sec ( θ ) tan ( θ ) + ln ∣ sec ( θ ) + tan ( θ ) ∣ ) ⟹
I ( x ) ∣ 0 8 = 6 2 5 ( 5 0 5 0 + 3 ( x − 4 ) 2 3 ( x − 4 ) + ln ∣ 5 0 5 0 + 3 ( x − 4 ) 2 + 3 ( x − 4 ) ∣ ) ∣ 0 8 = 2 8 + 6 2 5 ln ( 7 2 − 4 3 7 2 + 4 3 )
⟹ A h = − 4 − 6 2 5 ln ( 7 2 − 4 3 7 2 + 4 3 ) ≈ − 2 1 . 6 9 7 8 6
The area of the ellipse is A e = ∫ 0 8 y e ( x ) d x .
A e = 2 0 1 3 4 7 5 ∫ 0 8 − 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 + 4 x − 8 d x = 4 5 3 4 7 1 ∫ 0 8 − 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 + 4 x − 8 d x .
For I ( x ) = ∫ 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 d x
Let 3 4 7 x − 1 4 4 8 = 2 2 0 7 7 4 4 sin ( θ ) ⟹ d x = 3 4 7 2 2 0 7 7 4 4 cos ( θ )
⟹ I ( θ ) = 3 4 7 2 2 0 7 7 4 4 ∫ cos 2 ( θ ) d θ = 6 9 4 2 2 0 7 7 4 4 ∫ ( 1 + cos ( 2 θ ) ) d θ = 6 9 4 2 2 0 7 7 4 4 ( θ + sin ( θ ) cos ( θ ) )
⟹ I ( x ) = 6 9 4 2 2 0 7 7 4 4 ( arcsin ( 2 2 0 7 7 4 4 3 4 7 x − 1 4 4 8 ) + 2 2 0 7 7 4 4 3 4 7 x − 1 4 4 8 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 ) ⟹
A e = − ( 3 4 7 ) 2 3 5 2 7 5 9 6 8 ( arcsin ( 2 2 0 7 7 4 4 3 4 7 x − 1 4 4 8 ) + 2 2 0 7 7 4 4 3 4 7 x − 1 4 4 8 2 2 0 7 7 4 4 − ( 3 4 7 x − 1 4 4 8 ) 2 ) ∣ 0 8 =
− ( 3 4 7 ) 2 3 5 2 7 5 9 6 8 ( arcsin ( 2 2 0 7 7 4 4 1 3 2 8 ) + arcsin ( 2 2 0 7 7 4 4 1 4 4 8 ) + 2 2 0 7 7 4 4 1 3 2 8 4 4 4 1 6 0 + 2 2 0 7 7 4 4 1 4 4 8 1 1 1 0 4 0 ) − 8 =
− ( 3 4 7 ) 2 3 5 2 7 5 9 6 8 ( arcsin ( 2 8 1 1 8 3 ) + arcsin ( 5 6 1 1 1 8 1 ) + 3 4 4 9 6 5 1 3 1 7 3 5 ) − 8 ≈ − 6 6 . 9 1 2 9 5
⟹ A = ∫ 0 8 y h ( x ) − y e ( x ) d x = A h − A e = 4 4 . 9 1 2 9 5 .