I am waiting for you to Toss Toss
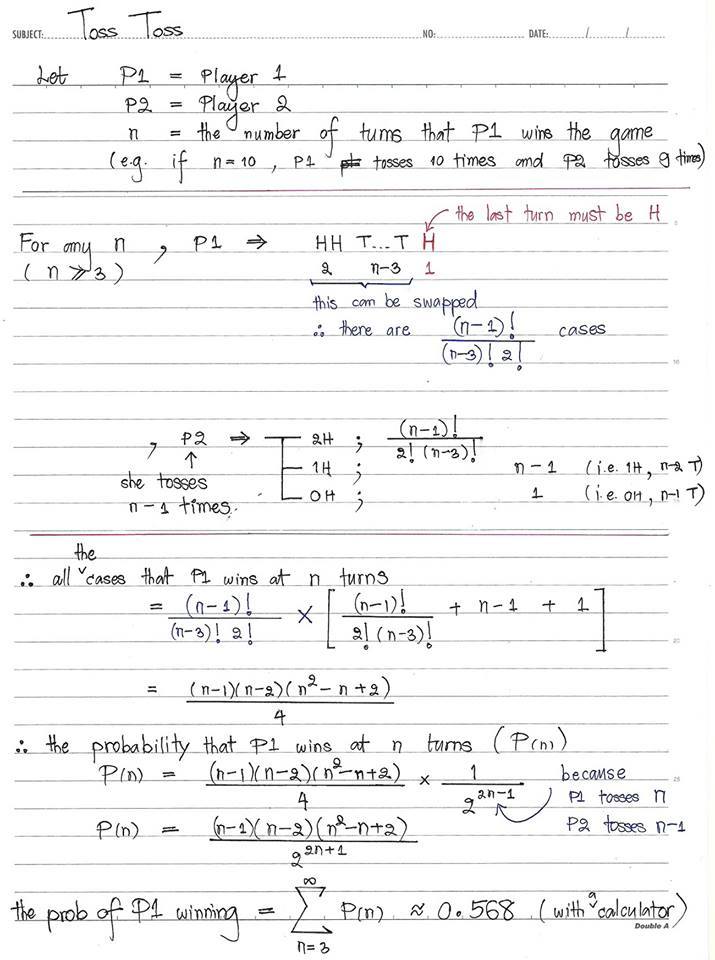
 You and Jessica take turns to toss a fair coin, with you going first. Whoever is the first to get 3 heads in total is the winner.
You and Jessica take turns to toss a fair coin, with you going first. Whoever is the first to get 3 heads in total is the winner.
What is the probability of you winning? Give your answer to three significant figures.
The answer is 0.568.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
I realise that there should be another method which is simpler than mine; look forward to seeing another one.
It only makes a difference who goes 1st is if Jess and I toss our 3rd head after the same number of turns. The probability of rolling one's 3rd head after k turns is ( 2 k − 1 ) 2 − k . Therefore the probability us rolling our 3rd head after the name number of turns is ∑ ( ( 2 k − 1 ) 2 − k ) 2 = 4 1 ∑ ( ( 2 k ) 2 − k ) 2
I'm not sure of the best way to evaluate this sum. What I did was consider the function 1 − x 1 = ∑ x k , the differentiate twice and multiply by x 2 , then differentiate twice and multiply by x 2 . You end up with the probability being 3 7 3 4 5 = 0 . 1 1 2 .
Now the probability of me winning is the probability of my going 1st being the deciding factor + 1/2 * the probability of my going first not being the deciding factor. 0 . 1 1 2 + 2 1 − 0 . 1 1 2 = 0 . 5 6 8