I can't figure this one out

The needed are is $[COPN] = a+b+c$ .

$a = \dfrac {1}{2} ([\text{Sector }ABC] -[\triangle ACO] ) = \dfrac {1}{2}\left( \dfrac {28^2\pi}{4}- \dfrac {28^2}{2} \right) \\ \quad = 111.8760801 cm^2$

To find $b$ and $c$ , let us assign coordinates as follows:

\(\begin{array} {} A(-14,14) & B(14,14) & C(14,-14) & D(-14,-14) \\ M(0,-14) & O(0,0) & P(x,y) & N(14(\sqrt{2}-1),-14(\sqrt{2}-1) \end{array} \)

Since $P(x,y)$ is the meeting point of the circles:

$\Rightarrow \begin{cases} x^2+(y+14)^2 & = 14^2 & ...(1) \\ (x+14)^2+(y-14)^2 & = 28^2 &...(2) \end{cases}$

Eq. 2 $-$ Eq. 1: $\space 28x+14^2-56y = 28^2-14^2 \quad \Rightarrow x = 2y +14$

Substituting in Eq. 1:

$4y^2+56y+14^2+y^2+28y+14^2=14^2$

$\Rightarrow 5y^2+84y+196= 0 \quad \Rightarrow y = -2.8 \quad \Rightarrow x = 8.4$

We note that the area of sector $APN = b + c+d$ . Let $\angle PAN = 2 \theta$ . Then, $b+ c+d = 28^2\theta$ . We also note that:

$\begin{aligned} \sin{\theta} & = \dfrac {\frac{1}{2} PN} {28} = \dfrac {\sqrt{[8.4-14(\sqrt{2}-1)]^2+[-2.8+14(\sqrt{2}-1)]^2}}{56} \\ & = 0.07088902 \quad \Rightarrow \theta = 0.070948527 rad \end{aligned}$

Therefore,

$b+ c+d = 28^2(0.070948527) = 55.6236454$

$\begin{aligned} b & = 55.6236454 - (c+d) = 55.6236454 - \dfrac{1}{2}\dot{} 14 \sqrt{2} \dot{} 28 \sin {2\theta} \\ & = 55.6236454 - 39.2 = 16.4236454 \end{aligned}$

To find $c$ , let $\angle OMP = 2 \phi$

$\begin{aligned} \sin{\phi} & = \dfrac {\frac{1}{2} OP} {14} = \dfrac {\sqrt{8.4^2+(-2.8)^2}}{28} \\ & = 0.316227766 \quad \Rightarrow \phi = 0.321750554 rad \end{aligned}$

Area of sector $MOP = 14^2\phi = 63.06310866$

$\begin{aligned} c & = 63.06310866 - [\triangle MOP] = 63.06310866 - 14^2\sin{\phi}\cos{\phi} \\ & = 63.06310866 - 58.8 = 4.263108662 \end{aligned}$

Therefore, the shaded area:

$= a+b+c = 111.8760801+16.4236454+4.263108662 = \boxed{132.56}cm^2$

1) Put "M" in the mid point on the line "BC" and "F" in the intersection point between arc "BD" and "BC".

2) Make the line "AM", "AF", "MF", "ME"

3) The [Area1] + [Area2] = Fan area "ABF"+ Fan area"MFB" - Kite area "ABMF"

  (∵ A ∪ B = A + B - (A ∩ B) )

4) Kite area "ABMF" = 2 × Triangle area "ABM"

  (∵ Triagle "ABM" = "AMF" , due to the same of 3 sides)

5) The [Area2] = Fan area "MEB" - Triangle area"MEB"

6) So, we could derive the [Area1] [Area1] = Fan area "ABF" + Fna area"MFB" - 2 × Triangle area "ABM" - Fan area "MEB" + Tiangle area "MEB"

= 1/2×(2r)^2×2×Θ1 + 1/2×r^2×2×Θ2 - 2×1/2×(2r)×r - 1/4×π×r^2 + 1/2×r^2
= 3×r^2×Θ1 + 1/4×π×r^2 - 3/2×r^2    (∵θ1 + θ2 = π/2)
= r^2×{3×Θ1 + 1/4×π - 3/2}

7) Finally, we could find the [Area1] with r=14cm, (θ1 = Tan-1(1/2) ≒ 0.46365 rad, with a calculater)

[Area1] = 14cm×14cm×{3×0.46365 + 1/4×π - 3/2} = 132.56cm^2

i have see many solutions without the approach of calculus ,amazing... ,i personally use coordinate system +calculus to solve

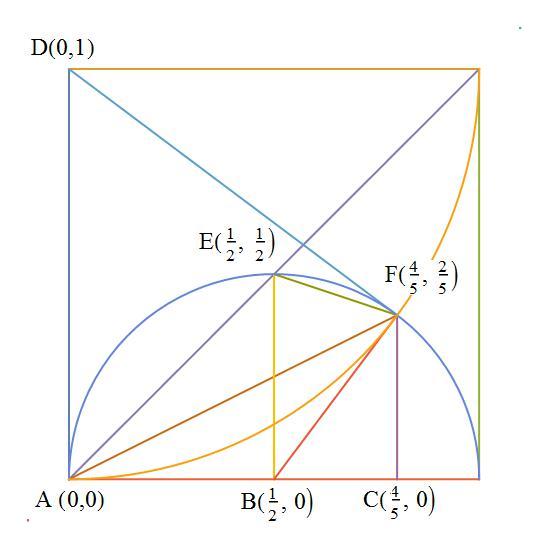
This depends on the fact that the arcs are orthogonal at point F. First, we rescale the square to an unit square. Then we have

Arc angle $D=ArcTan\left( \dfrac { 4 }{ 5 } \dfrac { 5 }{ 3 } \right)$

Arc angle $B=ArcTan\left( \dfrac { 3 }{ 10 } \dfrac { 5 }{ 2 } \right)$

From these, we can compute the areas of circle segments $AF$ and $EF$

$AF=\dfrac { 1 }{ 2 } D-Sin\left( \dfrac { 1 }{ 2 } D \right) Cos\left( \dfrac { 1 }{ 2 } D \right)$

$EF={ \left( \dfrac { 1 }{ 2 } \right) }^{ 2 }\left( \dfrac { 1 }{ 2 } B-Sin\left( \dfrac { 1 }{ 2 } B \right) Cos\left( \dfrac { 1 }{ 2 } B \right) \right)$

Next, we compute the area of triangle $AEF$

$AEF=\dfrac { 1 }{ 2 } \left( \dfrac { 1 }{ 2 } \dfrac { 1 }{ 2 } +\dfrac { 3 }{ 10 } \left( \dfrac { 1 }{ 2 } +\dfrac { 2 }{ 5 } \right) -\dfrac { 4 }{ 5 } \dfrac { 2 }{ 5 } \right)$

Combining all and rescaling back to square of side length $28$ , we have

${ 28 }^{ 2 }(AF+EF+AEF)=132.563...$

Let the lower left corner of the square be the origin $O(0,0)$ and let the sides of the square lie along the positive $x$ and $y$ -axes. Next, label the other corners of the square $P(0,28), Q(28,28), R(28,0),$ and let $M(14,0)$ be the center of the semicircle. Also, the semicircle and diagonal meet at $S(14,14).$

Now to find the point other than $O$ where the semicircle and quarter-circle meet can be determined by solving, simultaneously, the equations

$(x - 14)^{2} + y^{2} = 14^{2}$ and $x^{2} + (y - 28)^{2} = 28^{2}.$

Expanding these equations gives us that

$x^{2} - 28x + y^{2} = 0$ and $x^{2} + y^{2} - 56y = 0$

$\Longrightarrow 28x = 56y \Longrightarrow x = 2y \Longrightarrow 5y^{2} = 56y \Longrightarrow y = \dfrac{56}{5}, x = \dfrac{112}{5}.$

So label this point of intersection $T(\frac{112}{5}, \frac{56}{5}).$

So now we need to find the area of the shaded region $OST.$ To do this geometrically, we need to add the areas of the following three subregions:

• (i) the triangle $\Delta OST$ ;

• (ii) the sector $POT$ minus the triangle $\Delta POT$ ;

• (iii) the sector $MST$ minus the triangle $\Delta MST.$

For (i), we can use the standard distance-between-points formula to find that

$|OS| = 14\sqrt{2}, |ST| = \dfrac{14}{5}\sqrt{10}, |OT| = \dfrac{56}{5}\sqrt{5}.$

Using Heron's formula we find that the area of $\Delta OST$ is $78.400...$ .

For (ii), we note first that $\angle OPT = \tan^{-1}(\frac{4}{3}).$ Next, we note that the area of $\Delta POT$ is $\frac{1}{2}*28*\frac{112}{5} = \frac{1568}{5}.$ Thus the area of subregion (ii) is

$\frac{1}{2}*28^{2}*\tan^{-1}(\frac{4}{3}) - \frac{1568}{5} = 392\tan^{-1}(\frac{4}{3}) - \frac{1568}{5} = 49.8997.....$

For (iii), we note first that $\angle SMT = \tan^{-1}(\frac{3}{4}).$ Next, we note that the area of $\Delta MST$ is $\frac{1}{2}*14*(\frac{112}{5} - 14) = \frac{294}{5}.$ Thus the area of subregion (iii) is

$\frac{1}{2}*14^{2}*\tan^{-1}(\frac{3}{4}) - \frac{294}{5} = 98\tan^{-1}(\frac{3}{4}) - \frac{294}{5} = 4.263....$

Adding the areas of these three subregions gives us a total area of the shaded region of $78.400 + 49.900 + 4.263 = \boxed{132.563}$ to 3 decimal places.

Use coordinate geometry and some calculus.

Big circle with radius 28: x^2+(y+28)^2=28^2 = 28-sqrt(784-x^2)

Small circle with radius 14: (x-14)^2+(y^2=14^2= sqrt(28x-x^2)

If you are not sure how to formulate circle equation, refer to circle equation.

Find intersection points between two circles: Set both equation equal to each other and we get ( 0,0) and ( 112/5, 56/5)

Now we calculate areas

area a= Intergate sqrt(28x-x^2) from 28 to 112/5 = 43.835

area b= Integrate 28-sqrt(784-x^2) from 112/5 to 0 = 75.540

area c= 14^2- Integrate sqrt(28x-x^2) from 0 to 14= 196- 153.94= 42.06

area d= right triangle of (14*14)/2 - 42.06 = 55.94

area e= what the question seek= small semi circle- ( a+b+d)= 307.87- ( 43.835+ 75.540+55.94)= 132.561