I don't know how to count
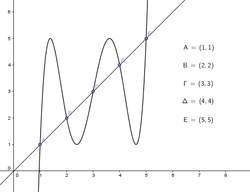 Consider a quintic function
f
(
x
)
such that
f
(
k
)
=
k
for
k
=
1
,
2
,
3
,
4
,
5
. Given that
f
(
x
)
=
g
(
x
)
+
q
(
x
)
such that
g
(
x
)
has all real roots and its leading coefficient is an integer and
q
(
x
)
is a linear function, which of these answer choices is
(
f
(
6
)
−
6
)
always divisible by?
Consider a quintic function
f
(
x
)
such that
f
(
k
)
=
k
for
k
=
1
,
2
,
3
,
4
,
5
. Given that
f
(
x
)
=
g
(
x
)
+
q
(
x
)
such that
g
(
x
)
has all real roots and its leading coefficient is an integer and
q
(
x
)
is a linear function, which of these answer choices is
(
f
(
6
)
−
6
)
always divisible by?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
I'm not quite sure how you got f ( x ) − x = g ( x ) . I understand the rest, but could you please explain that to me?
Log in to reply
f ( 1 ) = 1 , f ( 2 ) = 2 , f ( 3 ) = 3 , f ( 4 ) = 4 , f ( 5 ) = 5
⟹ f ( 1 ) − 1 = 0 , f ( 2 ) − 2 = 0 , f ( 3 ) − 3 = 0 , f ( 4 ) − 4 = 0 , f ( 5 ) − 5 = 0
⟹ f ( x ) − x = 0 , x = 1 , 2 , 3 , 4 , 5
Let g ( x ) = f ( x ) − x . ⟹ g ( x ) = 0 , x = 1 , 2 , 3 , 4 , 5
Since each function is given to us: let f ( x ) − x = g ( x )
By observing the values given, the zeroes of g ( x ) are 1 , 2 , 3 , 4 , 5 .
Hence, we have a system of polynomials for g ( x ) .
g ( x ) = k ( x − 1 ) ( x − 2 ) ( x − 3 ) ( x − 4 ) ( x − 5 )
where k is an arbitrary non zero integer constant
f ( x ) − x = k ( x − 1 ) ( x − 2 ) ( x − 3 ) ( x − 4 ) ( x − 5 )
By shifting the x to R.H.S, it can be seen that q ( x ) = x
f ( 6 ) − 6 = k ( 5 ) ( 4 ) ( 3 ) ( 2 ) ( 1 ) = k ( 5 ! )
Hence f ( 6 ) − 6 is a multiple of 5 ! .