A geometry problem by Vishwash Kumar ΓΞΩ
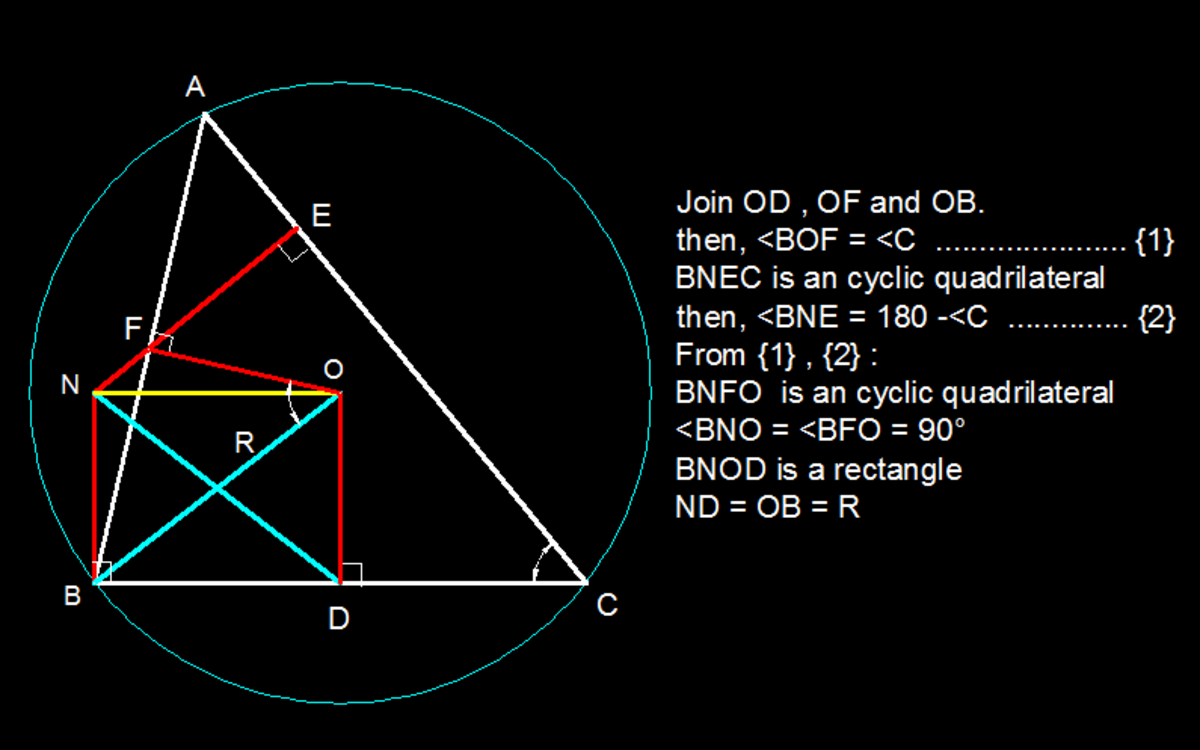
Let ABC be an acute triangle, let D , F be the midpoints of BC , AB respectively. Let the perpendicular from F to AC and the perpendicular at B to BC meet at a point N. Let R be the circumradius of triangle ABC.
Find the ratio ND : R.
The answer is 1.00.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Let the circumcentre O of the triangle be the origin for a system of vectors. Then O A = a O B = b O C = c where ∣ a ∣ = ∣ b ∣ = ∣ c ∣ = R , the circumradius. Consider the point N with position vector O N = 2 1 ( b − c ) = 2 1 C B = D B . Then, since O D = 2 1 ( b + c ) , we see that O N ⋅ O D = 0 .
Now N B = 2 1 ( b + c ) = O D , so that N lies on the line through B perpendicular to B C . Moreover N F = 2 1 ( a + b ) − 2 1 ( b − c ) = 2 1 ( a + c ) , and hence N lies on the line through F perpendicular to A C . Thus N is the point described in the question.
It is clear from the above that O N B D is a rectangle, and hence its diagonals are equal. Thus N D = O B = R , making the desired ratio equal to 1 .