I love being inspired! 8

We have $m+n=500-260=240$ By the cosine rule on dividing the quadrilateral into two triangles whose two sides are $(130,n)$ and $(130,m)$ , and a common side, we have $130^2+m^2-260m\cos\theta=130^2+n^2-260n\cos\theta$ $m+n=260\cos\theta=240$ So we get $\cos\theta=\frac{12}{13}$ So $\Large{\left\lfloor\frac{12*1000}{13}\right\rfloor=923}$

I hope my image comes in ok. I took a visual approach.

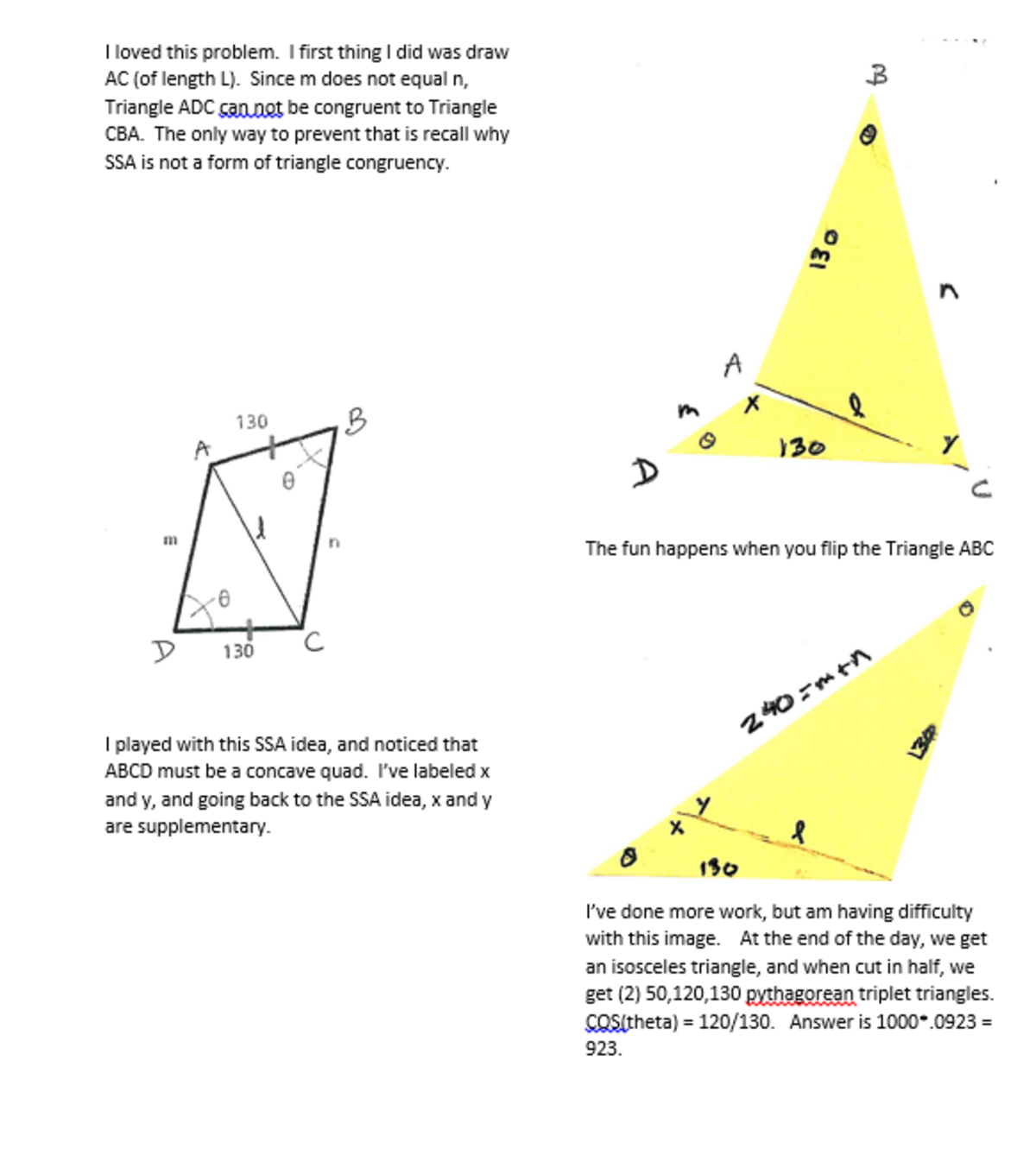
$\text{Let p be the diagonal through the vertices containing the unequal angles.}\\ \text{From the top triangle, applying Cos Rule, }p^2=130^2+n^2 -260*n*Cos\theta.\\ \text{From the bottom triangle, applying Cos Rule, }p^2=130^2+m^2 -260*m*Cos\theta.\\ \therefore ~m^2 - n^2= 260* (m - n)*Cos\theta.~ But~ m~\neq~ n. ~And ~ m+n=500 - 260=240.\\ \implies~Cos\theta=\dfrac{12}{13}. ~~~\therefore~\lfloor 1000*Cos\theta \rfloor ~=\Huge ~~~~923.\\ ~~\\$

This is hardly any different from the solution of Aditya Agarwal .