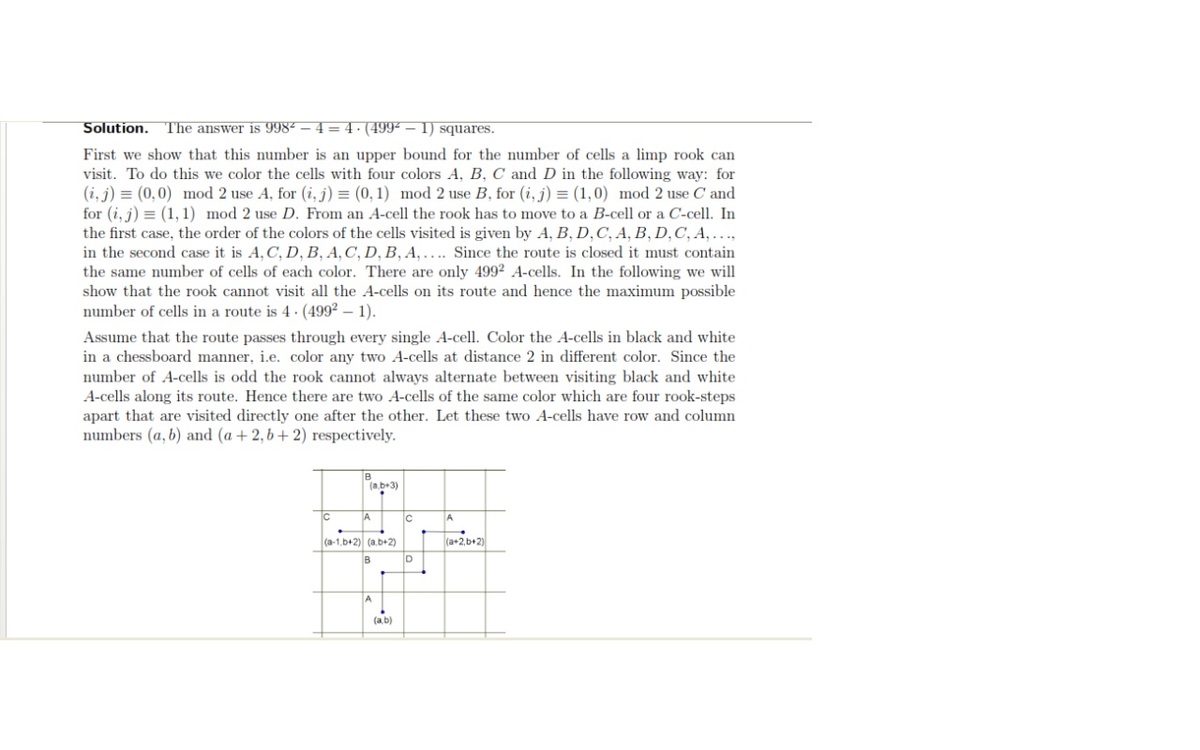
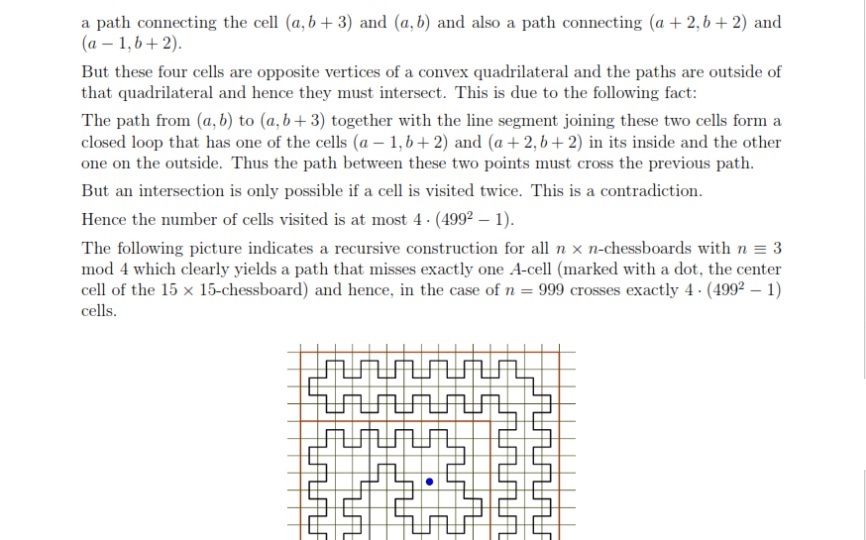
IMO Problem 5

On a $999 \times 999$ board a limp rook can move in the following way: From any square it can move to any of its adjacent squares, i.e. a square having a common side with it, and every move must be a turn: i.e. the directions of any two consecutive moves must be perpendicular. A non-intersecting route of the limp rook consists of a sequence of pairwise different squares that the limp rook can visit in that order by an admissible sequence of moves. Such a nonintersecting route is called cyclic, if the limp rook can, after reaching the last square of the route, move directly to the first square of the route and start over. How many squares does the longest possible cyclic, non-intersecting route of a limp rook visit?

This problem is from the IMO.This problem is from the IMO.This problem is part of this set .