Incenter and ratios

Since the incenter is the intersection of all the triangle's bisectors, then by the angle bisector theorem , $\frac{BI}{IM} = \frac{BC}{CM} = \frac{AB}{AM}$ and $\frac{CI}{IN} = \frac{BC}{BN} = \frac{AC}{AN}$ .

From $\frac{BI}{IM} = \frac{BC}{CM}$ we have $2 = \frac{4}{IM}$ or $IM = 2$ , and from $\frac{CI}{IN} = \frac{BC}{BN}$ we have $\frac{3}{2} = \frac{4}{BN}$ or $BN = \frac{8}{3}$ .

From $\frac{BI}{IM} = \frac{AB}{AM}$ we have $2 = \frac{AB}{AC - 2}$ , and from $\frac{CI}{IN} = \frac{AC}{AN}$ we have $\frac{3}{2} = \frac{AC}{AB - \frac{8}{3}}$ , and these two equations solve to $AB = 6$ and $AC = 5$ .

Thus $\triangle ABC$ has sides $4$ , $5$ , and $6$ , and by Heron's Formula its area is $A = \frac{15\sqrt{7}}{4}$ , so $a = 15$ , $b = 7$ , and $c = 4$ , and $15 + 7 + 4 = \boxed{26}$ .

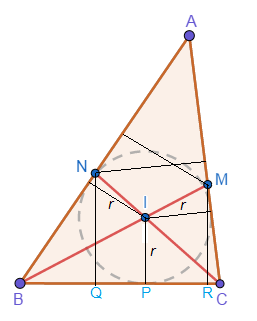
Let the inradius be $r$ and side lengths $BC=u=4$ , $AC = v$ , and $AB=w$ . Then the area of $\triangle ABC$ , $[ABC]=\dfrac 12(u+v+w)$ .

As $\triangle CIP$ and $\triangle CNQ$ are similar, we have $\dfrac {NQ}{IP} = \dfrac {CN}{CI} = \dfrac 53$ , $\implies NQ = \dfrac 53 r$ . Then area of $\triangle BCN$ , $[BCN] = \dfrac 56ru$ . Similarly, $[ACN] = \dfrac 56rv$ . Note that $[ABC]=[BCN]+[ACN] = \dfrac 56r(u+v)$ .

Similarly, $\triangle BIP$ and $\triangle BMR$ are similar, we have $\dfrac {MR}{IP} = \dfrac {BM}{BI} = \dfrac 32$ , $\implies MR = \dfrac 32 r$ . Then area of $\triangle BCM$ , $[BCM] = \dfrac 34ru$ . Similarly, $[ABM] = \dfrac 34rw$ . Note that $[ABC]=[BCM]+[ABM] = \dfrac 34r(u+w)$ .

Then, we have $[ABC] = \dfrac 56r(u+v) = \dfrac 34r(u+w) = \dfrac 12r(u+v+w)$ . Therefore,

$\begin{aligned} \frac 56(u+v) & = \frac 12 (u+v+w) & ...(1) \\ 5(u+v) & = 3(u+v+w) \\ \implies w & = \frac 23(u+v) \end{aligned}$

$\begin{aligned} \frac 34(u+{\color{#3D99F6}w}) & = \frac 12 (u+v+{\color{#3D99F6}w}) \quad ...(2) \\ 3\left(u+{\color{#3D99F6}\frac 23(u+v)}\right) & = 2 \left(u+v+{\color{#3D99F6}\frac 23(u+v)}\right) & \small \color{#3D99F6} \text{Since }w = \frac 23(u+v) \\ \implies v & = \frac 54 u = 5 \\ \implies w & = \frac 23(u+v) = 6 \end{aligned}$

By Heron's formula ,

$\begin{aligned} [ABC] & = \sqrt{s(s-u)(s-v)(s-w)} & \small \color{#3D99F6} \text{where }s = \frac {u+v+w}2 = \frac {15}2 \\ & = \sqrt{\frac {15}2 \cdot \frac 72 \cdot \frac 52 \cdot \frac 32} \\ & = \frac {15\sqrt 7}4 \end{aligned}$

Therefore, $a+b+c=15+7+4=\boxed{26}$ .

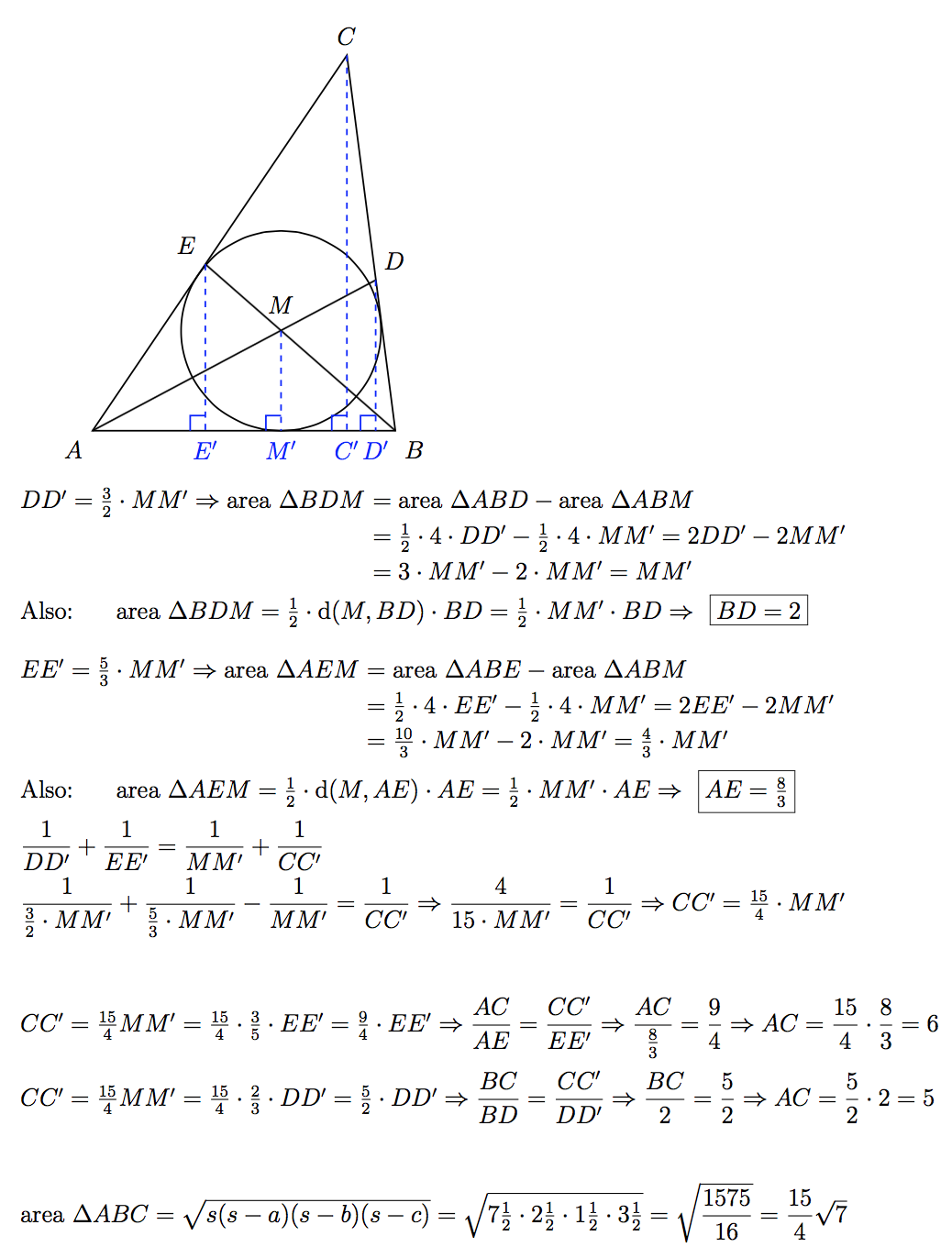
Let $C_1$ and $I_1$ be orthogonal projections of $C$ and $I$ , respectively, on side $AB$ . Then $CC_1 = h_c$ is the altitude of $\triangle ABC$ adjacent to side $AB$ and $II_1 = r$ is the radius of the incircle. Both of these lines are perpendicular to $AB$ so $CC_1 || II_1$ and $\triangle CC_1N \sim \triangle II_1N$ . By similarity:

$\dfrac{h_c}{r} = \dfrac{CC_1}{II_1} = \dfrac{CN}{IN} = \dfrac{CI + IN}{IN} = 1 + \dfrac{CI}{IN}$

We can find another relationship between $h_c$ and $r$ using formulas for area of $\triangle ABC$ :

$\dfrac{AB \times h_c}{2} = \dfrac{AB + BC + CA}{2}r \\ \dfrac{h_c}{r} = \dfrac{AB + BC + CA}{AB} \\ 1 + \dfrac{CI}{IN} = 1 + \dfrac{BC + CA}{AB} \\ \dfrac{CI}{IN} = \dfrac{BC + CA}{AB}$

Similarly, considering altitude from $B$ and point $M$ as in the figure in problem statement, we get:

$\dfrac{BI}{IM} = \dfrac{AB + BC}{CA}$

So far, we have: $\dfrac{BC + CA}{AB} = \dfrac{CI}{IN} = \dfrac{3}{2} \\ \dfrac{AB + BC}{CA} = \dfrac{BI}{IM} = 2$

Since we know that $BC = 4$ , solving this system of equations gives us: $AB = 6$ and $CA = 5$

Now we can find the area using Heron's formula: $\sqrt{S(S - AB)(S - BC)(S - CA)} = \boxed{\dfrac{15 \sqrt{7}}{4}}$ , where $S = \dfrac{AB + BC + CA}{2} = \dfrac{15}{2}$ is the semiperimeter of $\triangle ABC$ .

The answer is $15 + 7 + 4 = \boxed{26}$ .