Incircles on a regular tetrahedron
A regular tetrahedron has an edge length of . The incircles of two adjacent faces are drawn. Find the maximum distance between a point on the first incircle and a point on the second incircle. The maximum distance can be written as , where and are positive integers, and is square-free. Find .
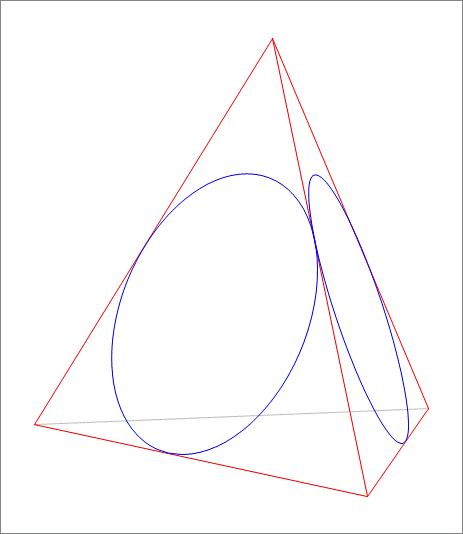
The answer is 52.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
and so x − y = 3 r ⎝ ⎛ sin θ ( 2 + cos ( s + t ) + cos ( s − t ) ) sin ( s + t ) − sin ( s − t ) cos θ ( cos ( s + t ) − cos ( s − t ) ) ⎠ ⎞ = 3 2 r ⎝ ⎛ sin θ ( 1 + cos s cos t ) cos s sin t − cos θ sin s sin t ⎠ ⎞