Incoming!

Here is a distance vs time graph of the scenario. The path of the ship is shown in blue, moving from A to E, while the path of the light rays moving from the ship to the Earth are shown in red.

Notice how the path of the light rays have a constant slope. This slope is the speed of light, $c$ .

This is all the working out shown in the image:

• The observed difference in time between the light rays is $\Delta t$ , so the line FE is also $\Delta t$ , as is BC. Note that this is not the same as AC, the time in which the ship has moved between the two observed distances.

• The ship moves a distance of $3c\Delta t$ between the two points, as given in the question, which is the length of CE.

• If the ship is moving at a constant speed $v$ , then we can find AC in terms of $v$ : $time=\frac{distance}{speed}$ $AC=\frac{3c\Delta t}{v}$ .

• The time taken for the first light ray to travel from A to F is represented by AB, which we can find knowing the speed of light, $c$ : $time=\frac{distance}{speed}$ $AB=\frac{3c\Delta t}{c}=3\Delta t$

Now we can form an equation with the lengths of the top lines: $AC=AB+BC$ $\frac{3c}{v}=3\Delta t+\Delta t$ $\frac{3c}{v}=3+1$ $v=\frac{3}{4}c$

My solution is based on the fact that while the light from the observations is moving towards the earth the spaceship is also getting closer. Let $D_1$ and $D_2$ be the first and second observed distances. The times taken for the light to reach the earth from these observations are $\frac{D_1}{c}$ and $\frac{D_2}{c}$ respectively

Then, if the speed of the spaceship is $v$ , the actual distances of the spaceship at the moment the observations are made are

$D_1-\frac{D_1}{c}v$ and $D_2-\frac{D_2}{c}v$

Take the difference of these to see that the actual distance travelled by the space ship between the observations is

$(1-\frac{v}{c})(D_1-D_2)$

We are told in the question that $D_1-D_2=3c \Delta T$ substituting this into the above expression and dividing by $\Delta T$ to find the velocity gives an easy equation -

$v=(1-\frac{v}{c})3c$

leading to the solution $\boxed{v=\frac{3c}{4}}$

By taking the initial time ( $t_0$ ) as the time when when we first observe the spaceship, then the second time we observe the spaceship it is $t_0 + \Delta t$ .
Therefore it has been $\frac {D}{c}$ time for the light to reach the observer at $t_0$ .
Then, in the time $\Delta t$ the spaceship travels for time $\frac {3c\Delta t}{v}$ , since it’s distance travelled is $3c\Delta t$ because:
$D - (D - 3c\Delta t) = 3c\Delta t$
After which its light then travels for time $\frac {D - 3c\Delta t}{c}$ .
Overall the time prior to $t_0$ at which the spaceship is at distance $D$ is:
$\frac {D}{c}$
and the time prior to $t_0 + \Delta t$ at which the spaceship is at distance $D - 3c\Delta t$ is:
$\frac {D - 3c\Delta t}{c} + \frac {3c\Delta t}{v}$
Therefore prior to $t_0$ at which the spaceship is distance $D - 3c\Delta t$ is:
$\frac {D - 3c\Delta t}{c} + \frac {3c\Delta t}{v} - \Delta t$
At which point the two values of time ellapsed prior to $t_0$ are equal and can be equated and rearranged for $v$ . This gives:
$\frac {D}{c} = \frac {D - 3c\Delta t}{c} + \frac {3c\Delta t}{v} - \Delta t$
Multiplying by $c$ and $v$ gets rid of fractions:
$Dv = Dv - 3cv\Delta t + 3c^2\Delta t - cv\Delta t$
Simplifying gives:
$4cv\Delta t = 3c^2\Delta t$
Cancelling out $\Delta t$ and $c$ results in:
$\boxed{v = \frac {3}{4}c}$ .

Assume that the second signal received was emitted at at time $\Delta \tau$ after the first signal was emitted. $\Delta \tau > \Delta t$ because the second signal took less time to get to the earth.

The time between the reception of the signals is $\Delta t = \Delta \tau + \frac{-\Delta D}c = (-\Delta D)\left(-\frac 1 v + \frac 1 c\right),$ where we used $\Delta D = -v\Delta \tau$ . Thus $\Delta D = -\frac{1}{\frac 1 v - \frac 1 c}\Delta t = -\frac{v}{c - v}c\Delta t.$ Since we are told that the right-hand side equals $-3c\Delta t$ , we conclude $\frac{v}{c - v} = 3 \ \ \therefore\ \ \ v = \boxed{\dfrac 3 4 c}.$

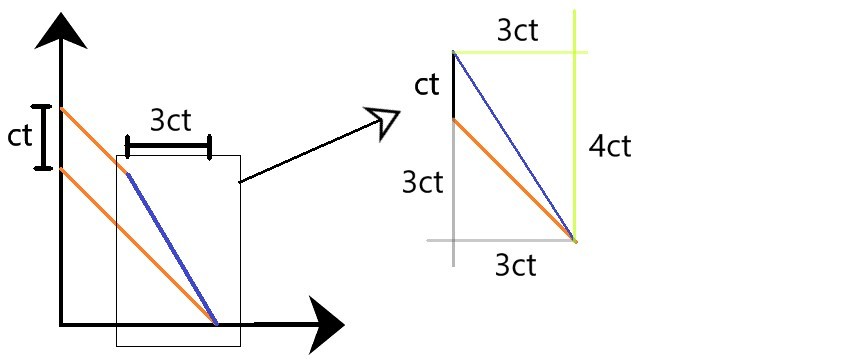
Compare the weak green lines, get $\frac{v}{c}=\frac{3}{4}$ .