An Infinity of Circles

In equilateral △ A B C above, extend the diagram to an infinite number of inscribed circles and let A n be the area of the n th inscribed circle.
If A △ A B C ∑ n = 1 ∞ A n = b a π , where a and b are coprime positive integers, find a + b .
The answer is 11.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
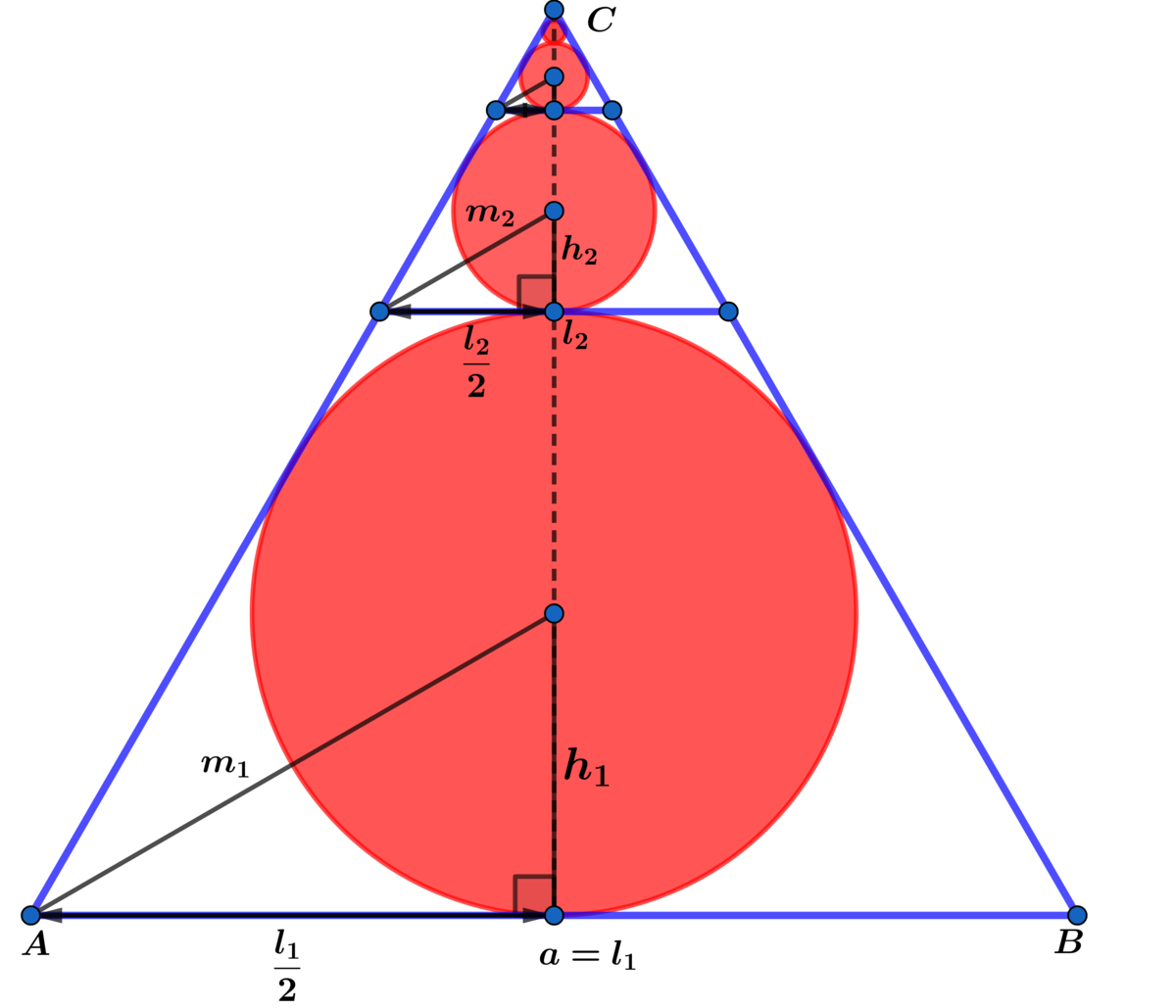
The height of △ A B C is H 1 = 2 3 a and 2 a = 2 3 m 1 ⟹ m 1 = 3 a ⟹ h 1 = 2 3 a
H 2 = H 1 − 2 h 1 = 2 3 a and H 2 H 1 = 3 = l 2 a ⟹ l 2 = 3 a ⟹ 6 a = 2 3 m 2 ⟹ m 2 = 3 3 a ⟹ h 2 = 6 3 a = 3 ( 2 3 ) a
Note: H 1 H 2 = 3 1 = l 1 l 2 , so where actually done here. I.E; h n = ( 2 3 a ) ( 3 n − 1 1 )
H 3 = H 2 − 2 h 2 = 6 3 a and H 3 H 2 = 3 = 3 l 3 a ⟹ l 3 = 9 a ⟹ 1 8 a = 2 3 m 3 ⟹ m 3 = 9 3 a ⟹ h 3 = 1 8 3 a = 3 2 ( 2 3 ) a
Containing in this manner we have:
h n = ( 2 3 a ) ( 3 n − 1 1 ) ⟹ A n = 1 2 a 2 π ( 9 n − 1 1 )
⟹ T = ∑ n = 1 ∞ A n = 1 2 a 2 π ∑ n = 1 ∞ 9 n − 1 1 = 1 2 a 2 π ( 8 9 ) = 3 2 3 π a 2
and A △ A B C = 4 3 a 2
⟹ A △ A B C T = 8 3 π = b a π ⟹ a + b = 1 1
Rocco,
This is just a suggestion.
I was curious to solve a series of problems in String art mathematical patterns and post those problems on brilliant.
Here is a link
https://dumielauxepices.net/drawn-pattern/drawn-pattern-straight-line
It will be nice if we can start posting problems in these.
Especially, problems like:
What is the area of the shield formed by three parabola-string-art patterns within an equilateral triangle given the dimensions of the sides of the equilateral triangle etc..
Cheers Vijay
https://dumielauxepices.net/wallpaper-713012
I checked it out. Sounds like a good idea. It's new to me. This is the first time I heard of it.
But with your skill level and imagination, you will surely come up with a lot of such problems which can all be part of a new set of problems.
This is, in fact, the same solution as Dr. Otto Bretscher's. It is just expressed differently: 3 3 π ∑ n = 1 ∞ ( 3 n − 1 1 ) 2 = 8 3 π .
Make the side of the triangle 2 3 , for convenience, so that A △ = 3 3 . The radius of the largest circle is r 1 = 1 , and the second circle is the incircle of an equilateral triangle with height 1, so r 2 = 3 1 . Likewise, r n = 3 n 1 . Now ∑ n = 1 ∞ π r n 2 = 1 − 9 1 π = = 8 9 π and the ratio we seek is 8 3 π . The answer is 1 1 .