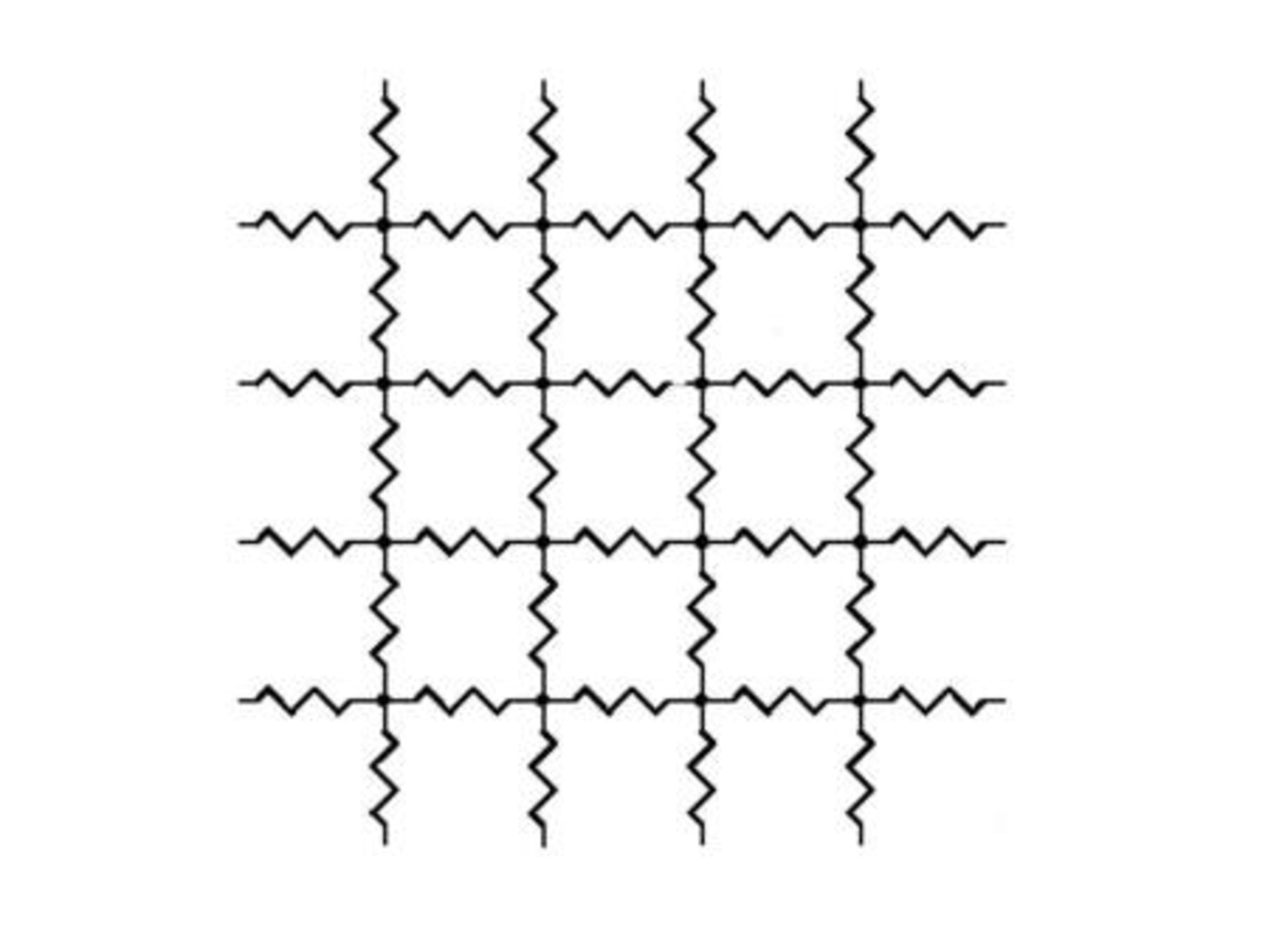
Infinite grid

The quickest way I know to get the answer uses the Principle of Superposition

Consider two copies of the grid, both earthed at infinity. Imagine injecting a current of 1 Amp into the grid through a wire attached to node A as shown in the top left of the picture. The current will run to earth through the grid, but, by symmetry, it is obvious that 1/4 of the current entering the grid through A must travel through each of the 4 wires radiating out from it. Each of the wires leading out from A therefore carries 0.25 Amps in this solution.

Now, forget about A, but attach a wire to B and pull out 1 Amp from the earth (at infinity). By a similar argument to the first diagram, 0.25 Amps must be flowing into B along each of the wires connected to it in the directions shown.

We now have two perfectly good solutions for currents flowing in resistive networks. The principle of superposition says that if we add the two solutions we also get a solution. Adding the two configurations above means that the resistor joining A to B must be carrying 0.5 Amp (0.25 from the first solution and 0.25 from the second, both in the same direction). But this is a 1Ω resistor so the Voltage across AB must be 0.5 V.

Now think of the whole mesh as being a black box in between the input wire and output wire. This black box has a current of 1 Amp flowing through it and the voltage dropped is 0.5 V. It’s resistance is therefore 0.5 Ω.!