Infinite oscillating springs
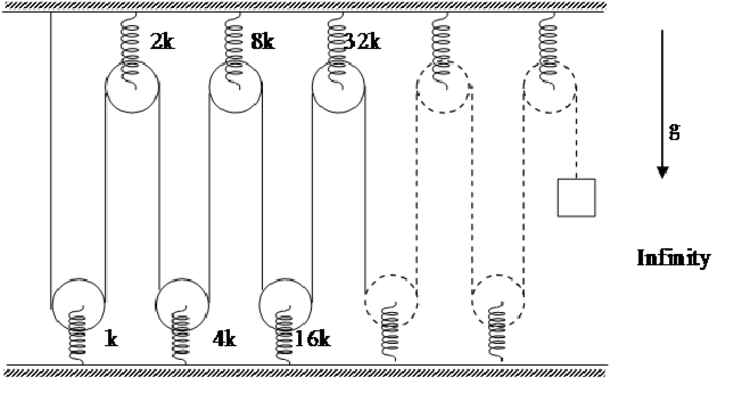 There is a system of infinite pulleys and springs as shown in the figure above. Spring constants follows a geometric progression,
. All the pulleys are massless and frictionless. Find the time period of oscillation.
There is a system of infinite pulleys and springs as shown in the figure above. Spring constants follows a geometric progression,
. All the pulleys are massless and frictionless. Find the time period of oscillation.
Take the mass of the block to be . If the answer is of the form , where is a natural number, submit your answer as .
The answer is 8.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Can this be the solution....
We have.... By constraint equation
x = 2 x 1 + 2 x 2 + 2 x 3 + . . . ∞
k e q T = k 4 T + 2 k 4 T + . . . ∞
⟹ k e q 1 = k 8
So T = 2 π m 8 k