Infinite Potential
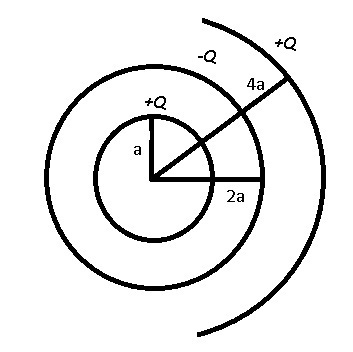 An infinite number of concentric rings carry a charge
each alternatively positive and negative. Their radii are
in geometric progression as shown. where
. The potential at the center of the rings will be?
An infinite number of concentric rings carry a charge
each alternatively positive and negative. Their radii are
in geometric progression as shown. where
. The potential at the center of the rings will be?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Potential at the centre is given by :
V = 4 π ϵ 1 ( a Q − 2 a Q + 4 a Q − ⋯ )
⟹ V = 4 π ϵ a Q ( Infinite GP 1 − 2 1 + 4 1 − ⋯ )
⟹ V = 4 π ϵ a Q ( 1 + 2 1 1 ) = 4 π ϵ a Q 2 3 = 6 π ϵ Q