Infinite Square-Circle Inscription
This figure is a square of side length in which is inscribed a circle, in which is inscribed a square ... and so on forever.
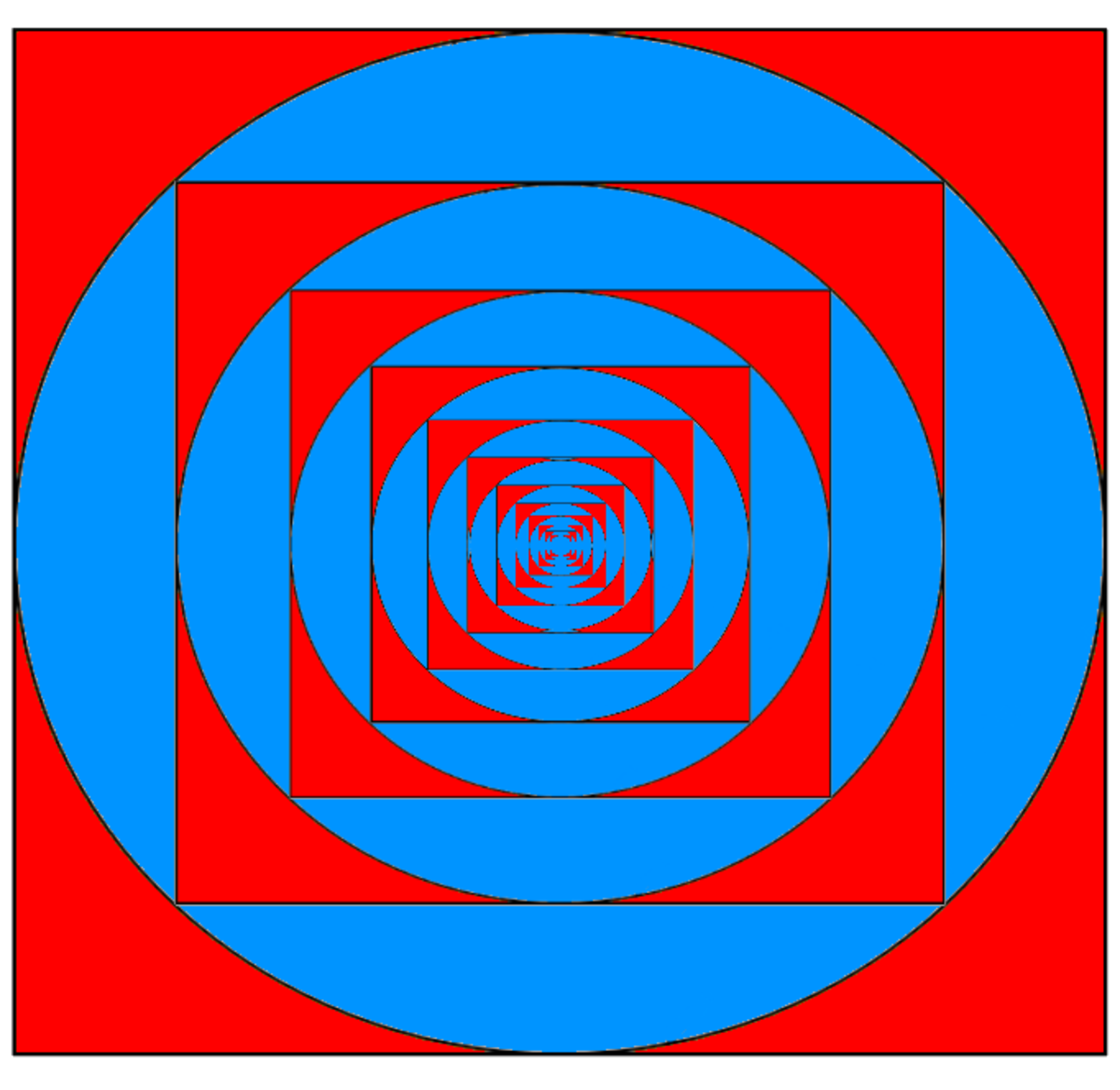
Which area is bigger ?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
If the side of the biggest square is a , then the biggest circle has radius 2 1 a , and the side of the second-biggest square is a / 2 .
The area of the biggest square is a 2 ; that of the biggest circle, 4 1 π a 2 ; that of the second-biggest square is 2 1 a 2 .
If we take the second-biggest square and all that is inside it, we have a square ring with area a 2 − 2 1 a 2 = 2 1 a 2 . The blue region in this ring has area 4 1 π a 2 − 2 1 a 2 . Therefore the blue fraction of this square ring is a 2 − 2 1 a 2 4 1 π a 2 − 2 1 a 2 = 2 − 1 2 1 π − 1 = 2 1 π − 1 ≈ 5 7 % , which is greater than 5 0 % . Therefore the outer blue region is slightly bigger than the outer red region; the same holds true for all blue and red regions, because of similarity.
Thus, the blue region is greater than the red region.