Awesome geometry -4
In a right angled triangle △ A B C with ∠ C = 9 0 ∘ with r + r A + r B = r C = 3 5 .
Find the perimeter of △ A B C .
Details and Assumptions :
-
r is inradius.
-
r A , r B , r C are radii of excircles opposite to vertices A , B , C .
The answer is 70.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
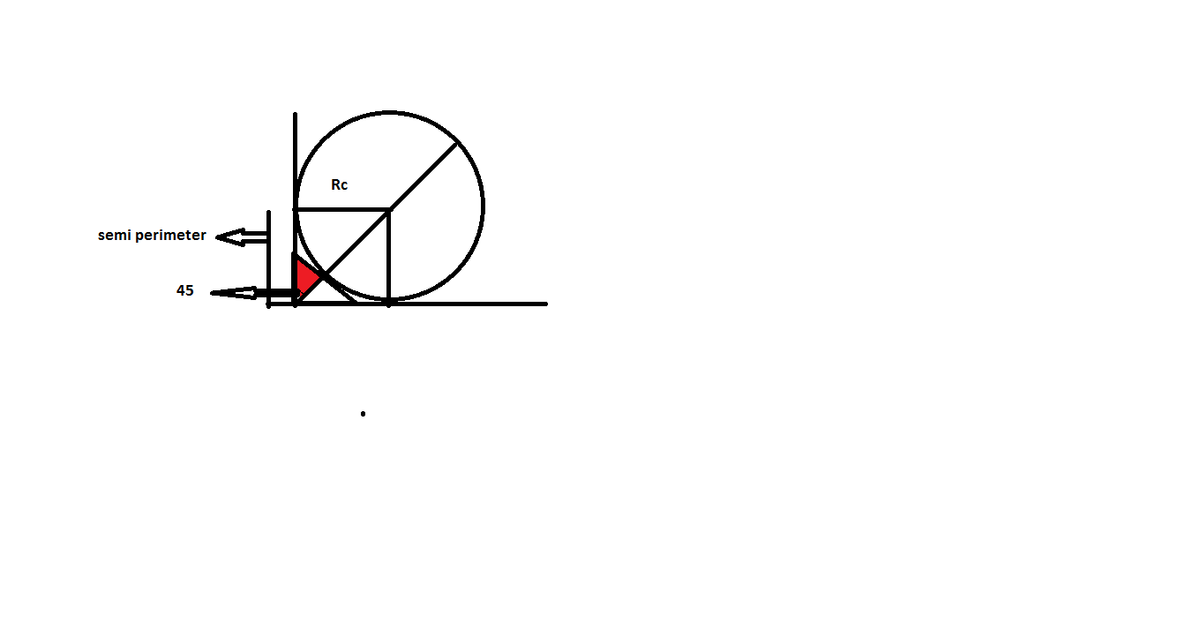 semiperimeter=Rc
we simply use the fact that excenter lie on internal angle bisector of C and use a little geometry.
semiperimeter=Rc
we simply use the fact that excenter lie on internal angle bisector of C and use a little geometry.
The first part is/was unnecessary. rc=0 was enough
Well it must be mentioned that it is right angled at C.
Anyways in a right triangle.
s ( s − c ) = Δ where s is the semi permeter.
Also r c = s − a Δ
Rest is self explanatory.
Actually , I had mentioned that earlier. But someone from the moderators has edited this question.