Inradius of a regular Octahedron

Triangle $ABC$ , where $A$ is a vertex, $B$ is a mid-point of a side, and $C$ the center of the octahedron, has a right angle at $C$ and sides $CB=\frac12$ and $AB=\frac{\sqrt3}2$ .

Point of contact between the sphere and the octahedron, $D$ is in the center of the face, therefore the median $AB$ is divided in the ration of $2:1$ making $DB=\frac13\cdot AB=\frac1{2\sqrt3}$ .

Triangle $CBD$ has a right angle at $D$ , so $CD=\sqrt{CB^2-DB^2}=\sqrt{\frac14-\frac1{12}}=\boxed{\frac1{\sqrt6}}$ .

Let edge length be $1$ . Then the distance from the center of any square to the edge is $\dfrac{1}{2}$ . The distance from the center of any equilateral triangle to the edge is $\dfrac{1}{2\sqrt{3}}$ . Then we have for the inradius

$\sqrt{ ( \dfrac{1}{2} )^2 - ( \dfrac{1}{2\sqrt{3}} )^2 } = \dfrac{1}{\sqrt{6}}$

1/√6

Using a unit octahedron the radius and the ratio are the same number. That number can be found using several steps of basic geometry as shown below.

The diameter of the sphere must be smaller than the length of the edge. So the double of an answer must be smaller than 1 to be valid. There is only one such answer.

A regular octagon is most easily described by placing vertices at the points $(\pm 1, 0, 0),\,(0, \pm 1, 0),\,(0, 0, \pm 1)$ .

The side length is the distance between $(0,1,0)$ and $(1,0,0)$ (for instance): $d = \sqrt{1^2 + 1^2} = \sqrt 2$ .

The faces may be described by equations of the form $\pm x \pm y \pm z = 1$ . Symmetry shows that the sphere touches each face at $\pm x = \pm y = \pm z$ , which is therefore equal to $\tfrac13$ . This means that the radius of the sphere is $r = \sqrt{(\tfrac13)^2 + (\tfrac13)^2 + (\tfrac13)^2} = 1/\sqrt 3.$ Thus $\frac r d = \frac{1/\sqrt 3}{\sqrt 2} = \frac{1}{\sqrt 6}.$

Just look at one of the face of octahedron, let its side length be $\sqrt2a$ then the length from the center of octahedron to one of its verticle will be $a$ .

Then, by setting the origin of 3d-coordinate system be the center of octahedron, and let $x,y,z$ axis point to three of its verticles, then the plane equation will be $x+y+z=a$ , so the distance from origin to the plane must be $\displaystyle r=\bigg|\frac{0+0+0-a}{\sqrt{1^2+1^2+1^2}}\bigg|=\frac{a}{\sqrt3}$ , after compare, we get the ratio of radius to its side length be $\boxed{\frac1{\sqrt6}}$ .

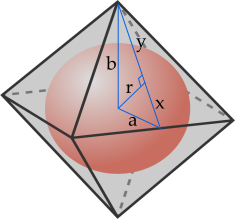
Purely Pythagorean: if side length is 1,

$\begin{aligned} a &= \frac{1}{2} \\ x + y &= \frac{\sqrt{3}}{2} \\ b &= \frac{1}{\sqrt{2}} \\ r^2 &= a^2 - x^2 \\ &= \frac{1}{4} - (\frac{\sqrt{3}}{2} - y)^2 \\ &= y\sqrt{3} - y^2 - \frac{1}{2} \\ r^2 &= b^2 - y^2 \\ \frac{1}{2} - y^2 &= y\sqrt{3} - y^2 - \frac{1}{2} \\ y &= \frac{1}{\sqrt{3}} \\ r &= \frac{1}{\sqrt{6}} \end{aligned}$

Solution