Insane-gon

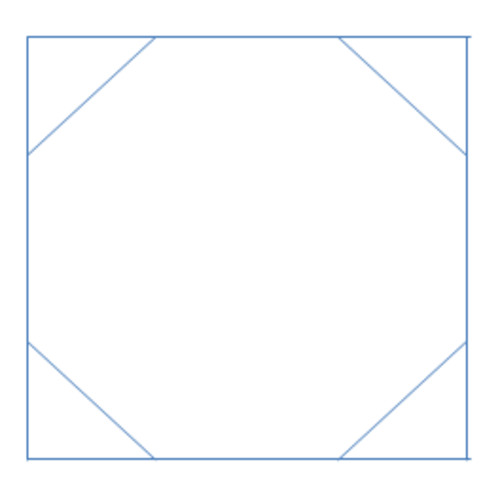The area of a square can be easily found by squaring the length of one of its sides. Now, Imagine a regular octagon inside the square. (As shown in the picture above) Now, imagine a regular 16-gon inside the octagon. (The same way the octagon was inscribed inside the square). Then, imagine a regular 32-gon inside the 16-gon. Continue this process until you reach a regular $1073741824$ -sided figure. What is the area of this $1073741824$ -sided figure given that the area of the square is 16 $cm^{2}$ ?

Clarification

All of the shapes that will be inscribed will be regular i.e. all sides are the same.

Please leave your answer rounded off to two decimal places.