Inscribed Rectangle

Geometry Level 3

A rectangle is inscribed within a circle of radius $7\text{ cm}$ , and this rectangle has a perimeter $32\text{ cm}$ . What is the area of this rectangle?

28\text{ cm}^2

30\text{ cm}^2

35\text{ cm}^2

36\text{ cm}^2

49\text{ cm}^2

1 solution

Isaac Reid
Sep 14, 2016

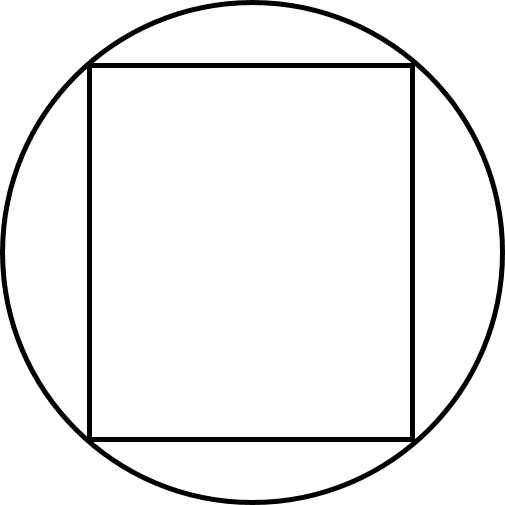
This is the most elegant solution I can think of. Consider the diagram below.

Firstly, note that $2a+2b=32$ from the perimeter of the rectangle. It follows that $a+b=16$ and thus $(a+b)^2=16^2=256$ . Multiplying out the brackets yields $a^2+2ab+b^2=256$

The diagonal of the rectangle is equal to double the radius: $2\times7=14$ . Using Pythagoras' theorem yields: $a^2+b^2=14^2=196$ .

Subtract the second equation from the first and you find: $2ab=256-196=60$ . Therefore, $ab=30$ . This is the area of the rectangle, so the answer is $30$ .

Best solution I have ever read.

Fin Moorhouse - 4 years, 9 months ago

Elegant :) i got their using x and 16-x and pythagoras

Peter van der Linden - 4 years, 9 months ago

Which program did you use to create these images? :)
Also, maybe this solution should include a proof for the diagonal of the rectangle being the diameter of the circle?

Jesse Nieminen - 4 years, 9 months ago

Microsoft Powerpoint. That's a good idea - I'll do it when I have time.

Isaac Reid - 4 years, 9 months ago

If you didn't notice, you can prove it in one sentence using Thales' theorem .

Jesse Nieminen - 4 years, 9 months ago

This is the method I used.

Andre Bourque - 4 years, 8 months ago

Inscribed Rectangle

1 solution

0 pending reports