Inspired By A JEE 2014 Question

Electricity and Magnetism Level 4

The radiation corresponding to the $2\rightarrow 1$ transition of hydrogen atom falls on a metal surface to produce photoelectrons. These electrons are made to enter an electric field of $10^{-5} \text{ N/C}$ as shown in the figure. If the electrons just change their direction of motion after 1 second, then the work function of the metal is close to which of the following choices?

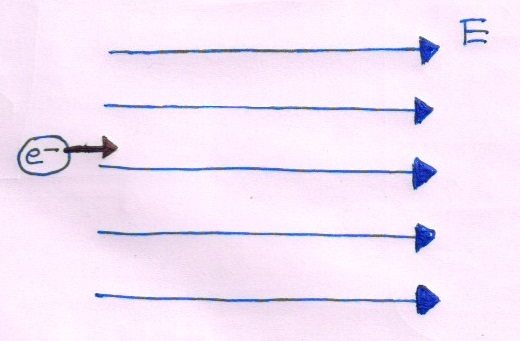
Details and Assumptions :

Take the mass of electron, $m_{e}=9.1\times 10^{-31}\text{ kg}$ ,
The charge on electron, $e=-1.6\times 10^{-19}\text{ C}$ and $1\text{ eV}=1.6\times 10^{-19}\text{ J}$ .
The Ionization energy of Hydrogen atom in ground state is $13.6 eV$

0.8\text {eV}

1.8\text {eV}

1.1\text {eV}

1.4\text {eV}

1 solution

Arjen Vreugdenhil
Mar 26, 2016

The acceleration of the electron is $a = -\frac{eE}{m_e}.$ If it reaches zero speed after time $t$ , it's initial velocity must have been $v_0 = -at = \frac{eEt}{m_e},$ which means it had a kinetic energy of $K_0 = \tfrac12m_ev^2 = \frac{e^2E^2t^2}{2m_e} = 8.8\:e\ \text{V}.$ (To find the energy in eV, I simply left one factor $e$ out.)

The photon that was released had energy $E_\gamma = -(E_1 - E_2) = \frac{\text{Ry}}{1^2} - \frac{\text{Ry}}{2^2} = \tfrac34\text{Ry},$ where $\text{Ry} = 13.6\ \text{eV}$ is the well-known ionization energy of the electron in hydrogen in its ground state. The work function of the metal, then, is $\phi = E_\gamma - K_0 = 10.2\ \text{eV} - 8.8\ \text{eV} = \boxed{1.4}\ \text{eV}.$

Nice solution!

Sahil Bansal - 5 years, 2 months ago

Inspired By A JEE 2014 Question

1 solution

0 pending reports