Integral Volume of a Solid
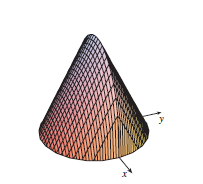
The figure above shows a solid with a circular base of radius 1. Parallel cross sections perpendicular to the base are equilateral triangles. The volume of the solid can be written as B A B , where A and B are positive coprime integers.
Find A + 2 B .
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
The function for a circle with radius one centered at the origin is,
x 2 + y 2 = 1
Note that this is half the height of each equilateral triangle.
The area of an equalterial triangle,
A = 4 3 s 2
Each base of the triangle can be represented by the unit circle function above. As the height of the circle centered at 1 only gives us half of the base of the triangle, we have to double it. Thus, the area of each cross section at point x on the circle is,
A = 4 3 ( 2 ∗ 1 − x 2 ) 2
We now want the sum of all of these cross-sections times an infitesimal Δ x ,
lim Δ x → 0 ∑ i = 1 n 4 3 ( 2 ∗ 1 − i 2 ) 2 Δ x = ∫ − 1 1 4 3 ( 2 ∗ 1 − x 2 ) 2 d x
The function is even,
2 ∫ 0 1 3 ( 1 − x 2 ) d x = 3 4 3
2 ∗ ( 3 ) + 4 = 1 0
Using x 2 + y 2 = 1 as the equation of a unit circle, and 4 3 s 2 as the area of an equilateral triangle with side s , the volume of the solid is ∫ − 1 1 4 3 ( 2 1 − x 2 ) 2 = 3 4 3 , so A = 4 , B = 3 , and A + 2 B = 1 0 .
To do it like this, you have to account for the y value of the unit circle function being only half of the entire length of the base of each triangle. This integral comes out to 1/3 * sqrt(3) not 4/3 * sqrt(3). The four would come from a two being inside the squared quantity of the integrand.
Log in to reply
I edited my solution. Thanks for pointing that out!
Let's take circle as x 2 + y 2 =1 centered at origin, since all cross sections are equilateral triangles , y = 1 − x 2 will be half of the base length of any triangle,
4 y 2 − y 2 =height of triangle= 3 y ,
Area(x) = 2 1 3 y 2 y = 3 y 2
∫ − 1 1 A r e a ( x ) d x =Integral volume of triangles=Volume of the solid
∫ − 1 1 3 ( 1 − x 2 ) d x = − 3 ( 3 x 3 − x ) ∣ ∣ ∣ ∣ ∣ − 1 1 = 4 3 3