This Semis -- I Mean Seems Familiar

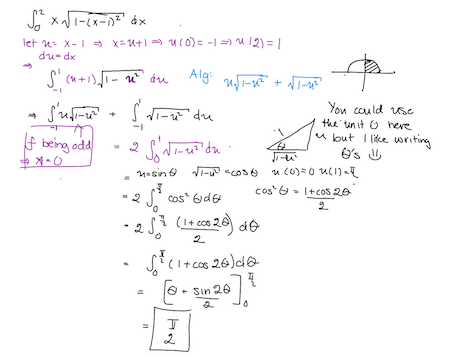
First let $u = x - 1.$ Then $x = u + 1, du = dx$ and as $x: 0 \rightarrow 2$ we have $u: -1 \rightarrow 1.$ The integral then becomes

$\displaystyle\int_{-1}^{1} (u + 1)\sqrt{1 - u^{2}} du = \int_{-1}^{1} u\sqrt{1 - u^{2}} du + \int_{-1}^{1} \sqrt{1 - u^{2}} du.$

Now the integrand of the first of these integrals is an odd function, and since we are integrating from $-1$ to $1,$ this integral evaluates to $0.$ Thus we just need to focus on the second of these integrals.

Letting $u = \sin(\theta),$ we have that $du = \cos(\theta) d\theta$ and $\sqrt{1 - u^{2}} = \cos(\theta).$ The (indefinite) integral then becomes

$\displaystyle\int \cos^{2}(\theta) d\theta = \dfrac{1}{2} \int (1 + \cos(2\theta)) d\theta =$

$\dfrac{1}{2}(\theta + \sin(\theta)\cos(\theta)) = \dfrac{1}{2}(\arcsin(u) + u\sqrt{1 - u^{2}}),$

which when evaluated from $-1$ to $1$ yields $\dfrac{1}{2}((\arcsin(1) + 0) - (\arcsin(-1) + 0)) =$

$\arcsin(1) = \dfrac{\pi}{2} = \boxed{1.57}$ to 2 decimal places.