Intelligent Orthocenter
In a triangle A B C , if the altitude drawn from vertex A meets the circumcircle at a point P = ( 1 , 5 ) and the equation of the side B C is, x + y + 4 = 0 . Then the orthocenter , H is ( a , b ) . Find ∣ a + b ∣ .
The answer is 14.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
There's a direct formula for finding the image of a point ( x 1 , y 1 ) w.r.t. the line L : a x + b y + c = 0 which is
a x − x 1 = b y − y 1 = a 2 + b 2 − 2 ( a x 1 + b y 1 + c )
I hope this approach would be helpful since you already know the property that the image of the orthocenter w.r.t. any side of the triangle lies on the circumcircle of the triangle, so there's no need to find the foot of the perpendicular of the point to the line as you've done.
P.S. I loved your handwriting. Such a nice and neat solution. (+1) for this. Kudos!
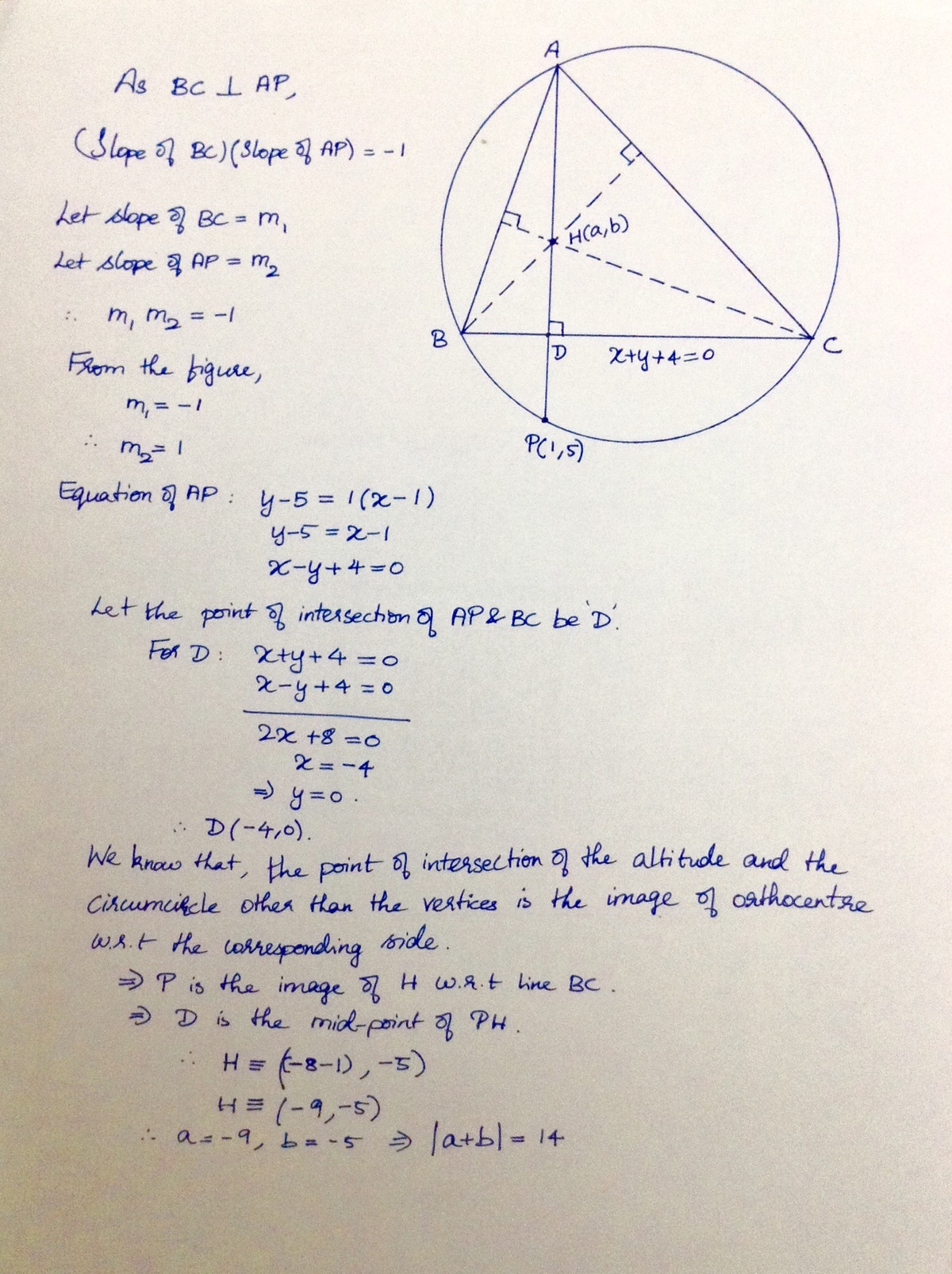
Orthocenter and point of intersection of altitude and circumcircle are always equidistant from the foot of the given altitude. In our case we are looking for a point on perpendicular line to BC: − x + y − 4 = 0 . The foot of the altitude from A is at D ( − 4 , 0 ) .
a = − 4 − ( 1 − ( − 4 ) = − 9 , b = ( 0 − ( 5 − 0 ) = − 5 ⇒ H ( − 9 , − 5 )
⇒ a + b = − 1 4 ⇒ ∣ a + b ∣ = 1 4 .