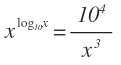Interesting log

first, multiply bot sides with $x^3$ $x^{log_{10}(x) +3}=10^4$ take log in base x in both sides $log_{10}(x)+3=log_x (10^4)$ now, change the base of log $log_x (10^4)= \dfrac{log_{10}(10^4)}{log_{10} (x)}=\dfrac{4}{log_{10} (x)}$ puting the value in the original equation, we get, $log_{10}(x)+3=\dfrac{4}{log_{10}(x)}$ let $log_{10}(x)=z$ $z+3=\dfrac{4}{z}\longrightarrow z ^2+3z-4=0\longrightarrow z=1,3$ since $log_{10}(x)=z$ $log_{10}(x)=1,3\longrightarrow x= \boxed{10,30}$

• Lets take log to the base 10 on both sides of the equation. The result will be:
  $[log(x)]^{2} = -3log(x) + 4$ . $[log(x)]^{2} + 3log(x) - 4 = 0$ .
  
• Now, lets assume $log(x) = Y$ .
• As you see, we have a second degree equation $Y^{2} + 3Y - 4 = 0$ .
• Solving with Bhaskara's, we have:
  
  

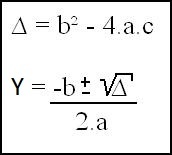
• Replacing terms, we find {Y¹ = 1 and {Y² = -4 .

• Afterwards, we have {log(x) = 1 => x = 10^{1} and {log(x) = -4 => x = 10^{-4} .

• Multipling x¹ and x² , we have the product of the roots of the equation is $10^{1}\times10^{-4} = 10^{-3} = 0.001$ .