Intersection of right triangles
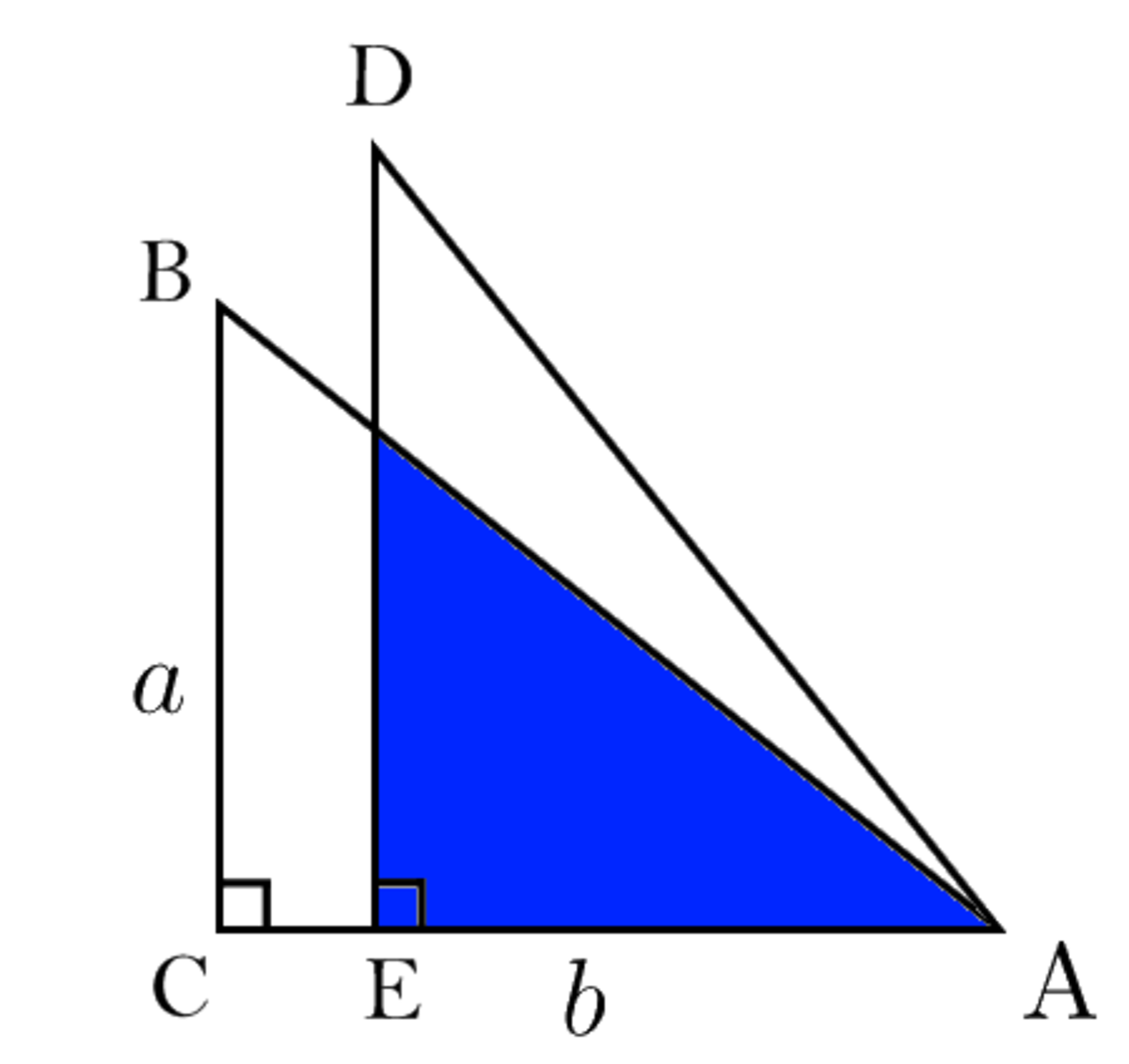
is a right triangle such that and with
is a point on segment such that triangles and are congruent. The area of their intersection (in blue) can be expressed as where are rational numbers.
What is
The answer is -1.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Be F the point of intersection of the segments A B and D E . The △ A B C is similar to the △ A E F . We can get this relation:
The segment A E has a length of a , and the segment E F has a length of x . We need to find the value of x in terms of a or b . How the triangles is similar the following relationship is valid:
A C B C = A E E F and with it we can find x :
b a = a x
b x = a 2
x = b a 2
The area of the blue triangle is 2 E F ⋅ A E . Then, 2 b a 2 ⋅ a = 2 b a 3 = a 3 ⋅ b − 1 ⋅ 2 1 . The answer must be 3 ⋅ ( − 1 ) ⋅ 2 1 = − 1 . 5