Intersection points on circle
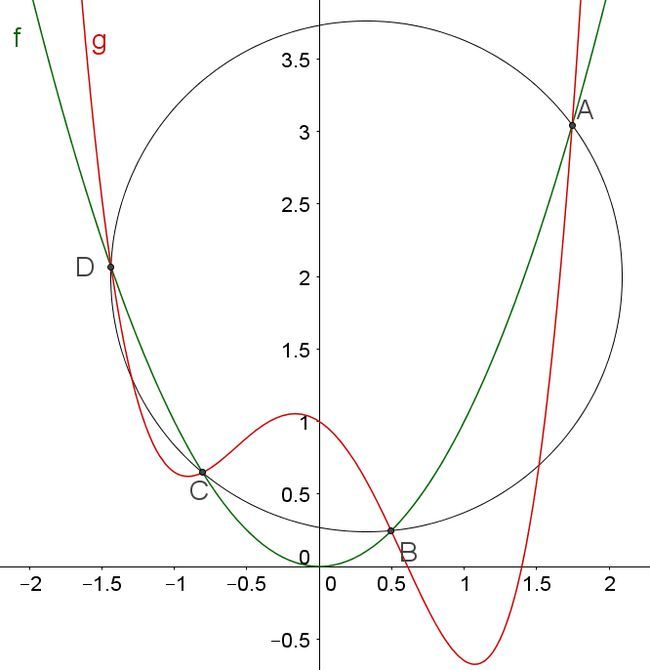 The parabola
intersects the graph of
at four distinct points. These four points on a same circle. Find the value of
.
The parabola
intersects the graph of
at four distinct points. These four points on a same circle. Find the value of
.
This problem is part of Curves... cut or touch?
The answer is 0.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Suppose the equation of the circle is ( x − h ) 2 + ( y − k ) 2 = r 2
Since it intersects with the graph f ( x ) = x 2 , the above equation can be rewritten as ( x − h ) 2 + ( x 2 − k ) 2 = r 2
Note that the coefficient of x 3 of above quartic equation is 0.
Let the x -coordinates of 4 intersection points be α 1 , α 2 , α 3 and α 4 . Then α 1 + α 2 + α 3 + α 4 = 0 . On the other hand, from g ( x ) = x 4 + a x 3 − 2 x 2 + b x + 1 , the sum of roots is − 1 a . So a = 0 and hence ⌊ 1 0 0 0 a b ⌋ = 0 .