Inverse Integrals

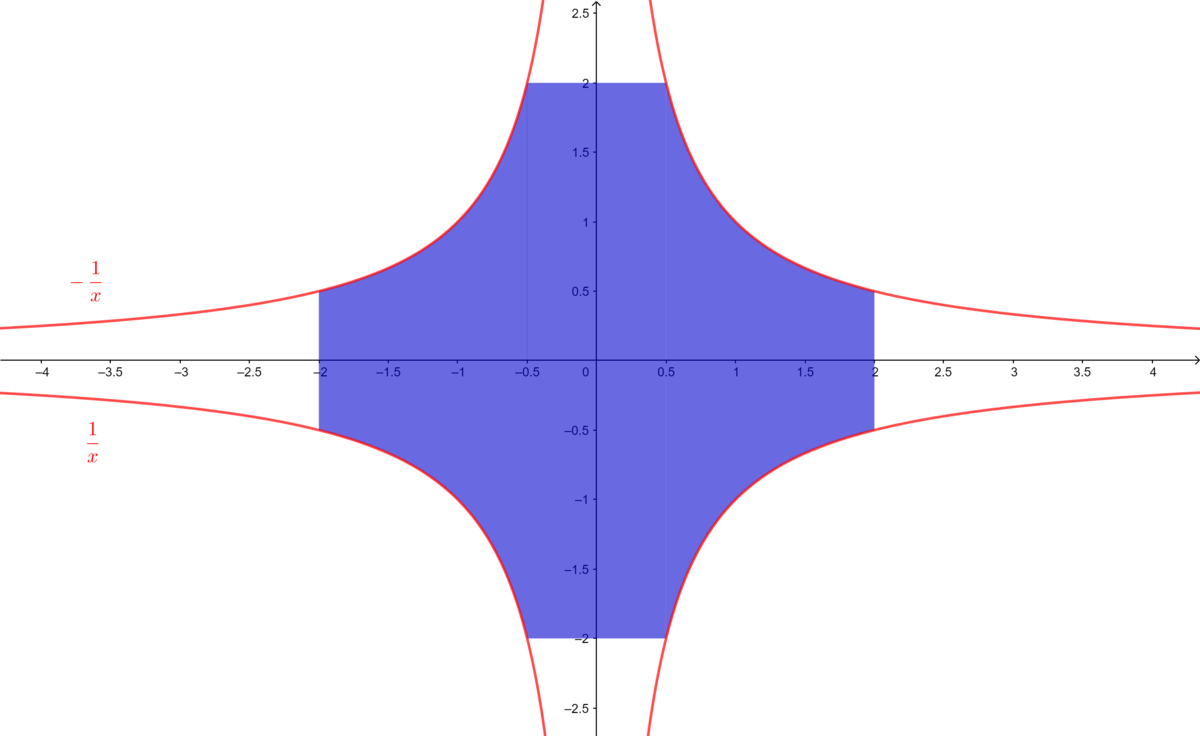
The formula for the area under the inverse function $\pm\ \frac{1}{x}$ with a range and domain constrained by $-a \leq x,y \leq a$ can be defined as:

$\frac{1}{4}\ Area = 1 + \int_{1/a}^{a} \frac{1}{x}\ dx$

Where the 1 prior to the integral is found by taking the area of the rectangle where $f(x)=a,\ f(a)=1/a,\ \therefore A_{rec} = 1$

Solving yields:

$\frac{A}{4}=\ln{x}|_{1/a}^{a}=\ln{a}-\ln{(1/a)}$

$Area = 4(1+2\ln{a})$

$\therefore A = 4(1+2\ln{2}) \approx 9.5452$