irregular octagon
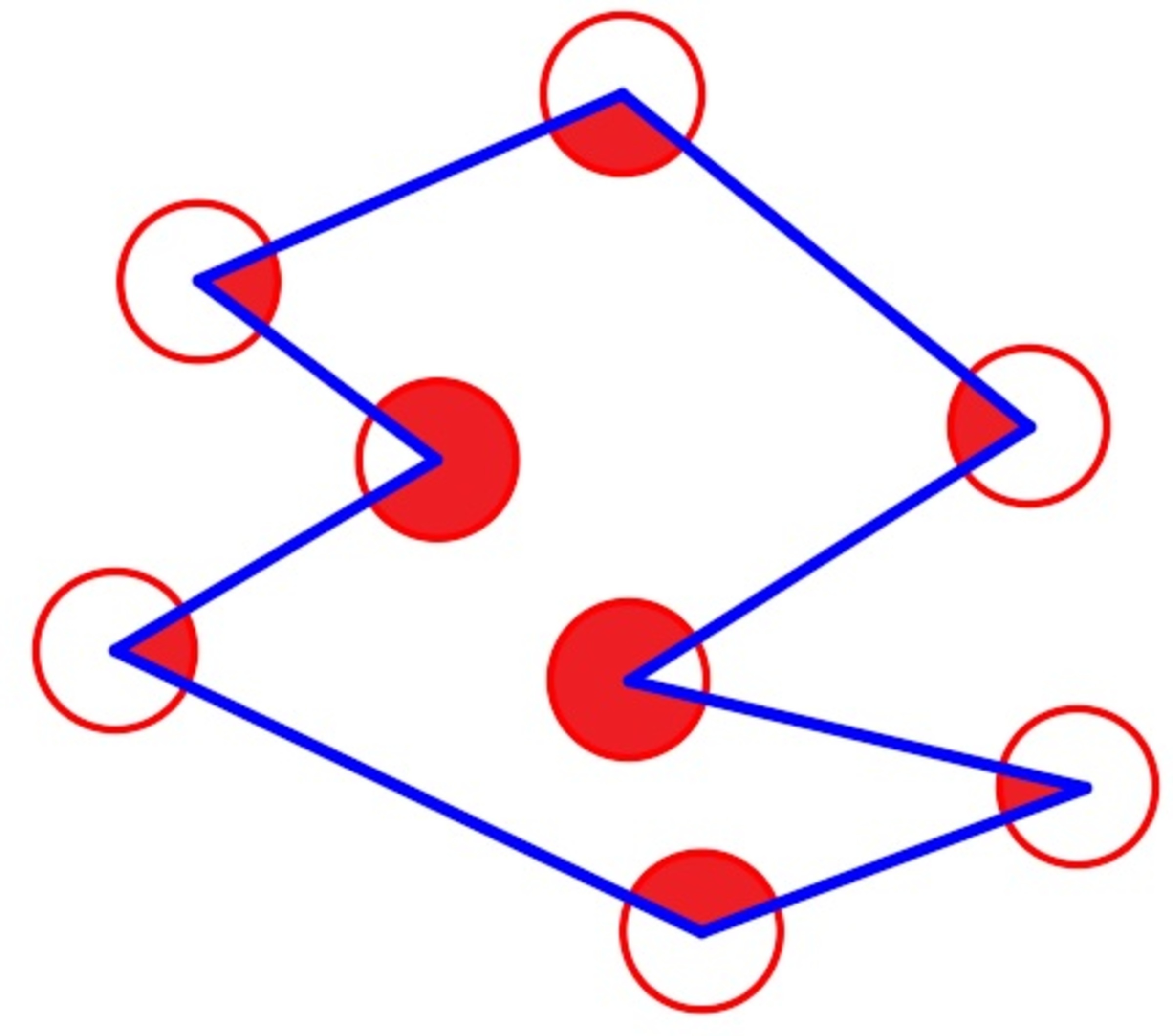 Circles of radius 2 cm are centered on the vertices of the blue irregular octagon shown. Using
, find the total area of the red regions in square centimeters.
Circles of radius 2 cm are centered on the vertices of the blue irregular octagon shown. Using
, find the total area of the red regions in square centimeters.
The answer is 37.68.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The sum of the interior angles of a polygon is ( n − 2 ) ( 1 8 0 ) where n is the number of sides. So the sum of the interior angles of the figure is ( 8 − 2 ) ( 1 8 0 ) = 1 0 8 0 . We can use the principle of the area of a circular sector: the area of a circular sector is 3 6 0 θ π r 2 where r is the radius of the circle. We have
area of the red regions = 3 6 0 1 0 8 0 ( 3 . 1 4 ) ( 2 2 ) = 3 7 . 6 8 square centimeters