Is it generalizable?
Consider the following integral
∫ 0 π sin 2 0 ( x + sin ( 1 5 x ) ) d x = B A π
where A and B are relatively coprime integers. Find A + B .
Extra work: Generalize the following integral ∫ 0 π ( sin ( x + sin ( D x ) ) ) 2 n d x ∀ n ∈ N where D is always an odd positive integer.
The answer is 308333.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
@Mark Hennings Sir, how do you add a box around your final answer ??
Log in to reply
In math mode, the command \boxed{…} does the trick.
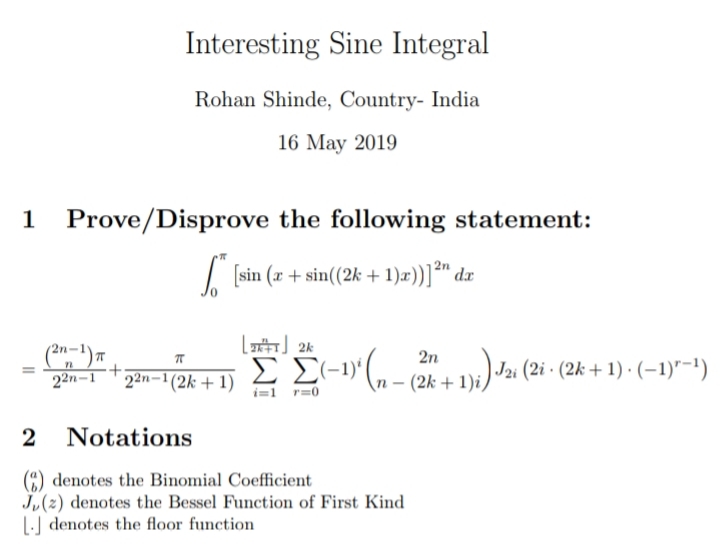 Here is the generalized version you needed. There might be a mistake,please verify it once.
Here is the generalized version you needed. There might be a mistake,please verify it once.
Wait, is that a paper you published????!!
Not a paper actually , it's just a problem I proposed few days back with the solution for a magazine.
If we define J ( m , D ) = ∫ 0 2 π cos 2 m ( x + sin ( D x ) ) d x m , D ∈ N then J ( m , D ) = R e [ ∫ 0 2 π e 2 m i ( x + sin ( D x ) ) d x ] = R e [ ∫ ∣ z ∣ = 1 z 2 m e m ( z D − z − D ) i z d z ] = 2 π R e [ R e s z = 0 z 2 m − 1 e m ( z D − z − D ) ] if m = 0 , then the Laurent series of e m ( z D − z − D ) will only contain terms of the form z D k , where k ∈ Z . Thus z 2 m − 1 e m ( z D − z − D ) will only have a term in z − 1 if 2 m is a multiple of D . Thus we can certainly ensure that J ( m , D ) = { 2 π 0 m = 0 1 ≤ m ≤ n provided that D is odd and n < D . Since sin 2 n θ ≡ 2 2 n − 1 1 j = 1 ∑ n ( − 1 ) j ( n + j 2 n ) cos 2 j θ + 2 2 n 1 ( n 2 n ) we deduce that ∫ 0 π sin 2 n ( x + sin ( D x ) ) d x = 2 1 ∫ 0 2 π sin 2 n ( x + sin ( D x ) ) d x = 2 2 n 1 j = 1 ∑ n ( − 1 ) j ( n + j 2 n ) J ( j , D ) + 2 2 n + 1 1 ( n 2 n ) J ( 0 , D ) = 2 2 n π ( n 2 n ) provided that D is odd and n < D . In the case n = 1 0 , D = 1 5 we get an integral of 2 6 2 1 4 4 4 6 1 8 9 π making the answer 3 0 8 3 3 3 .
The answer is not as straightforward when n ≥ D , since there would be other residues to consider. For example ∫ 0 π sin 2 D ( x + sin ( D x ) ) d x = 2 2 D π ( D 2 D ) − 2 2 D − 1 π J 2 ( 2 D ) for odd positive integers D . In this case J ( D , D ) is nonzero. We are using J 2 , a Bessel function of the first kind, here.