Is the information sufficient?
In the diagram, A C = 1 , where C is the midpoint of side A B of triangle A B J . Square B C D E is drawn, where D lies on side A J and E is in the interior of the triangle. Square F G I H is drawn, where G lies on side B J , H lies on side A J , I is in the interior of the square, and E is the midpoint of F G .
Find the area of triangle A B J .
The answer is 3.00.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
11 solutions
Moderator note:
Great usage of similar triangles to gain more information from the diagram.
Exactly the "nice", non-trigonometrical solution I was looking for!
Ooohh, filling in that "missing triangle" allows us to work directly with the numerous similar triangles :)
Very nice!
Why does fe=df/2?
Log in to reply
If you look at the equation, it says FE = FH / 2 = DF / 2.
The first equation follows because E is the midpoint of the square. (It might be clearer to say FE = FG / 2 = FH / 2 )
The second equation follows from line 1 of DF = FH.
Why is BEG similar to BJK?
Log in to reply
They are both made of a vertical side, a horizontal side, and a side at slant. They share the slanted side, BJ shortened to BG, so this side, being the same line, must make the same angles with the horizontal and vertical lines. Specifically EG corresponds to BK, EB to KJ, and BG to JB.
I love the way you used the similar triangles at the end. I created an infinite series and solved that, then smacked my head against the desk when I saw your solution :)

Hence, the height of the triangle is given by: 1 + 3 2 + 9 4 + . . . = 3
And therefore, the area of the triangle is: 2 3 × 2 = 3 .
Solution behind the height of the triangle.
Since the triangle ACD is a isosceles right triangle and DF // AC, hence, ∠ DAC = ∠ HDF = 4 5 ∘ .
Let FE = x . ⟹ F G = F H = F D = 2 x .
By Whole-Part Theorem, FD + FE = BC = 1 ⟹ x = 3 1 and hence, the side of square FGIH = 2x = 3 2 .
The side of the smaller square ( i.e. 9 4 is also obtained by doing these method. )
Now, as you observed, the sides of the square forms a infinite geometric progression, which when you add it all, it is equal to the height of the triangle.
The rest of the solution is given above. 😀😀
Nice setup.
Ideally, the solution should allow those who couldn't solve it to understand what you did. Can you provide a few more details like why D F = 3 2 .
Log in to reply
Sir, what do you think of the updated solution? 😄😄.
I like this problem.
good problem as well as the solution ,i used the same approach .
and make sure you replace the square F G H I by F G I H . because F G H I is an hyperboloid not a square
Did the same way
Do you mean point I is in the interior of the triangle, or on the perimeter of the square, because point I certainly isn't inside square HIGF.
we can see line F E satisfies two eqns: { D F + F E = 1 2 F E = D F the first eqn is true since the two segments are just part of another segment with length 1, the second is true since it is similar to triangle δ A D C , meaning it is isosceles. we can solve to get F E = 3 1 . we consider two similar triangles: A B J , D G J . we can see that [ A B J ] [ D G J ] = ( A B D G ) 2 = ( 2 1 + 3 1 ) 2 = 9 4 the area of the trapazoid [ A B G D ] = 2 D C ( D G + A B ) = 3 5 we can see [ D G J ] + [ A B G D ] = [ A B J ] let v = [ A B J ] . then 9 4 v + 3 5 = v → v = 3
I think there's a typo when comparing the area of two similar triangles, it should be 9 4 instead of 3 4 , but the latter part is written correctly :)
sideways upvote
Similar triangles and squares: △ A B J ∼ △ D G J ; □ B C D E ∼ □ G F H I ; △ A C D ∼ △ D F H .
From A C = C B it follows that D F = F G . On the other hand, D F + 2 1 F G = 1 . From this we conclude D F = 3 2 , which is also the ratio of similarity, x = 3 2 .
We could continue to build smaller squares on top of the existing square, in the same manner. The top of this infinite tower converges to point J . Thus the height of J above base A B is y = 1 + x + x 2 + ⋯ = 1 − x 1 = 1 − 3 2 1 = 3 . The area of the triangle is therefore 2 1 ⋅ A B ⋅ y = 3 .
Nicely presented! It helps that the ratios of similarities of the triangles and the squares is the same.
I liked how you found the height of J above base A B by placing similar squares with sides in a geometric progression. Another way to find the total area of the triangle, but along similar lines, is to find areas of similar trapeziums.
We start with A B G D , which has area 2 2 + 3 4 × 1 = 3 5 . The next trapezium D G I H would have 9 4 the area of A B G D . Continuing this, we get total area of 3 5 ( 1 + 9 4 + 9 2 4 2 + ⋯ ) = 3 5 × 5 9 = 3 .
Note that the homothety mapping F G I H to C B E D has center at the intersection of D H and B G , which is just J . Since E is mapped to the midpoint M of C B with respect to this homothety, then J , E , M are collinear. Let h be the length of the altitude from J to line D E , and let h ′ be the length of the altitude from J to A B . Note that h ′ − h = B E = 1 . By similar triangles △ J D E ∼ △ J A M , it follows that h ′ h = A M D E = 1 + 2 1 1 = 3 2 ⟹ h ′ = 2 3 h = 2 3 ( h ′ − 1 ) = 2 3 h ′ − 2 3 ⟹ 2 1 h ′ = 2 3 ⟹ h ′ = 3 Thus, the area of △ A B J is 2 1 ⋅ A B ⋅ h ′ = 2 1 ⋅ 2 ⋅ 3 = 3 done.
Note that this solution is particularly nice in that it works with the exact same calculations no matter what length A C was; in particular, it does not rely on the fact that ∠ C A D = 4 5 ∘ in any way. □
Oh wow! I wasn't expecting a homothety solution to a problem like this. Very unusual yet elegant!
Is homothety your goto move when you see this problem? I would never have thought about this...
Log in to reply
I've been practicing Olympiad Geometry for the past year or two, so I think I have developed an eye for synthetic methods :)
Essentially, the answer is the height of the triangle. If point A is the origin then we can create two lines y=x and y=3x-6. They intersect at a point with a y value of three, so that is the answer
Good use of coordinate geometry. Could you explain how you found the equations of the lines, especially the y = 3 x − 6 line? That seems to be the key point of this problem.
Triangles ACD and BCD are two equal right isosceles then triangle ABD is also right isosceles. Then ∠ C A D = 4 5 ∘ which means ∠ G D H is also 4 5 ∘ (alternate angles) and ∠ H G D = 4 5 ∘ then triangle DGH is right isosceles at H (since it has two 4 5 ∘ angles) but [HF] is a height of it then it is a median which means D F = F G = 2 F E therefore D F + F E = 1 then 3 F E = 1 therefore F E = 3 1 then D G = 4 F E = 3 4 . Now, ( A B ) ∣ ∣ ( D G ) then A B D G = 2 3 4 = 3 2 then J A J D = 3 2 then J A = 3 D A , but A D = 2 (Pythagorean Th.) then A J = 3 2 Therefore Area of Triangle A B J = 2 1 B D × A J = 2 2 × 3 2 = 3 .
Nice observation that B D is perpendicular to A J . It lets us use A J as the base of the triangle for calculating the area.
I didn't understand how you found out ∠ H G D = 4 5 ∘ before knowing that D F = F G . Could you please explain that part?
After we find that EG = 1/3 we know that EB = 1 then we can find the angle <GBE by doing Tan<GBE = 1 1 / 3
<GBE = 18.43
therefore <JBA = 90 + 18.43
<JBA = 108.43
we know that <A = 45
we can find <J which is 180 - 45 - 108.43
<J = 26.56
now we can find the length of AJ by doing
s i n 1 0 8 . 4 3 A J = s i n 2 6 . 5 6 2
Which gets us to AJ = 4.2435
and so after we find AJ we can calculate the area by the formula 2 ( A J ∗ A B ∗ s i n < A ) 2 4 . 2 4 3 5 ∗ 2 ∗ s i n 4 5 which gets us to 3.000.
Because this solution is based heavily on numerical approximations. Let me ask you this: You have only ensured that the first three digits after the decimal is 0, how does that tell you that the answer is indeed 3 and not something like 3.0001?
Suppose we construct line segments between D and B and between H and G. Since AC = CD, angle CAD is 45 degrees. So is angle CBD, since segment DB is the diagonal of a square, and bisects a 90 degree angle. Similarly, angles FDH and FGH are also 45 degrees.This means that the square constructed in triangle DGJ is the same proportion to the square inside triangle ABJ as the triangle DGJ is to triangle ABJ. This construction can therefore be continued ad infinitum. As the upper right corner of the square is always interior to the triangle, it asymptotically approaches vertex J. If we now draw a line through all these corners through the baseline, it is clear that it must bisect segment BC at point K, since E bisects FG, and the fact that all the squares are constructed similarly. The tangent of angle IEG is 2, so it must also be the tangent of angle JKB. Finally, we drop a perpendicular to an extension of the baseline, intersecting at point L. Since AL = JL, and KL = 1/2 JL, KL = 1/2 AL. AL = AC + 1/2 BC = 1.5, so AL = JL = 3. The area of triangle ABJ is 2 * 3 / 2 = 3.
Since AC = CD, angle CAD is 45 degrees.
AC = CD is not given. How did you deduce it.
I think you are going at a different approah that others which is really nice. It'd help others to undersand if you put up a diagram.
Log in to reply
A C = C D because C B E D is a square, and we know A C = C B .
Very simple:
AB = 2, DG = 4/3, vertical distance between AB and DG = 1. Assume vertical distance between DG and Jamal = x.
DG/AB = x / (x + 1)
x/(x+1) = 4/3/2 = 2/3
3x = 2x + 2
Solving for x, x = 2
height = 3
Area = 1/2 × 2 × 3 = 3
It is not clear to me why is DG equal to 4/3. Could you please explain how you got this result?
Also, do you mean to say J instead of Jamal?
@Pranshu We can see that DFG maintains the same proportions as ABC. Since B is the midpoint of ABC, F must be the midpoint of DFG.
For easy visualization, we can add a point Q at the midpoint of DF, so that DG is now broken into 4 equal segments, and Since we know DE = 1, and is 3 segments long, each segment = 1/3. Thus, the 4 segments together must be 4/3.
Log in to reply
Oh yes, I get it now. Thanks for explaining :) I didn't realize that the two squares are similar in the same ratio as the triangles JDG and JAB
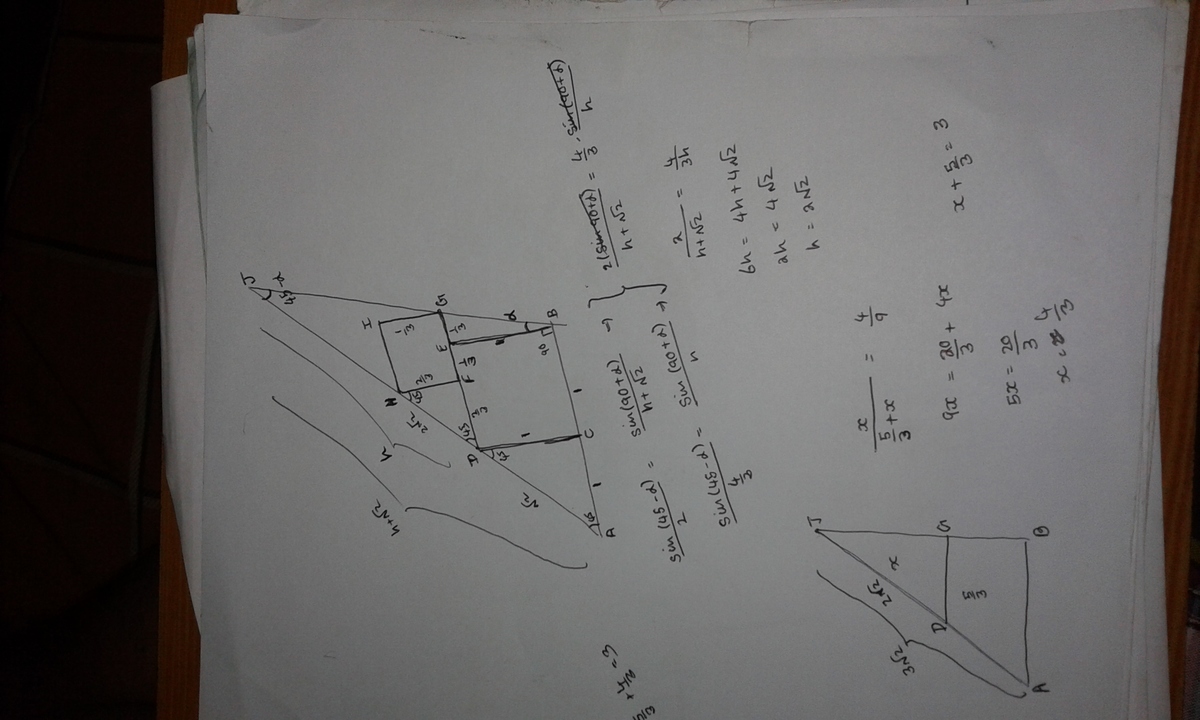
∠ C A D = 4 5 ∘ , therefore D F = F H .
F E = 2 F G = 2 F H = 2 D F , so the segment D E is divided in thirds with D F = 3 2 and F E = E G = 3 1 .
This will give us ratio of E G E B = 3 .
Triangle J B K is similar to triangle B G E so B K J K = 3 .
But J K = A K because of the 4 5 ∘ angle, so 3 a = 2 + a and a = 1 . The height of triangle A B J = 3 a = 3 and the area is also 3 .