What Is The Sandwiched Angle?

Area of triangle = $\frac{1}{2}$ * sinθ * ab, here, a = 2, b = 2, max sinθ = sin90 = 1, Max area = 2

Let $\triangle ABC$ be an isosceles triangle with $AB = AC = 2$ and $0 \lt \angle BAC \lt \pi$ then $0 \lt \sin \angle BAC \leq 1$

Using the sine rule to calculate the area of the triangle.

area $(\triangle ABC) = \frac{1}{2} \cdot AB \cdot AC \cdot \sin \angle BAC \leq \frac{1}{2} \cdot 2 \cdot 2 \cdot 1 = 2$

So

$\color{#3D99F6} {area (\triangle ABC) \leq 2}$

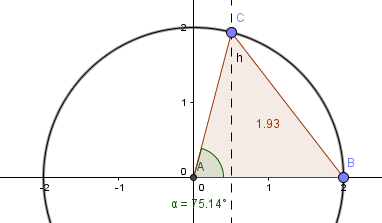
If we put the one of two sides with lengh = 2 as the base of the triangle, the other vertex is in a cercle of radius=2

and AREA = 2 * h / 2 (the maximum value of h is 2, the maximum area is 2 when the triangle is rectangle)

The area of triangle with sides $a$ and $b$ , subtending an angle of $\theta$ is $\dfrac{1}{2} ab \sin \theta$ .

So the area of this triangle is $\dfrac{1}{2}(2)(2)(\sin \theta)=2\sin \theta$ .

Since $\sin \theta$ has a maximum of $1$ , the maximum area is $2$ .

Using Heron's formula for area of a triangle A=√s(s-a)(s-b) (s-c), where a,b and c are three sides and s=(a+b+c)/2 i. e. half the perimeter, we find in the instant case that a=b=2 and let the third side be 2x. Then s=(2+2+2x)/2,
s=(x+2). Therefore, area of triangle.
A=√(x+2)(x+2-2)(x+2-2)(x+2-2x) =√x^2(4-x^2) =√{4-(4-4x^2+x^4)=√{4-(2-x^2)^2} Therefore, A will be maximum when x^2-2=0 i. e. the third side is 2x=2√2, hypotenuse of the right triangle, and the maximum value of area will be A=√4=2.

note that area of this isosceles triangle is 2sin2x where x is the base angle and since max value sin2x can achieve is 1, the answer is 2