It is simple if you think about it
If a line is drawn through a fixed point P ( α , β ) to the circle x 2 + y 2 = a 2 at A and B , then express P A × P B in terms of α , β and/or α .
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
If one was pressed for time, maybe one could assume ( α , β ) to be a point on the circle. Then, α 2 + β 2 = a 2 ⋅
Moreover, P A × P B = 0 and the result follows from the options.
Nice Solution by the way ! Cheers
Draw a diameter A 0 B 0 passing through P.
P A ∗ P B = P A 0 ∗ P B 0
from the diagram we can see that P A 0 = r − a & P B 0 = r + a where r = α 2 + β 2
So our equation now becomes
P
A
∗
P
B
=
(
r
−
a
)
(
r
+
a
)
=
r
2
−
a
2
=
α
2
+
β
2
−
a
2
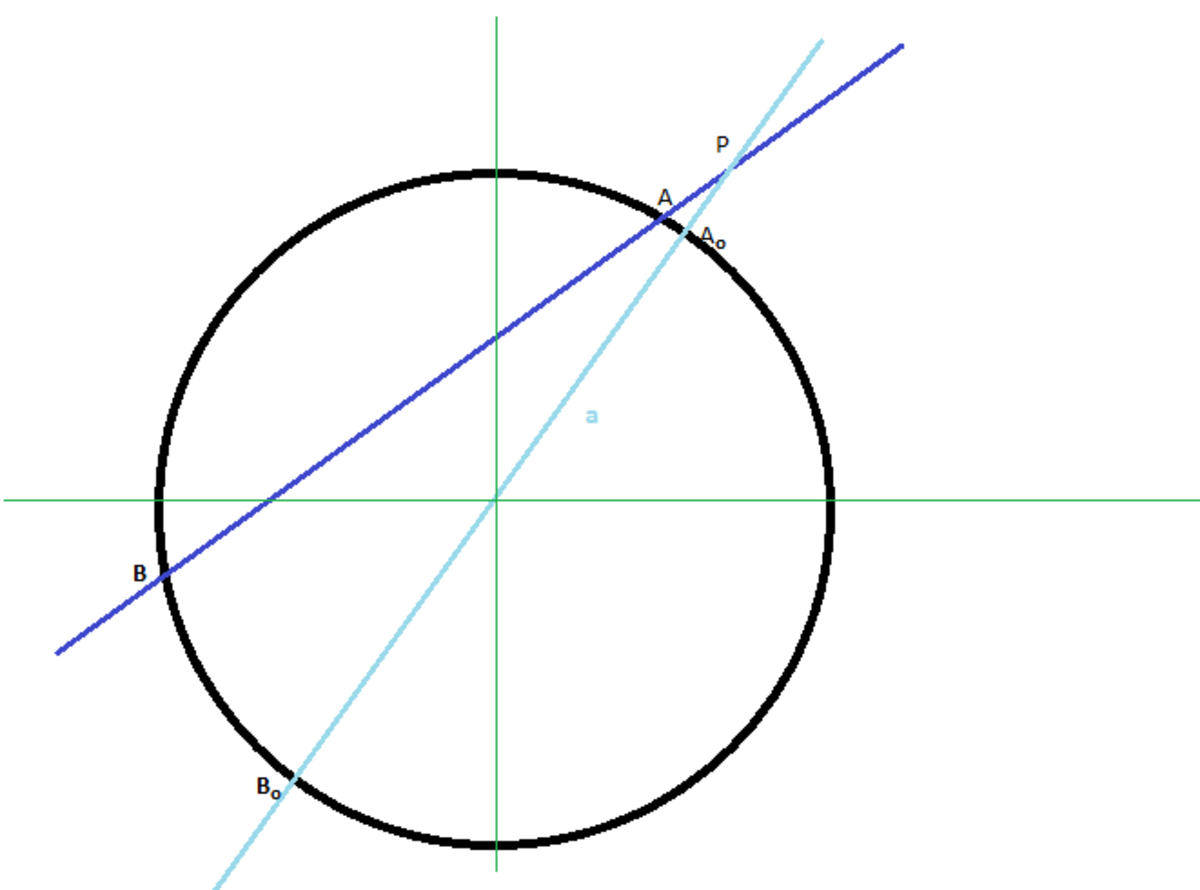
From the image above and using Tangent-Secant theorem, it is known that
P A × P B = P T 2
Using notation as found in S.L.Loney's Coordinate geometry (to avoid ambiguity):
The length of the tangent, i.e., P T = S 1
S 1 = α 2 + β 2 − a 2
P A × P B = P T 2 = S 1 = α 2 + β 2 − a 2
Note