It Seemed Like A Good Idea At The Time

Classical Mechanics Level 2

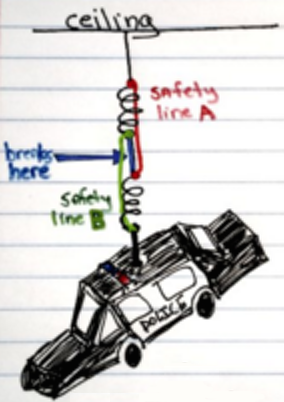
The sketch above shows a police car suspended from the ceiling. The suspension system is two identical springs connected by a short length of rope.

After the springs have reached equilibrium, two safety lines are attached with no slack in them: one connecting the tops of the two springs and one connecting the bottoms of the two springs.

But the springs aren't the weakest link. It's the short length of rope between the two springs that snaps!

What happens when the rope between the two springs breaks?

My alma mater has a bit of an obsession with suspending cars from the ceiling.
The car drops a bit down towads the people The car crashes down to the ground The car moves up towards the ceiling The car stays at the same height

Zandra Vinegar by Zandra Vinegar

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

3 solutions

Jihan Ryeosomnia
Feb 8, 2016

The car moves up towards the ceiling and the springs aren't the weakest link

3 Helpful 0 Interesting 0 Brilliant 0 Confused
Pranav Rao
Feb 8, 2016

Correct me if I'm wrong, but I think this has to do with springs in series and parallel. In the first case the springs are in series and the equivalent spring constant is (K1)(K2)/[K1+K2]. In the second case when the middle string snaps, the springs are connected in parallel. This is because the extensions in each of the springs is same and the force due to each spring add to give net force pulling the block upwards. So the equivalent spring constant is K1+K2 which is more than case 1. So the net extension in second case is less and the car moves up to remain in equilibrium.

1 Helpful 0 Interesting 0 Brilliant 0 Confused
Beng Ah
Feb 9, 2016

Assume there is no tension on safety lines before the rope snaps. The 2 springs are connected in series, and hence k' = 1/(1/k + 1/k) = k/2. After rope snaps, tension is on the safety lines and the 2 springs form a parallel connection, hence k'' = k+k = 2k. Since F=k'/x, x= F/k', before snapping, x= F/k/2 = 2F/k, after snapping, x= F/2k, so after snapping, the car will move upwards

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...