It's chess time!
Logic
Level
4
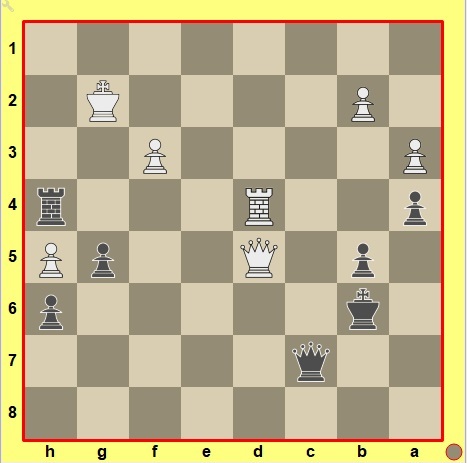It's black turn to move. What is the minimum number of moves for black to checkmate the white king? Assume that both white and black play optimally.
6
5
4
3
10
7
8
9
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
My solution goes like this: 1. Rh2+ Kf1 2. Rh1+ Ke2 3. Qh2+ Kd3 4. Rd1+ Ke2 5. Re1+ Kd3 6. Qe2+ Kc3 7. Rc1+ Kb4 8. Qxb2+ Qb3 9. Qxb3#
Other choices are possible for black, but end up with earlier mates as far as I could tell. That was a long sequence to visualize, especially with the many side choices!