It's Golden!
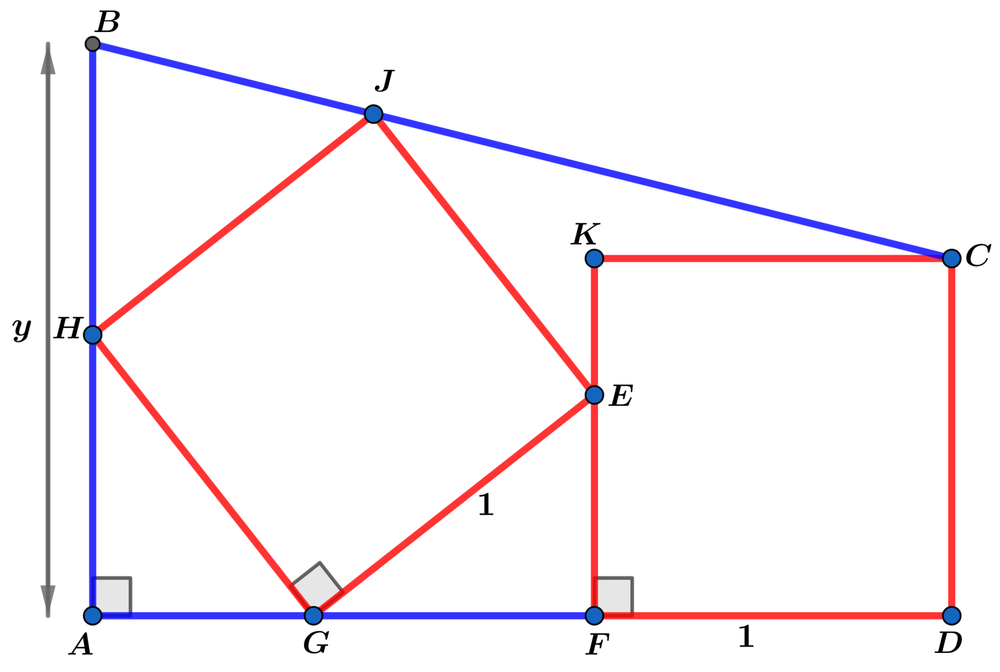
In quadrilateral A B C D , F K C D and G H J E are squares with side length 1 as shown above.
If max ( y ) = α + ϕ β ( ϕ − α ) β λ , where α , β and λ are coprime positive integers and ϕ is the golden ratio, find α + β + λ .
The answer is 6.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions

Using the above diagram I K = ( m + n ) sin ( θ ) = sin ( θ ) and J I = sin ( θ ) + cos ( θ ) − 1
and △ J I C ∼ △ B P C ⟹ y − 1 sin ( θ ) + cos ( θ ) + 1 = sin ( θ ) + cos ( θ ) − 1 sin ( θ ) + 1
⟹ y = 1 + sin ( θ ) + 1 sin ( 2 θ ) ⟹
d x d y = ( sin ( θ ) + 1 ) 2 ( 2 cos ( 2 θ ) ) ( sin ( θ ) + 1 ) − sin ( 2 θ ) cos ( θ ) = 0 ⟹
( 2 cos 2 ( θ ) − 1 ) ( sin ( θ ) + 1 ) = sin ( θ ) cos 2 ( θ ) ⟹ sin 3 ( θ ) + 2 sin 2 ( θ ) − 1 = 0
Let u = sin ( θ ) ⟹ u 3 + 2 u 2 − 1 = 0 ⟹ ( u + 1 ) ( u 2 + u − 1 ) = 0 and
u = − 1 ⟹ u = 2 5 − 1 = ϕ − 1 = sin ( θ ) dropping the negative root
⟹ cos ( θ ) = ϕ − 1 ⟹ y ( m a x ) = 1 + sin ( θ ) + 1 2 sin ( θ ) cos ( θ ) =
1 + ϕ 2 ( ϕ − 1 ) 2 3 = α + ϕ β ( ϕ − α ) β λ ⟹ α + β + λ = 6 .
You can check that a max does occur at θ = arcsin ( ϕ − 1 ) .
I checked and d θ 2 d 2 y ∣ θ = arcsin ( ϕ − 1 ) < 0 .
Let A be the origin ( 0 , 0 ) of the x y -plane with A D and A B be along the x -axis and y -axis respectively., and ∠ A G H = θ . Then J = ( sin θ , sin θ + cos θ ) and C = ( sin θ + cos θ + 1 , 1 ) . The equation of the line B C is given by:
x − sin θ − cos θ − 1 y − 1 ⟹ y d t d y t 4 + 4 t 2 ( t 2 + 2 ) 2 ⟹ t ⟹ max ( y ) = sin θ − sin θ − cos θ − 1 sin θ + cos θ − 1 = 1 + cos θ + 1 2 sin θ cos θ = 1 + 1 + t 2 1 − t 2 + 1 2 ⋅ 1 + t 2 2 t ⋅ 1 + t 2 1 − t 2 = 1 + 1 + t 2 2 t ( 1 − t 2 ) = ( 1 + t 2 ) 2 2 ( t 4 + 4 t 2 − 1 ) = 1 = 5 = 5 − 2 = 2 φ − 3 = φ φ 1 = φ φ − 1 = 1 + 1 + t 2 2 t ( 1 − t 2 ) = 1 + φ 2 φ − 1 ⋅ φ 2 + φ − 1 φ 2 − φ + 1 = 1 + φ 2 φ − 1 ⋅ 2 φ 2 = 1 + φ 2 ( φ − 1 ) 2 3 Putting x = 0 Let t = tan 2 θ To find max ( y ) Putting d t d y = 0 Since θ < 9 0 ∘ Golden ratio φ = 2 1 + 5 ⟹ 5 = 2 φ − 1
The required answer α + β + γ = 1 + 2 + 3 = 6 .