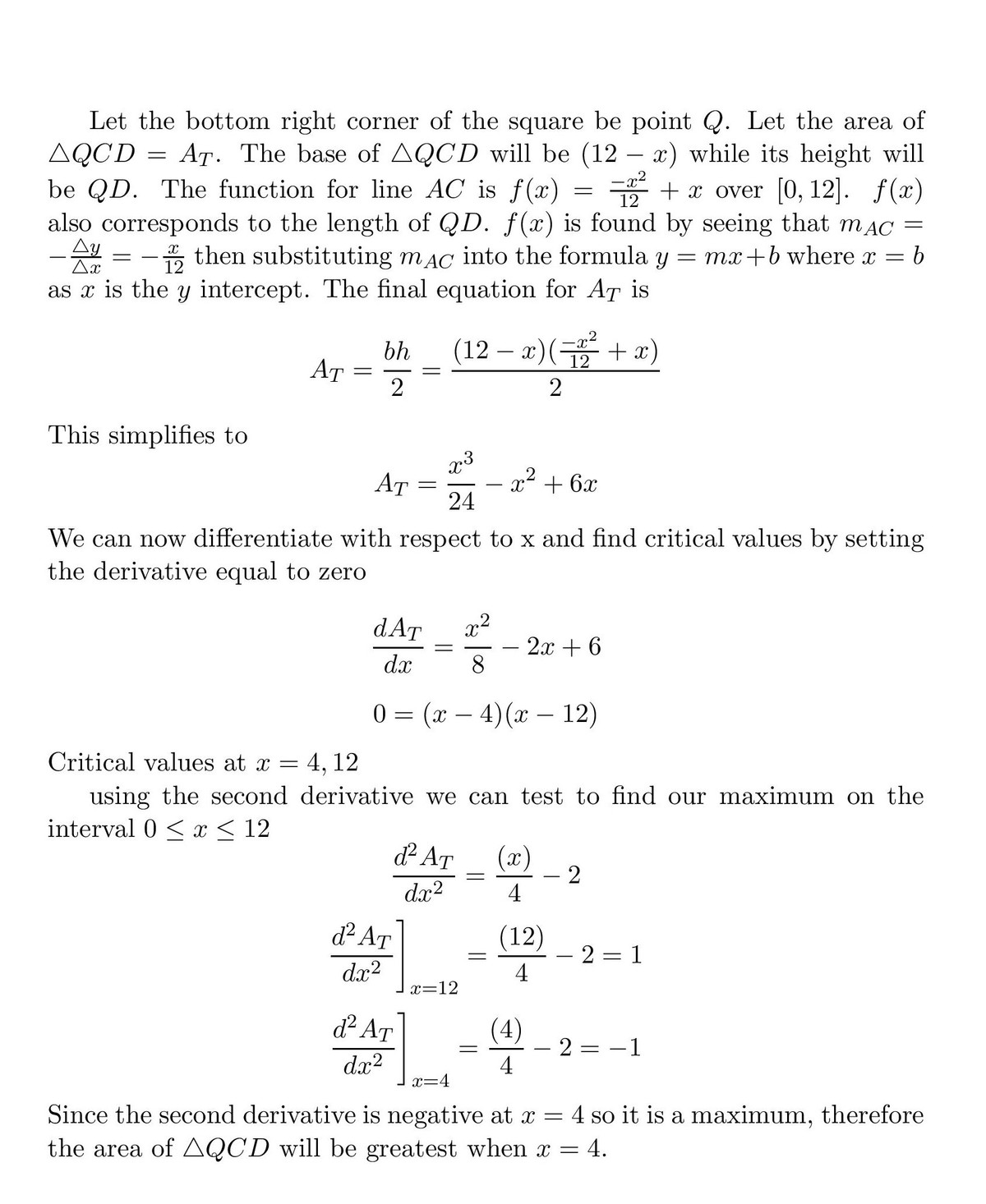
Its maximum area
A right triangle
with side
and
intersects a square with side
at the point
. Assuming
is variable, find the value of
that maximizes the shaded portion of the triangle.
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the lower right corner of the square be P . Then Δ A B C and Δ D P C are similar, and so
∣ B C ∣ ∣ A B ∣ = ∣ P C ∣ ∣ D P ∣ ⟹ 1 2 x = 1 2 − x ∣ D P ∣ ⟹ ∣ D P ∣ = 1 2 x ( 1 2 − x ) .
Now the area A of the shaded region is
A = 2 1 ∗ ∣ P C ∣ ∗ ∣ D P ∣ = 2 4 1 x ( 1 2 − x ) 2 .
Differentiating A with respect to x and setting d x d A = 0 gives us that
d x d A = 2 4 1 ( ( 1 2 − x ) 2 − 2 x ( 1 2 − x ) ) =
2 4 ( 1 2 − x ) ( ( 1 2 − x ) − 2 x ) = 2 4 ( 1 2 − x ) ( 1 2 − 3 x ) = 0
when either x = 1 2 or x = 4 . Now if x = 1 2 then there would be no shaded region at all, so the value we are interested in is x = 4 . Now
d x 2 d 2 A = 4 x − 8 < 0 for x = 4 , and thus by the second derivative test we conclude that A is maximized for this value of x , i.e., the desired value of x is 4 .